Antiderivative of a Fraction: Complete Explanation and Examples

The antiderivative, also called the integral of a function, is the inverse process of taking the derivative of a function.

When we have a function $\dfrac{p}{q}$ where $q \neq 0$, then such an expression is called a fraction, and if we take the antiderivative of such a function, then it will be called the antiderivative of that fraction.

In this topic, we will discuss how to take the antiderivative or integral of a fraction, and we will discuss in detail solving fraction problems using the partial fraction technique of integration.

What Is the Antiderivative of a Fraction?

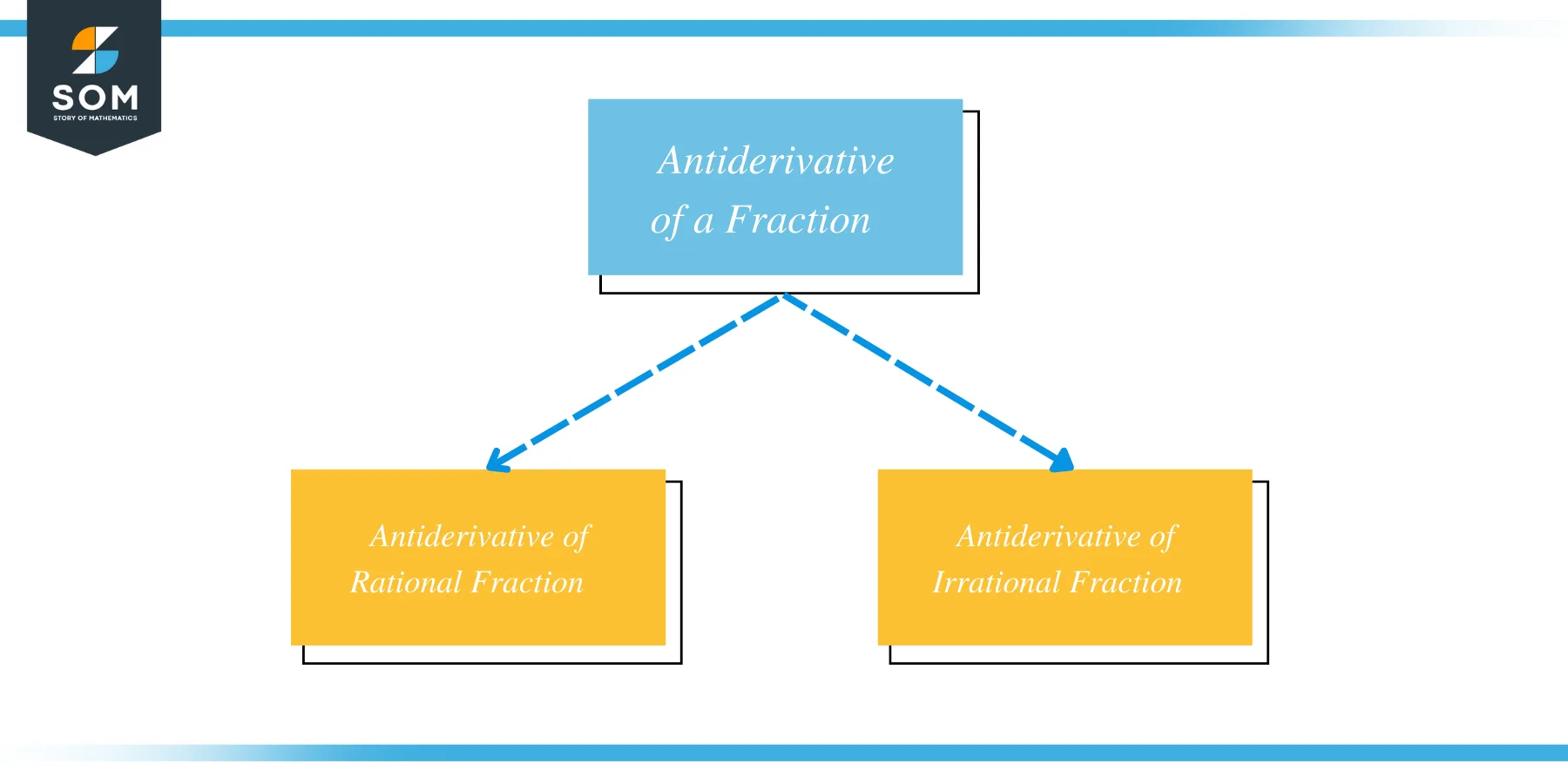
The antiderivative, also called the integral of a function, is the inverse process of taking the derivative of a function; if we take the antiderivative of an algebraic function that is written as a fraction, we call it the antidifferentiation of a fraction. We know that a fraction is given in $\dfrac{p}{q}$ with $q \neq 0$. The antiderivative of a fraction can be divided into two types.

To solve antiderivative problems, some basic antiderivative relations must be memorized. For example, the antiderivative of a constant fraction is $\int \dfrac{1}{k} = \dfrac{1}{k} x +c$; the antiderivative of $\frac{1}{x}$ is $ln|x| +c$. Similarly, the antiderivative of $\dfrac{1}{x^{2}} $ is $-\dfrac{1}{x} + c$. 

How To Find the Antiderivative of Fractions

The simple answer to finding the antiderivative of an algebraic expression having multiple or complicated fractions is by using the fraction decomposition or separation of the fraction into smaller parts and then taking the antiderivative of those smaller fractions. Most rational fractions are solved by using partial fractions, while irrational fractions are solved by using the substitution method.

We will now discuss different examples related to fractions and how we can take the antiderivative of fractions with different types of quotients algebraic expressions.

Antiderivative of a Rational Fraction

A rational fraction is a fraction wherein both the numerator and denominator consist of polynomials. For example, $\dfrac{x + 7}{x}$ is a rational fraction.

We can easily calculate the antiderivative for the above given rational fraction by dividing it into parts. We can write $\dfrac{x + 7}{x}$ as $( \dfrac{x}{x} + \dfrac{7}{x})$. Let us now calculate the antiderivative of the given rational function.

$\int \dfrac{x + 7}{x} = \int(\dfrac{x}{x} + \dfrac{7}{x})$

$\int \dfrac{x + 7}{x} = \int ( 1 + \dfrac{7}{x})$

$\int \dfrac{x + 7}{x} = \int 1 + \int \dfrac{7}{x}$

$\int \dfrac{x + 7}{x} = x –  \dfrac{7}{x^{2}}$

It is not necessary that all the rational numbers can easily be divided into parts to find their antiderivative. The denominator can consist of multiple linear factors or repeated linear factors; in such cases, it is advisable to solve the problem using the partial fraction technique.

Fractions with Two Linear Factors

When we are given a fraction function such that the power/degree of the numerator is less than that of the denominator while the denominator has two distinct linear factors, then we can use a partial fraction to separate the fraction into smaller parts and then find out the antiderivative of the function.

For example, we are given an integral function $\int \dfrac{x}{(x + 3) (4 – x)}$, we will use partial fraction decomposition to separate the given fraction.

$\dfrac{x}{(x + 3) (4 – x)} = \dfrac{A}{(x + 3)} + \dfrac{B} {(4 – x)}$

$\dfrac{x}{(x + 3) (4 – x)} = \dfrac{A}{(x + 3)} + \dfrac{B} {(4 – x)}$

$\dfrac{x}{(x + 3) (4 – x)} = \dfrac{A (4 – x) + B (x-3)}{(x + 3) (4 – x)}$

$x = A (4 – x) + B (x – 3)$

Now we will choose the value of “x” in such a manner that it makes an algebraic expression with “A” or “B” zero. So let us take $x = 3$ and put it in the above equation:

At $x = 3$

$3 = A ( 4 – 3) + B ( 3 – 3)$

$A = 3$

At $x = 4$

$4 = A (4 – 4) + B ( 4 – 3)$

$B = 4$

$\dfrac{x}{(x + 3) (4 – x)} = \dfrac{3}{(x + 3)} + \dfrac{4} {(4 – x)}$

$\int \dfrac{x}{(x + 3) (4 – x)} = \int (\dfrac{3}{x + 3} + \dfrac{4} {4 – x})$

$\int \dfrac{x}{(x + 3) (4 – x)} = \int \dfrac{3}{x + 3} + \int \dfrac{4} {4 – x})$

$\int \dfrac{x}{(x + 3) (4 – x)} = 3 \int \dfrac{1}{x + 3} – 4 \int \dfrac{-1} {4 – x})$

$\int \dfrac{x}{(x + 3) (4 – x)} = 3 ln (x +3) – 4 ln (4 – x) + c$

The examples we have studied so far used definite integrals but with no upper and lower limits. Let us now solve an example with upper and lower limits using the partial fraction decomposition method.

Example 1: Evaluate the given antiderivative function.

$\int_{2}^{4} \dfrac{4}{x (x + 2)}$

Solution:

$\int_{2}^{4} \dfrac{4}{x (x + 2)}$

By using the partial fraction decomposition method, we can write the above equation as:

$\dfrac{4}{x (x + 2)} = \dfrac{A}{x} + \dfrac{B} {(x + 2)}$

$\dfrac{4}{ x (x + 2)} = \dfrac{A}{x} + \dfrac{B} {(x + 2)}$

$\dfrac{4}{x (x + 2)} = \dfrac{A (x + 2) + Bx }{x (x + 2)}$

$4 = A (x + 2) + Bx$

Now we will choose the value of “x” in such a manner that it makes an algebraic expression with “A” or “B” zero. So let us take x = 0 and put it in the above equation:

At $x = 0$

$3 = A ( 0 + 2) + B (0)$

$3 = 2A$

$A = \dfrac{3}{2}$

At $x = -2$

$4 = A (2 – 2) – 2B$

$4 = -2B$

$B = -2$

$\dfrac{x}{(x + 3) (4 – x)} = \dfrac{3}{(x + 3)} + \dfrac{4} {(4 – x)}$

$\int_{2}^{4} \dfrac{x}{(x + 3) (4 – x)} = \int_{2}^{4} (\dfrac{3}{x + 3} + \dfrac{4} {4 – x})$

$\int_{2}^{4} \dfrac{x}{(x + 3) (4 – x)} = \int_{2}^{4} \dfrac{3}{x + 3} + \int_{2}^{4} \dfrac{4} {4 – x})$

$\int_{2}^{4} \dfrac{x}{(x + 3) (4 – x)} = 3 \int_{2}^{4} \dfrac{1}{x + 3} – 4 \int_{2}^{4} \dfrac{-1} {4 – x})$

$\int_{2}^{4} \dfrac{x}{(x + 3) (4 – x)} = [3 ln (x +3) – 4 ln (4 – x) ]_{2}^{4}$ 

$\int_{2}^{4} \dfrac{x}{(x + 3) (4 – x)} = [3 ln (4 +3) – 4 ln (4 – 4) – 3 ln (2 + 3) + 4 ln (4 – 2) ] $

$\int_{2}^{4} \dfrac{x}{(x + 3) (4 – x)} = ( 5.8377 – 4 – 4.828 + 2.772) = -0.22$

Fractions With Repeated Factors

When we are given a fraction function such that the power/degree of the numerator is less than that of the denominator while the denominator has repeated linear factors, we have to use a partial fraction to separate the fraction into smaller parts and then find out the antiderivative of the function.

For example, if we are given an integral function $\int \dfrac{x}{(x + 3) (4 – x)}$, we will use partial fraction to separate the given fraction.

$\dfrac{4}{(x – 4)^{2} (x + 4)} = \dfrac{A}{(x – 4)} + \dfrac{B} {(x – 4)^{2}} + \dfrac{C} {(x + 4)}$

$\dfrac{4}{(x – 4)^{2} (x + 4)} = \dfrac{A (x – 4) (x+4) + B (x + 4) + C (x-4)^{2}}{(x – 4)^{2} ( x +4)}$

$4 = A (x – 4) (x + 4) + B (x + 4) + C (x – 4)^{2}$

At $x = 4$

$4 = 0 + B ( 4 + 4) + 0 = B = \dfrac{1}{2}$

At $x = – 4$

$4 = 0 + 0 + C (-4 – 4)^{2}$

$4 = 64 C$

$C = \dfrac{1}{16}$

We know the value of B and C, now let us put x = 0:

At $x = 0$

$4 = -16 A + 4B + 16 C

$4 = -16A + 4 \times \dfrac{1}{2} + 16 \times \dfrac{1}{16}$

$4 = -16 A + 2 + 1$

$A = – \dfrac{1}{16}$

$\int \dfrac{4}{(x – 4)^{2} (x + 4)} = \int [\dfrac{A}{(x – 4)} + \dfrac{B} {(x – 4)^{2}} + \dfrac{C} {(x + 4)}]$

$\int \dfrac{4}{(x – 4)^{2} (x + 4)} = -\dfrac{1}{16} \int \dfrac{1}{(x – 4)} +\dfrac{1}{2} \int \dfrac{1} {(x – 4)^{2}} + \dfrac{1}{16} \int \dfrac{1} {(x + 4)}$

$\int \dfrac{4}{(x – 4)^{2} (x + 4)} = -\dfrac{1}{16} ln |x-4| + \dfrac{1}{ 2 (x-4)} +\dfrac{1}{16} ln |x + 4| + c$

Antiderivative of an Irrational Fraction

Antiderivative of an irrational function can be determined by using the substitution method only. Earlier, we discussed how to calculate the antiderivative of a rational function, and now we will discuss how to determine the antiderivative of an irrational fraction.

An irrational fraction includes non-polynomials in the numerator or the denominator. For example, $\dfrac{1}{\sqrt{x^{2} + 5x}}$ is an irrational number.

Example 2: Evaluate the given antiderivative function.

$\int \dfrac{5x}{\sqrt{x + 2}}  dx$

Solution:

Let $v = \sqrt{x + 2}$

So we know then that $v^{2} = x + 2$. Hence, $x = v^{2} – 2$.

Now taking derivative on both sides, we will get:

$dx = (2v – 0) dv = 2v dv$

Now putting the values of “x”, dx and v in the original equation:

$\int \dfrac{5x}{\sqrt{x + 2}}  dx = \int \dfrac{5 (v^{2}-2)}{v}. 2vdv$

$= 2 [\int 5v^{2}- 10 dv]$

$= 2 [ 5 \dfrac {v^{3}}{3} – 10 v ]$

$= 10 \dfrac {v^{3}}{3} – 20v + c$

So we can solve the antiderivative of rational and irrational fractions by using partial fraction and substitution methods, respectively.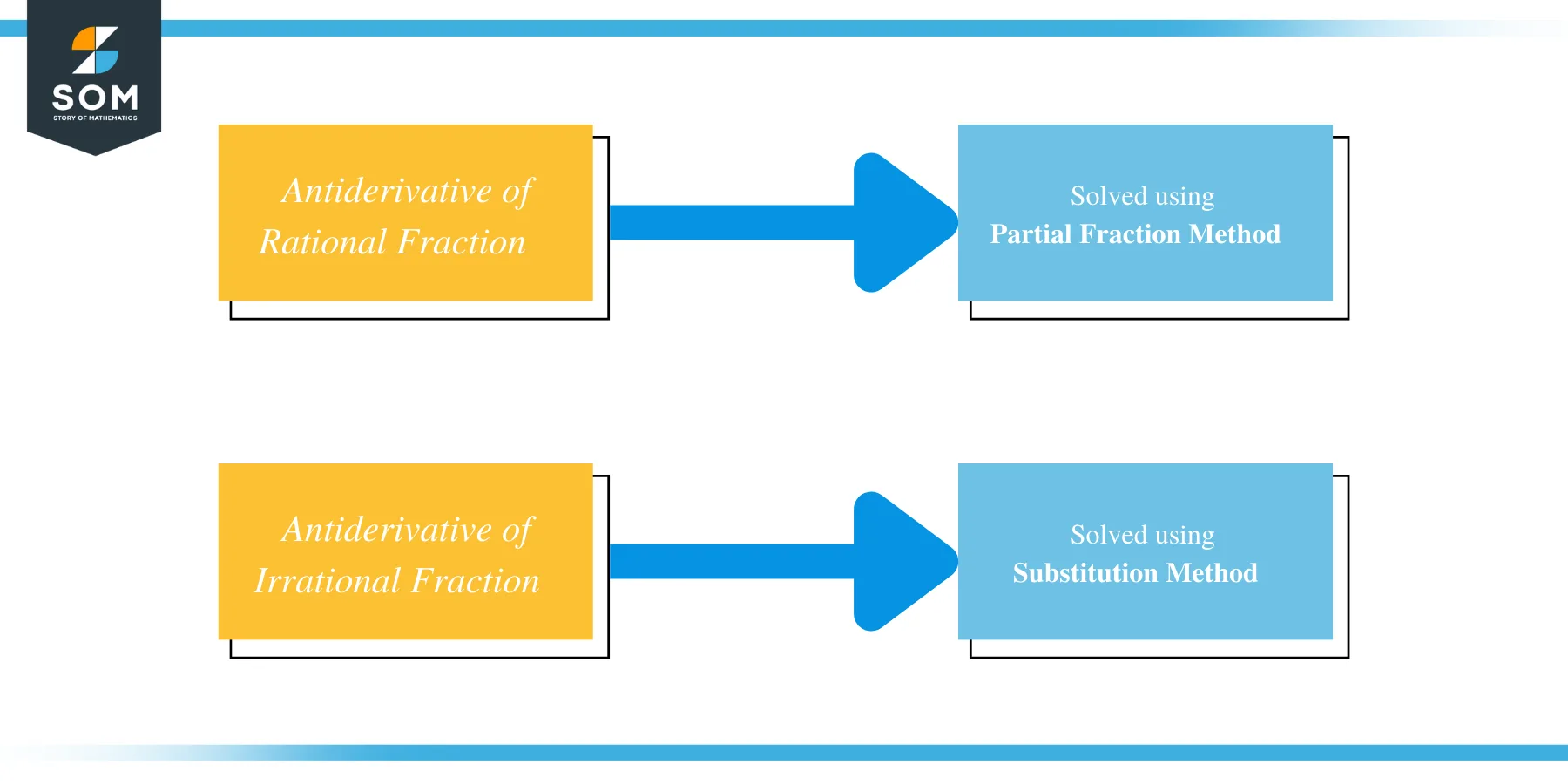

Practice Questions

1. Evaluate the antiderivative of function $y = \int \dfrac{3x^{2}}{x +1}$.
2. Evaluate the antiderivative of function $y = \int \dfrac{dx}{x \sqrt{x – 6}}$.

Answer Key

1)

The anti-derivative of the fraction is $\frac {3x^{2}}{2} -3x + 3 ln|x+1| + c$.

2)

The anti-derivative of the fraction is $tan^{-1} \dfrac{\sqrt{x-6}}{2} + c$.