JUMP TO TOPIC

Factors – All Factors of a Number

Factors are the numbers that are exactly divisible by the given original number, which means that they divide the original number leaving nothing as a remainder.

Figure 1 – Group of 6 students have been divided into 2 groups with 3 student each

The word “Factor” has been derived from the Latin word “faitor” which means creator or doer. The factor is a divisor of a given number. Factors are important because they allow you to break a number down into smaller parts. When two whole numbers are multiplied together, they are regarded as the factors of the resulting product.For instance, the product of two whole numbers 2 and 3 is given as:\[ 2 \times 3 = 6 \]such that 2 and 3 are the factors of 6.The Factoring technique has so many uses in real life! You can use it to find the factors of something, which will allow you to divide everything into equal parts or maybe in various real-life instances such as when exchanging money or understanding time while on vacation.Factoring comes up with all kinds of solutions for problems like these which must sound too complex right now but wait until we get more specific about how factored things are and how it is done.

What Are Factors?

Factors are the numbers that divide the other number such that the remaining number is zero.Factors can be determined using the division or multiplication method. In addition to these two methods, rules of divisibility can also be used to find the factors of any given number. Factors are not only in the form of numbers but they can also be the algebraic expressions dividing another expression equally.Factors can be positive as well as negative. The negative factors are the same as positive ones with the opposite sign.To better understand the concept of finding factors, let’s just find the factors of number 8.8 can be factored as following:\[ 1 \times 8 = 8 \]\[ 2 \times 4 = 8 \]Therefore, 8 can be factored in as 1, 2, 4, and 8 since we have already learned earlier that the negative factors are nothing but positive factors with opposite signs. So the negative factors are -1, -2, -4, and -8.The product of two negative numbers is always a positive number where 8 is the positive number, so the factors of 8 are given as:\[ 1, -1, 2, -2, 4, -4, 8, -8 \]Usually, the positive factors are considered while solving the problems related to factorization.

How To Find the Factors of a Number

To find the factors of any number, follow the steps mentioned below:

Step 1

Perform the prime factorization of the number whose factors are to be determined.

Step 2

Draw a factor tree for the number. All the numbers originating from the new branches are the factors of the given number.

Step 3

Now, multiply any two of the obtained prime factors to find the other factors of the number.

Step 4

Do not repeat the factors in the list.Let’s solve an example to understand the method better.For instance, find the factors of 136 using prime factorization.The prime factorization of 136 is given as: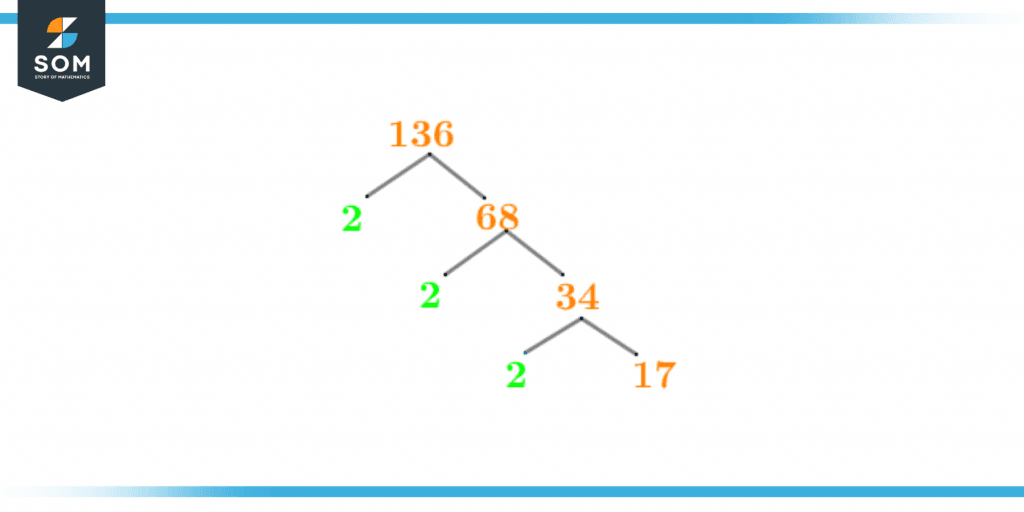

Figure 2 – Factor tree of 136 using prime factorization

Now, write the prime factors in the exponent form as:\[ Prime\ Factor\ of\ 136\ = 2 \times 2 \times 2 \times 17 \]So, the factors of 136 are:1, 2, 4, 8, 17, 34, 68, and 136.Here are some important properties of the factors of the number:

The factors of the number are either less than or equal to the given number in magnitude.
If a number is the factor of a given number then its additive inverse will also be the factor of the number.
The total number of factors is always finite.
The factors of a number can neither be in decimal form nor fractions.
The factors can be found by using the multiplication or division method.
Every number has at least two factors i.e. 1 and the number itself.
only 1 and 0 has 1 factor.

There are other methods such as the multiplication and division methods that can be used to determine the factors of the given number.Let’s explore each of the methods individually for a better understanding of the concept.

Multiplication Method

To find the factors of the number using the multiplication method, follow the steps given below:

Step 1

Express the given number in the form of the product of two whole numbers.

Step 2

Make all the possible pairs that give the number as the product.

Step 3

The whole numbers whose product is equal to the given number are the factors of that number.For instance, the factors of 36 using the multiplication method are given as:\[ 1 \times 36 = 36 \]\[ 2 \times 18 = 36 \]\[ 3 \times 12 = 36 \]\[ 4 \times 9 = 36 \]\[ 6 \times 6 = 36 \]Therefore, the factors of 36 found using the multiplication method are:\[ Factors\ of\ 36 = 1, 2, 3, 4, 6, 9, 12, 18, 36 \]

Division Method

To compute the factors of a number using the division method, follow the steps mentioned below:

Step 1

Make a list of numbers less than the given number whose factors are to be found.

Step 2

Divide each number by the given number.

Step 3

The numbers that when divided give zero as the remainder are the factors of the number.For example, the factors of 8 found using the division method are:\[ \dfrac{8}{1} = 8,\ r=0 \]\[ \dfrac{8}{2} = 4,\ r=0 \]\[ \dfrac{8}{3} = 2,\ r=2 \]\[ \dfrac{8}{4} = 2,\ r=0 \]\[ \dfrac{8}{5} = 1,\ r=3 \]Therefore, the factors of 8 are given as:\[ Factors\ of\ 8 = 1, 2, 4, 8\]The factors can be in the form of algebraic expressions as well. The algebraic factors can be found using various techniques such as factoring quadratic expressions or polynomials.Hence factors and multiples are very important mathematical concepts that are used for solving many problems

Factors of All the Numbers

Below is the list of factors of various numbers. Now, you do not have to worry about finding the factors of various numbers be it simple or complex, the solution is just one click away.

Factors Of 32
Factors Of 72
Factors Of 91
Factors Of 252
Factors Of 288
Factors Of 362
Factors Of 576
Factors Of 720
Factors Of 770
Factors Of 850

Images/mathematical drawings are created with GeoGebra.