• Home
  • >
  • Converting 0.44444 Repeating as a Fraction: Solutions and Examples

Converting 0.44444 Repeating as a Fraction: Solutions and Examples

Converting 0.44444 repeating as a fraction titleWriting 0.44444 repeating as a fraction is equivalent to $\frac{4}{9}$. You might be wondering how we arrive with $\frac{4}{9}$ as the fraction equivalent to the decimal 0.44444, repeating terms. Follow our step-by-step guide to transforming decimals with repeating and nonterminating terms. Learn how to quickly convert this type of decimal with actual examples.

Why 0.44444 is a Repeating Decimal?

Decimal numbers with terms or one or more numbers after the decimal point that repeats infinitely are called repeating or recurring decimals. These decimals have one or more digits that form a pattern that is repeating and nonterminating.0.44444 repeating is a repeating decimal because digit 4 is repeated without termination in the decimal. Similarly, 0.316316316 repeating is also another example of a recurring decimal because the digits 316, in this specific order, repeat infinitely in the given decimal.If these decimals go on forever repeating their digits, is there another way to write or denote a repeating decimal without indicating the word “repeating”? Yes, of course, there is.In denoting recurring decimals, we often write three dots or “…” after repeating the digit or pattern a few more times to indicate that the same digit or pattern before the dots repeats and goes on infinitely.

Solution 1

Check the example below to better understand the solution:
  • Instead of writing 0.44444 repeating, we could reduce repeating the digit 4 by a few and affix the dots after. It could be simply written as 0.444…..
  • The decimal 2.1333… is a recurring decimal where the digit 3 is repeated.
  • Note that the repeating decimal 0.267267… repeats the pattern 267 infinitely.
Another way, or could be a simpler way, of writing these decimals is by drawing an overline on the digit or terms that repeat in the decimal. Note that the overline should only include the pattern that recurs in the decimal.

Solution 2

For a detailed example, read further:
  • We could simply write 0.44444… as $0.\overline{4}$.
  • The decimal 3.145555… can also be written as $3.14\overline{5}$. Since 5 is the only digit that is repeated throughout the decimal, the overline will be placed only on the digit 5.
  • Consider the decimal 0.189189…, the term 189 is repeated, so we can rewrite the decimal into $0.\overline{189}$.
Note that these decimals are nonterminating so you might ask, “Since the terms repeat endlessly, is there a way we could convert it into a simpler format?” Yes. We can make our recurring decimals look more simple and that is by finding their equivalent in fractions. You will be surprised at how plain and simple these decimals look in their fraction form.
Now that we know how to represent recurring decimals, let’s now proceed with learning how to transform them into fractions.

How to Convert Recurring Decimals to Fractions?

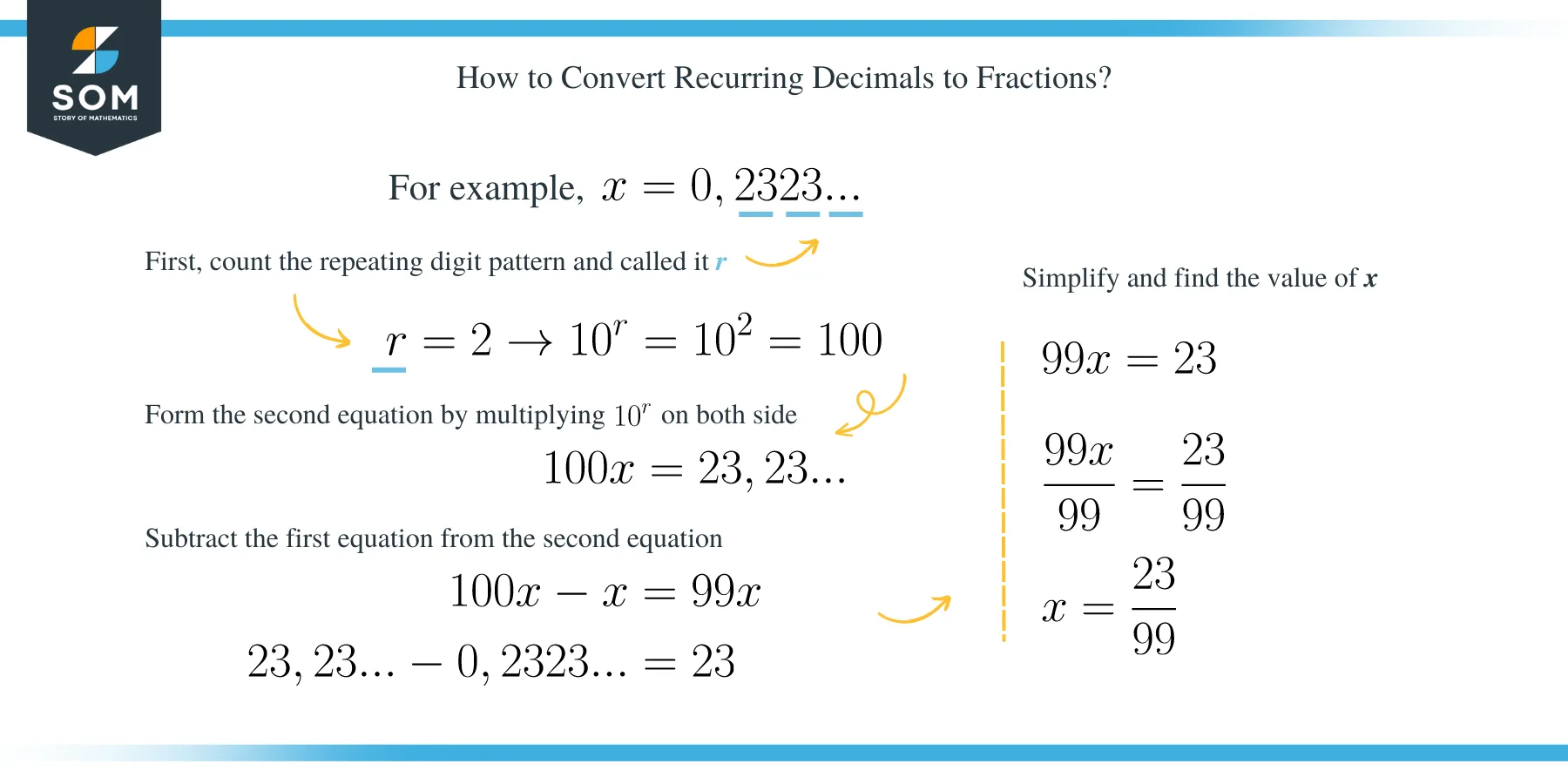
A nonterminating decimal with repeating terms can be converted into its equivalent fraction by following these five easy steps.
  • Step 1. Equate the decimal to a variable, say $x$, to form the first equation.
  • Step 2. Count the digits in the pattern that is repeated throughout the decimal.
  • Step 3. Say $r$ is the number of the digits that forms a recurring pattern in the decimal.
  • Step 4. Form the second equation by multiplying $10^r$ on both sides of the first equation.
  • Step 5. Subtract the first equation from the second equation.
  • Step 6. Solve for the value of $x$ from the resulting equation in the previous step. How To convert 0.444 Step
We can see that the steps we need to take are far from how we transform a terminating decimal into a fraction. Because the recurring decimals are nonterminating, we need to come up with a solution that we could eliminate the repeating terms in the decimal. By doing this, we are able to simplify the numbers that we get so we could convert them into their respective fractions. Let’s apply these steps to transform the recurring decimal 0.44444 as a fraction in the simplest form.

How Recurring Decimal Looks in Its Simplest Form?

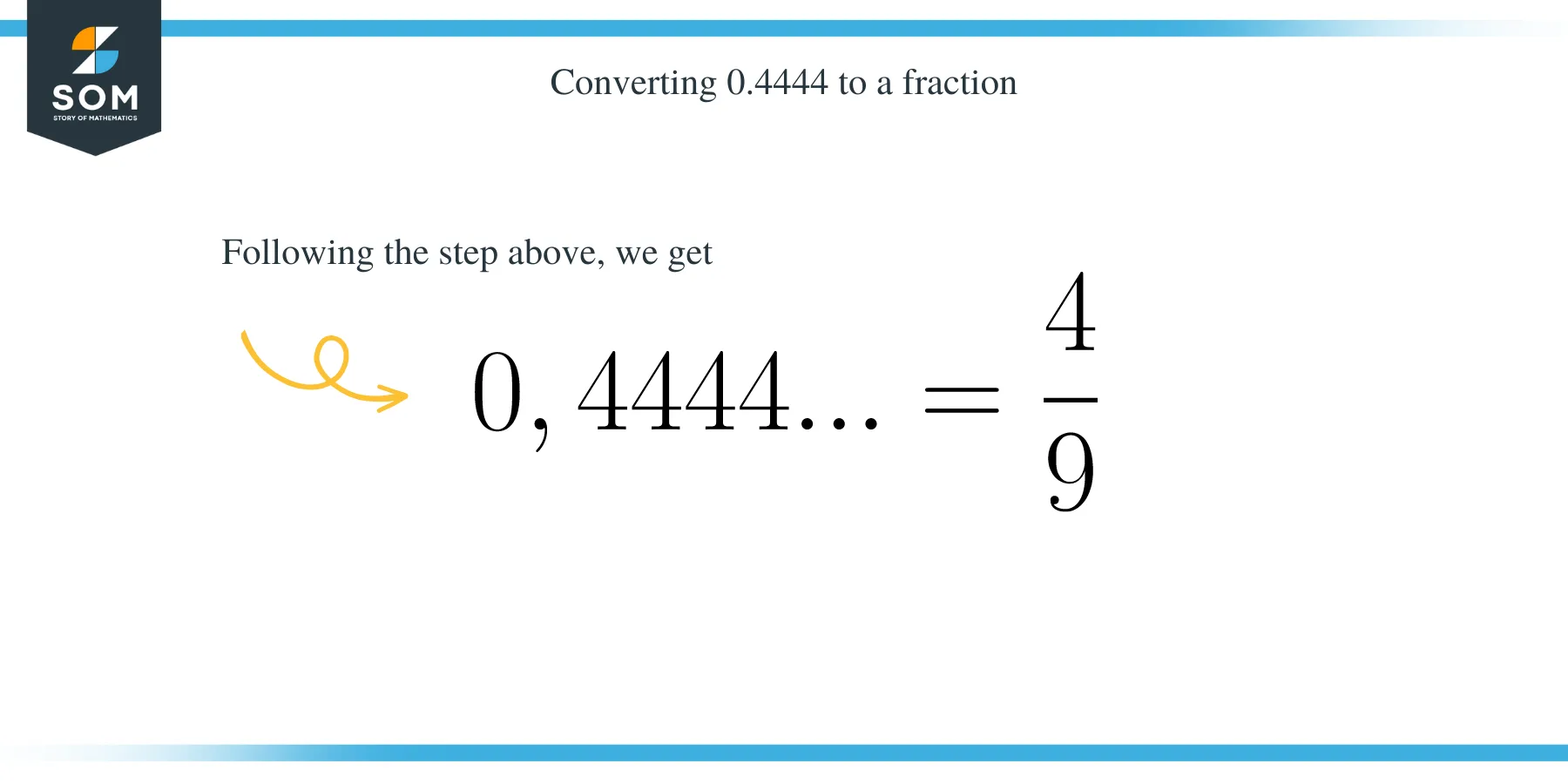
First, we form the first equation by assigning $x$ equal to 0.444…. \begin{equation} x=0.444… \end{equation}We know that only digit 4 is repeated in the decimal. So, we have $r=1$, since only one digit is repeated. Thus, we have $10^r =10^1=10$. So, we multiply 10 on both sides of the first equation.\begin{align*} 10x&=100.444…\\ 10x&=4.444… \end{align*}Now, we subtract the first equation from the second equation. Note that $10x-x=9x$ and $4.444…-0.444…=4$. Thus, the resulting equation is $9x=4$. Finally, solving for, we get\begin{align*} \dfrac{9}{9}x&=\dfrac{4}{9}\\ x&=\dfrac{4}{9}. \end{align*}Since $x$ is both equal to 0.44444… and $\dfrac{4}{9}$, then the decimal 0.44444… is equal to the fraction $\dfrac{4}{9}$.How To convert 0.444 ResultNotice that 0.11111 repeating as a fraction is $\dfrac{1}{9}$, 0.22 repeating as a fraction is $\dfrac{2}{9}$, and 0.55555 repeating as a fraction is $\dfrac{5}{9}$. Similarly, 0.6666 repeating as a fraction is $\dfrac{2}{3}$ or $\dfrac{6}{9}$. Do you see the pattern now? If a decimal has only one repeating digit, then its fraction has the denominator 9, and the numerator is the repeated digit in the decimal.Since we have determined the pattern for the equivalent fraction of those decimals with only one repeating digit like $0.\overline{1}$, $0.\overline{2}$, and so on. Here is a question for you: following this pattern, does it mean that the recurring decimal 0.9999… is equal to $\dfrac{9}{9}$, which is equal to one?Let’s check another example of converting a recurring decimal into a fraction such that the number of digits in the repeating pattern is more than one.

Example

Let’s follow the steps that we presented in converting $0.\overline{267}$ into a fraction.
  • Step 1. We formulate the first equation by equating $x$ to $0.\overline{267}$.\begin{align*} x=0.\overline{267} \end{align*}
  • Step 2. We count the number of repeating digits in the decimal. Since the repeated term in the decimal is 267, which has three digits, then $r=3$.
  • Step 3. We multiply both sides of the first equation by $10^r$. Since $r=3$, then $10^r=10^3=1,000$. Note that the decimal $0.\overline{267}$ can be further expanded to $0.267\overline{267}$. Thus, the second equation is given by this equation.\begin{align*} 1,000x&=1,000\times 0.267\overline{267}\\ 1,000x&= 267.\overline{267} \end{align*}
  • Step 4. We form another equation by getting the difference between the two equations we obtained.\begin{align*} 1,000x&= 267.\overline{267}\\ -(x&=0.\overline{267})\\ \Rightarrow999x &= 267 \end{align*}
  • Step 5. From the equation $999x=267$, we solve for the value of $x$. \begin{align*} \dfrac{999x}{999}&=\dfrac{267}{999}\\ x&=\dfrac{267}{999}=\dfrac{89}{333} \end{align*}
This means that $0.\overline{267}$ is equal to the fraction $\dfrac{89}{333}$.

How to Convert Recurring Decimals to Percents?

So we are done learning how to transform a recurring decimal into a fraction. Let’s now explore how to convert these decimals into percent format. Note that it’s a lot easier than the previous discussion.Transforming recurring decimals to percent is more straightforward compared to when converting them to a fraction. We only need to multiply the decimal by $100\%$, and then we already have the percent equivalent of the recurring decimal. We can mathematically represent it using the following formula. Say $y$ is a recurring decimal, then the formula is given by $y\times100\%$.If you want to do it more quickly, just move the decimal point two places to the right and affix the percent sign ($\%$). Let’s take a look at these examples to illustrate this better.

Example

To better understand our explanation, check the detailed example below:
  • 0.44444 repeating as a percent is $44.444…\%$. We move the decimal point two places from the right, so we place the decimal point after 44 and see that digit 4 still repeats infinitely in the decimal part.
  • We solve for the percent form of the number $3.\overline{189}$. Note that $3.\overline{189}$ is equivalent to $3.189\overline{189.}$ Thus, multiplying this number by $100\%$ gives us $31.89\overline{189}$. Another note in this example is that we should be careful about where we place the overline.

FAQ

We gathered some questions related to this topic to give you a better understanding of the areas we are not able to discuss.

Is 0.44444 a Rational Number?

Yes. The recurring decimal 0.44444… is rational because we can always solve for its equivalent fraction. Indeed, every recurring decimal is rational because we can always express them as a ratio of two integers.

Do Repeating Decimals Exist in Reality?

Yes, of course, they do. These decimals exist, even if the numbers or terms are repeatedly infinitely because they are a representation of their equivalent fractions.

Which Decimals Are Not Considered Repeating?

Terminating decimals, decimals with finite repetitions, and nonrepeating nonterminating decimals are not recurring decimals. If the repetition of the digits or pattern in the decimal stops at some point, then it is not a recurring decimal. Regardless of how many repetitions in the digits or patterns were present in the decimal, it is not a recurring decimal if the pattern terminates after some repetitions.

Conclusion

Transforming decimals with repeating terms might look like a very tedious task. But in this article, we learned how to do it one step at a time so that we cannot miscalculate and give the wrong equivalent fractions to these decimals. Below, we listed down some of the important points we pick up in this article.
  • Recurring decimals are decimals with repeating digits or patterns. These repetitions go on infinitely.
  • We can always convert any repeating decimal into its fraction form by following the steps we specified.
  • We can solve the percent form of any recurring decimal by moving the decimal point two places to the right and affixing the percent sign after.
  • All recurring decimals are rational.
  • If a decimal has only one repeating digit, then its fraction has the denominator 9.
Using the steps we provided, you can practice transforming any recurring decimal into its fraction form and percent form.