To graph a polynomial function, I always start by determining its degree, which tells me the maximum number of turns the graph can have.
For example, a polynomial function of degree $n$ can have at most $n-1$ turning points. The graph of these functions is always continuous, which means it can be drawn without lifting my pencil off the paper.
This continuity reflects the predictability and smoothness of polynomial functions, which range from simple linear equations to more complex expressions with higher powers.
Understanding the degree helps me predict end behavior; for a polynomial of degree $n$, if $n$ is even, the ends of the graph will point in the same direction – either both up or both down.
If $n$ is odd, the ends will point in opposite directions. Another fundamental aspect I look at is the leading coefficient, as it influences whether the graph opens upwards or downwards.
A zero’s multiplicity affects how the graph behaves at those points: If it’s odd, the graph will cross the $x$-axis, and if it’s even, the graph will touch and rebound off the axis. Stick around as I reveal more insights on translating the elegant symmetry of polynomials onto a graph!
Steps Involved in Graphing Polynomial Functions
Graphing a polynomial function involves several steps. I always start by identifying the degree of the polynomial, which gives me a hint on the function’s shape and the number of turning points.
Firstly, I determine the end behavior of the function. The sign of the leading coefficient and the degree tell me how the function behaves as $x$ approaches positive or negative infinity.
If the degree is odd, the ends of the graph go in opposite directions. For an even degree, both ends go in the same direction. I apply the Leading Coefficient Test which states if $a > 0$, the right end of the graph rises, and if $a < 0$, it falls.
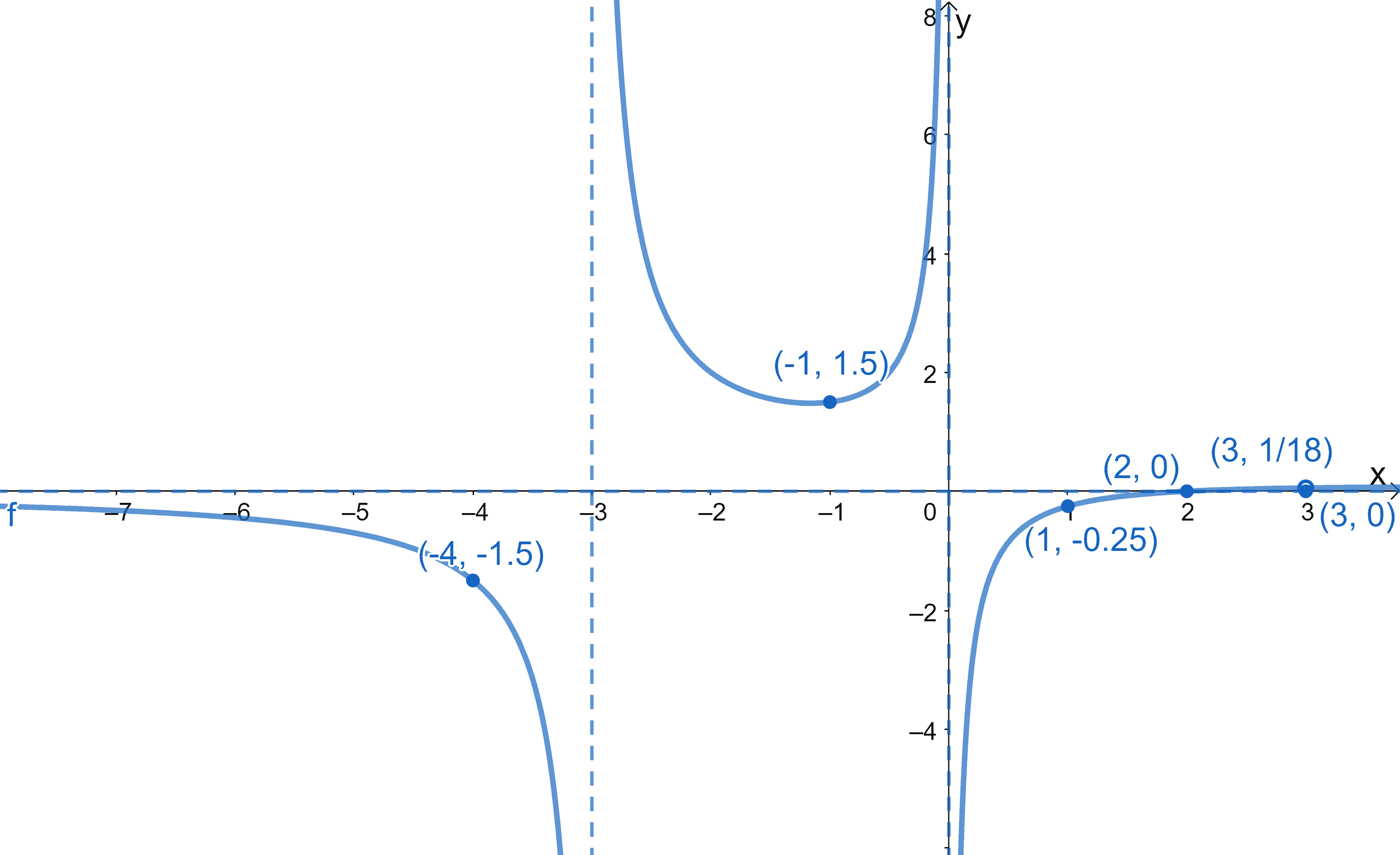
Next, I find the zeros or $x$-intercepts of the function by setting the function to zero and solving for $x$. The multiplicities of these zeros indicate how the graph behaves at these points. A zero with even multiplicity means the graph touches the $x$-axis and turns around, while a zero with odd multiplicity means the graph crosses the $x$-axis.
I also check for symmetry. If the function is an even function, its graph is symmetric with respect to the $y$-axis. This means $f(-x) = f(x)$. An odd function displays symmetry about the origin, so $f(-x) = -f(x)$.
Lastly, I plot points, draw the smooth curve representing the polynomial, and confirm it aligns with the identified end behavior, intercepts, and symmetries.
Here’s a table summarizing the steps:
| Step | Action |
|---|---|
| 1 | Determine degree and leading coefficient for end behavior. |
| 2 | Find and plot the zeros; note multiplicities. |
| 3 | Check and plot symmetry for even/ odd functions. |
| 4 | Plot additional points and draw the graph. |
By following these steps, I can accurately graph any polynomial function.
Graphing Techniques for Polynomial Functions
When I approach graphing polynomial functions, I start by identifying the x-intercepts and the y-intercept.

The x-intercepts are the values of ( x ) where the function equals zero (the roots of the polynomial equation). To find them, I either factor the polynomial or use technology. The y-intercept is simply the point where the graph crosses the y-axis, found by evaluating the function at ( x = 0 ).
Next, I consider the turning points. These are points where the graph changes direction. The number of turning points of a polynomial function is at most one less than its degree.
Polynomial functions exhibit a continuous and smooth curve; there are no sharp corners. Using a table of values can support my graphing process, particularly when I’m using graphing technology like calculators or software to plot the points.
Here’s how I visualize the key components in a table:
| Feature | Description | How to Find |
|---|---|---|
| X-Intercepts | Where the graph crosses the x-axis | Factor/Solve ( f(x) = 0 ) |
| Y-Intercept | Where the graph crosses the y-axis | Evaluate ( f(0) ) |
| Turning Points | Maximums and minimums on the graph | Use calculus or graphing technology |
Remember, while intercepts and turning points are important, they are just part of the story. The end behavior—how the graph behaves as ( x ) approaches infinity or negative infinity—also reflects the degree and leading coefficient of the polynomial.
Throughout the process, I interpret the graph as a continuous function with smooth curves, which gives me a better sense of the function’s overall shape.
Conclusion
In graphing polynomial functions, I’ve found success by following a clear, methodical process. Identifying the x-intercepts, or zeros, is typically my starting point. Using the equation $f(x) = 0$, I solve for the values of $x$ where the function intersects the x-axis. The graph will either cross the axis or touch it at these points, dictating its overall shape.
Once the zeros are marked, I pay close attention to the multiplicity of each zero. A zero with an even multiplicity means the graph will touch and turn at the axis, as opposed to an odd multiplicity where it will cross.
The number of turning points in a polynomial of degree n is also crucial; there will be at most $n-1$ turning points, offering a clue to the overall number of peaks and valleys.
A final aspect I consider is the end behavior, which hinges on the degree and the leading coefficient of the polynomial. If the polynomial is of even degree with a positive leading coefficient, the ends of the graph will point upwards.
Conversely, should the leading coefficient be negative, the ends will face downwards. Armed with these touchstones — zeros, multiplicity, turning points, and end behavior — I find sketching the accurate shape of a polynomial function much more intuitive.
By focusing on these core aspects, I’ve managed to graph polynomial functions reliably, and I hope these tips will aid you as they have me.
With practice, the complex curves and lines begin to form a coherent and predictable pattern, revealing the beautiful symmetry and behavior intrinsic to polynomials.
