JUMP TO TOPIC
The course Calc 4 or Calculus 4 may differ in every institution that offers or teaches the course. It involves a wide range of branches or subfields of calculus necessary in the further understanding of the vast field of calculus. Calculus is a certain branch of mathematics that deals with continuous change. In this complete guide, we’ll discuss the different sides of calculus 4 and what to expect when you go through the course.
What Is Calc 4?
According to Thomas Edison State University, Calculus 4 is an intensive, higher-level course in mathematics that builds on Calculus 2 and Calculus 3 and focuses on the calculus of real-and-vector valued functions of one and several variables. The topics that will be discussed in this course are infinite sequences and series, convergence tests, power series, Taylor Series, and polynomials and their numerical approximations.
Type of Calculus
Most probably when you’re going to take up calculus 4, you already have taken up a series of calculus courses beforehand, and calc 4 is just a continuation of these other courses. It could also be taken alongside other calculus courses that is not a prerequisite of Calculus 4.
Since we already mentioned that Calculus 4 is not universal and definitely will vary depending on the university or school you are in, we list some of the possible calculus course that will be assigned to you when you enroll in Calc 4.
• Differential Calculus
• Integral Calculus
• Vector Calculus
• Multivariable Calculus
• Complex Calculus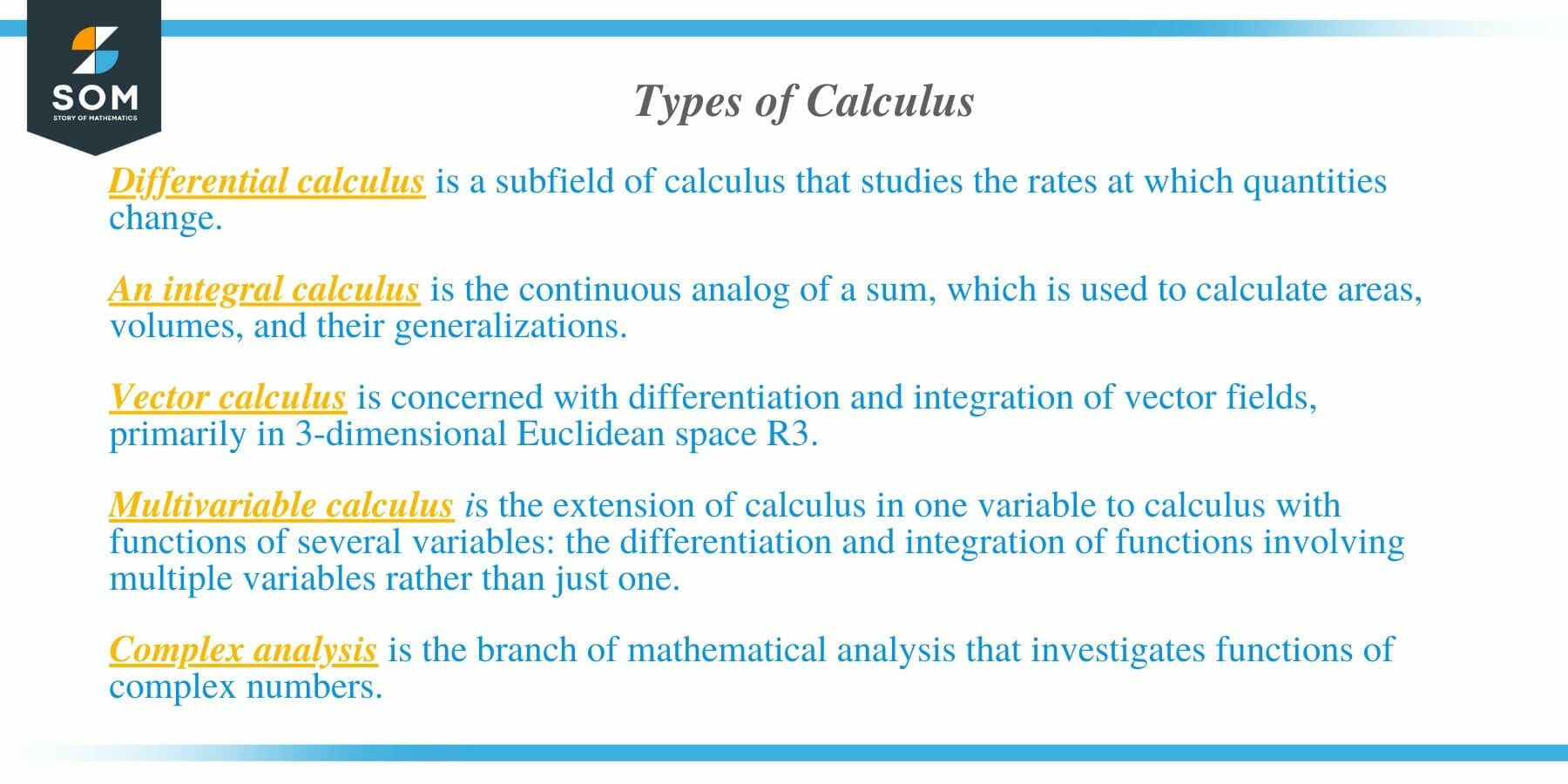
Most of the time, Vector Calculus and Multivariable Calculus are considered the same or will belong in one course. Calculus 4 will fall under higher calculus since it is already the 4th calculus you will take. Thus, it is not possible for calc 4 to be Basic Calculus or other fundamental calculus subfields.
We will try to dissect each calculus subfield that may be your next Calculus 4.
Differential Calculus
Differential calculus focuses on investigation of the methods used in solving first- and second-order ordinary differential equations, systems of differential equations, Laplace transforms, and power series problems.
The course will highlight the following lessons:
- Fundamental techniques in solving first-order and higher-order differential equations that includes linear and non-linear
- Mathematical modelling
- Laplace Transforms generated as a tool in solving differential and integral equations
- Eigenvector analysis utilized in finding solutions to linear systems of differential equations
- Power series
Among the optional subjects are:
- Fourier Series
- Partial Differential Equations
Integral Calculus
Integral calculus is another component of calculus that is focused on the consequences, uses, and theories involving integrals. It is heavily concerned with area and volumes that can be graphed in a coordinate plane. The fundamental theorem of calculus, which demonstrates how a definite integral is determined by employing its antiderivative, connecting the two disciplines: differential and integral calculus.
Vector Calculus
Vector calculus is a certain branch of calculus that thrives on the differentiation and integration of vector fields, mainly applied on three-dimensional Euclidean space. Most of the time, vector calculus is used as a shorthand for the more general area of Multivariable Calculus. Moreover, vector calculus also deals with integrals particularly line integrals and surface integrals.
What is the Vector-Valued Function?
The vector-valued function is a function $r$ where the domain is the set of real numbers $t$ and the range is the set of vectors $r(t)$. The vector $r(t)$ is in the form:
\begin{align*}
r(t)=\langle f(t),g(t)\rangle=f(t)i+g(t)j
\end{align*}
or
\begin{align*}
r(t)=\langle f(t),g(t),h(t)\rangle=f(t)i+g(t)j+h(t)k
\end{align*}
where $f$, $g$, and $h$ are real-valued functions.
The vector-valued function defines curve in a 3D space by actually defining vectors from the origin that point to all the points on the curve for values of $t$.
Example 1
Consider $r(t)=4 cos(t)i+3 sin(t)j$. This function can be written as:
\begin{align*}
r(t)=\langle4 cos(t),3 sin(t)\rangle.
\end{align*}
Since $4 cos(t)$, and $3 sin(t)$ are defined in the set of real numbers, thus the domain for the function $r$ is the set of real numbers.Now, we know that the range of $cos(t)$ for all real numbers $t$ is $[-1,1]$, this follows that the range for $4 cos(t)$ is $[-4,4]$. For $sin(t)$, the range is $[-1,1]$, hence the range of $3 sin(t)$ is $[-3,3]$.
Therefore, the range of $r(t)$ is the set of vectors containing $\langle a,b\rangle$, where $a\in[-4,4]$, and $b\in[-3,3]$.
Example 2
Some Calc 4 Textbooks You Can Use
We provide some of the textbooks that might help you with your studies in Calculus 4.
- CLP-4 Vector Calculus by Joel Feldman, Andrew Rechnitzer, and Elyse Yeager, 2017-21
- Introduction to Differential Calculus: Systematic Studies with Engineering Applications for Beginners by Ulrich L. Rhode, G. C. Jain, Ajay K. Poddar, and A. K. Gosh, 2011
- Vector Calculus by Paul C. Matthews, 1998
- Calculus by James Stewart, 2015
Take note that before choosing a calculus 4 textbook, check the course content and check whether the topics listed are covered in the textbook. This is to maximize the aid of your textbook in your studies.
Difficulty
Calculus, in its nature, is a very difficult course to take yet rewarding once completed. Thus, whether it is hard or not, it is still subjective and depends on the students’ effort and willingness to learn the course. It is important that you are well armored by your previous calculus courses before taking up Calc 4.
Conclusion
We have provided a brief but functional definition of possible Calculus 4 courses. Though the course is a varying subject to others, we can agree that Calculus 4 is an extensive exploration of numbers. Here are some of the important points tackled in this guide.
- Calculus 4 is a course proceeding previous calculus courses and may cover Differential calculus, Integral calculus, or Vector calculus.
- Differential calculus deals mainly on the dynamics and solutions of differential equations.
- Integral calculus focuses on integration techniques and its application on areas and volumes.
- Vector calculus is concerned with analysis, differentiation, and integration applied on vector fields.
We encourage you to explore these topics yourself — there’s an untapped world of mathematical discovery waiting for you!