 In general, trigonometry is considered hard, especially when right triangle numerals are given as word problems.
In general, trigonometry is considered hard, especially when right triangle numerals are given as word problems.
However, an exact answer to this question depends upon a number of factors as some people find trigonometry hard while others think it to be relatively easy. In many cases, students don’t comprehend the problem properly, which creates all the difficulties if the problem itself is quite easy and straightforward.
In this article, we will discuss the features or course outlines which make trigonometry hard for some students and share some tips on how to overcome these difficulties.
Is Trigonometry Hard?
Trigonometry is hard for some students while others find it easy. Science students learn trigonometry at the school level, while complex or advanced trigonometry is taught in high school. High-level trigonometry is unfortunately hard for students as it contains many formulas and becomes complex, especially when we have to find the unknown angles and values of multiple connected triangles.
Students often ask questions such as,: “Is trigonometry harder than statistics?” “Is trigonometry geometry?” “Is trigonometry harder than geometry?” “Why is trigonometry so confusing?” “Is trigonometry important?” etc.
Let us first discuss what trigonometry means and its significance, and then we will discuss the reasons which make trigonometry hard. Hopefully, our explanation will clear out most of the questions we mentioned above.
Trigonometry
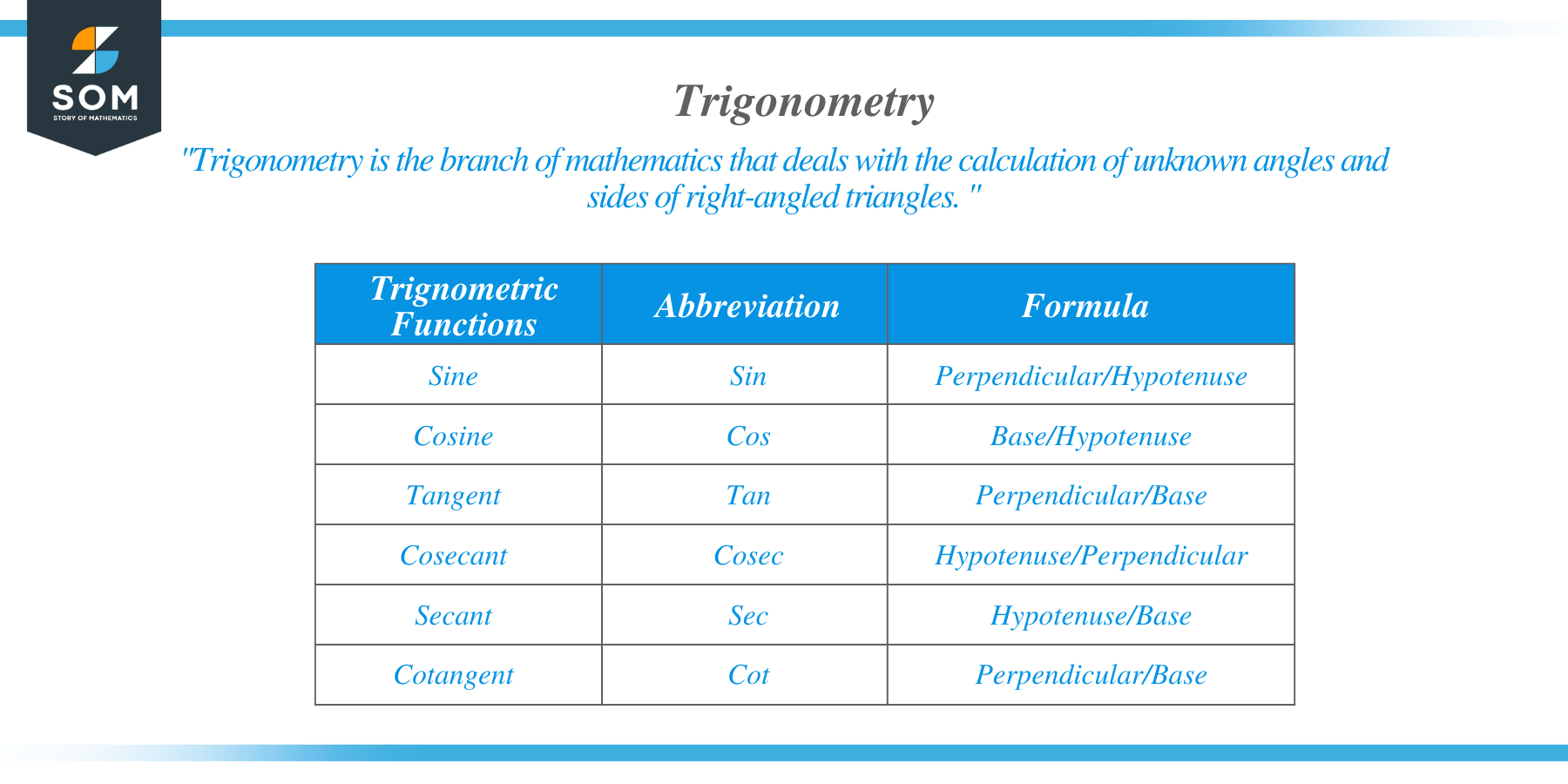
Trigonometry is the branch of mathematics that deals with the calculation of unknown angles and sides of right-angled triangles. The Greek mathematician Hipparchus introduced the concept of trigonometry and it evolved over time.
Trigonometry defines six different ratios for a right-angle triangle. Using these ratios, we can find out the unknown values of the angle and sides in a right-angle triangle. The names of these six ratios are:
- Sine
- Cosine
- Tangent
- Secant
- Cosecant
- Cot
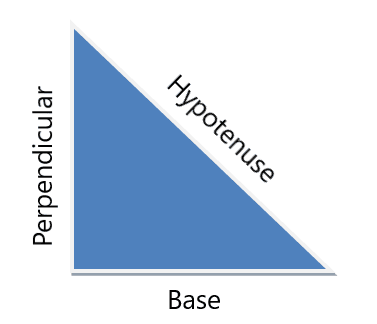
The definitions of these ratios are given in the table below. We can use these definitions to determine the sides and angles of a right-angle triangle. For example, if the angle between the base and hypotenuse is “x,” then it can be determined by using the ratio $tan(x) = \dfrac{perpedicular}{base}$ or $cos(x) = \dfrac{base}{hypotenuse}$.
Let us now discuss the reasons which make trigonometry difficult.
Difficulty of Trigonometry
Trigonometry is considered hard by students due to the following reasons:
- The memorization of formulas and values
- Non-linear functions
- Angle measurement in Radians/Degree
- Polar and Cartesian coordinates
- Unit Circle Calculations
- Lengthy and Complex Calculations
- Domain and Range of trigonometric functions
- Visualization
The Memorization of Formulas and Values
In order to be efficient in solving trigonometric problems, it is essential to memorize many formulas along with formulas and values of the trigonometric ratios. For example, you will have to learn the values of sin, cos, tan, cot, cosec and sec at angles of $0^{o}$, $30^{o}$ ,$60^{o}$,$90^{o}$ along with other formulas.
After learning the basic formulas, students then have to memorize long and complex formulae such as the law of cosines and the law of sines, etc., and you cannot solve most of the problems in exams unless you have learned the formulas by heart.
Learning all these formulas is a bit tedious, but instead of cramming them, a simple workaround is to do lots of practice. If you regularly solve trigonometric questions, you will realize that you remember all the formulas effortlessly.
Non-linear Functions
As already discussed, trigonometry defines six different ratios. If we plot these ratios as a function of the angle $\theta$, we get non-linear functions, and non-linear functions are more challenging to work with as opposed to linear functions, making it hard for students to solve questions related to trigonometry.
Also, unlike simple algebra where you use similar formulas to solve most of the problems, in trigonometry, we have varied formulas and each question requires a unique application of these formulas to arrive at the solution. This can be confusing to the students when they first approach trigonometry. However, again, with practice, these difficulties appear to melt away, and you start to enjoy the fact that each question has its own flavor.
Angle Measurement in Radians/Degrees
It is already tough for students to solve trigonometric equations involving angles with degrees but when they have to convert answers to radians or radians to degrees, it just makes the problem more complex. To convert to degrees from radians, you have to multiply your answer with 180 and then divide it by $\pi$ and conversely, when you convert from degree to radians, you multiply the value with $\pi$ and then divide it by 180.
A simple mistake or confusion in the conversion of angles can alter the values of all trigonometric functions resulting in incorrect solutions.
In some questions, you are allowed to use a calculator. You have to be mindful if the mode of the calculator is set to radians or degrees and you would have to re-adjust the mode based on the question that you are solving. It is a common mistake for students to use the incorrect calculator mode while solving trigonometric questions, resulting in incorrect answers.
Note that the conversion between radians to degrees is not hard in itself. The difficulty lies in attention to detail. So when solving questions, keep on asking yourself if you are working with radians or degrees and if you encounter calculations with very large or very small numbers, it is better to check if you are working with the correct units of angle.
Polar and Cartesian Coordinates
The formulas and non-linear functions alone are tough enough for students, but to make the matter more complex, the students must have a solid background in the polar and cartesian systems. For example, students must know what is an ordered pair and what is meant by the coordinate points. If a point $(-3,2)$ is given, the student should know the value of “$x$” and “$y$” coordinates, and furthermore, he should know in which coordinate this point lies in the cartesian system.
Trigonometric questions use the Cartesian system coordinates to solve the problems, so if you are not familiar with the cartesian system and even if you know the trigonometric functions, you won’t be able to solve the problems.
Initial or beginner-level problems related to trigonometric equations require an understanding of the Cartesian system, but as you go further and study advance level trigonometric systems, you will also have to deal with a polar coordinate system. The polar coordinate system has its alternative for $x$ and $y$ coordinates as “$r$” and “$\theta$”.
The polar coordinate system uses radians or degrees while plotting a function, so students not only have to deal with the conversion from cartesian coordinate to polar coordinate, but they also have to deal with radian to degree and the degree to radian conversion when dealing with polar coordinates. This conversion, along with the trigonometric functions, makes trigonometry complex.
Unit Circle and Triangles
Trigonometry makes a lot of use of the unit circle. A unit circle is a circle having a radius of 1. Trigonometry uses the unit circle in many of its problems, and then you have to solve for the triangles inside the unit circle.
The problem becomes complex when you start dealing with a circle having a radius greater than 1. In Trigonometry, many assumptions are made while dealing with problems involving a unit circle so such problems become complex, and if students don’t remember the basic function of a unit circle, then they will find it very hard to solve trigonometric problems involving a unit circle.
Lengthy and Complex Calculations
Trigonometry hard questions involve lengthy and complex calculations. Some of the calculations in trigonometry can become quite long and students who like it short and easy will find it difficult to solve such problems.
The problems become lengthy because of the calculations of all sides and angles of a given function or triangle, and to make matters worse, you might also have to deal with the conversion from radian to degree or cartesian to polar coordinates. Some students just get confused by the sheer length of the problems in Trigonometry. It should be remembered that while the questions might be long, they involve the same calculations over and over and a little practice and patience from the students will definitely help them overcome the difficulty.
Domain and Range of Trigonometric Functions
The domain and range of any function are the input and expected output values of the function, and the same is the case with trigonometric functions. The domain of the trigonometric function is the value of the angles used in any of the six trigonometric functions, while the resultant value will be the range. Note that the trigonometric ratios become the trigonometric functions if we view them as a function of the angle $\theta$.
The values of angle can have a variety of range values as they can be positive or negative, so the range changes according to that, and to make the matter more difficult, students not only have to deal with domain and range of normal functions, they also have to find out the domain and range of the inverse of six trigonometric functions. For example, the domain and range of $tan(\theta)$ is $R – (2n+1) \dfrac{\pi}{2}$ and $(-\infty,\infty)$ respectively while the domain and range of $tan^{-1}(\theta)$ is $(-\infty,\infty)$ and $( -\dfrac{\pi}{2}, \dfrac{\pi}{2})$.
We have mentioned only the domain and range of a general $tan(\theta)$ and its inverse function, and when we put in the value of $\theta$ and we have to convert it from radians to degree or vice versa, things will surely get complicated. There will be open-end and close-end domains and ranges so students need to know the difference between them as well while solving problems related to finding domains and range of trigonometric functions. So, in short, the more you go deeper into Trigonometry, the harder it becomes.
Visualization
The last and final reason for trigonometry to be confusing and difficult is the concept of visualization. The branch of Trigonometry heavily relies on visualization and visual analysis. As most of the graphs are non-linear and students are required to deduce the properties, domain and range of a given function by looking at the available graph, it becomes a difficult process and it requires good visual analysis skills.
The students with good visual analysis skills will find it easier to comprehend a given graph or to draw the graph by using the calculated values, while students who don’t have good visual analysis skills will find it hard to relate a given problem to a circle, triangles and other non-linear bell shaped graphs.
These are some of the reasons which make Trigonometry so confusing for students, but in general, it is easier than statistics but harder than algebra and geometry.
Conclusion
Let us conclude this topic by revisiting what we have learned so far.
- Trigonometry is a branch of mathematics that uses trigonometric functions to find angles and sides of right-angle triangles.
- Remembrance of various formulas, conversion from radians to degrees, the degree to radians, Cartesian to polar coordinates, along with lengthy calculations, make Trigonometry difficult for some students.
- Beginner-level Trigonometry is not difficult if you memorize the formulas and understand the basics of Trigonometry.
