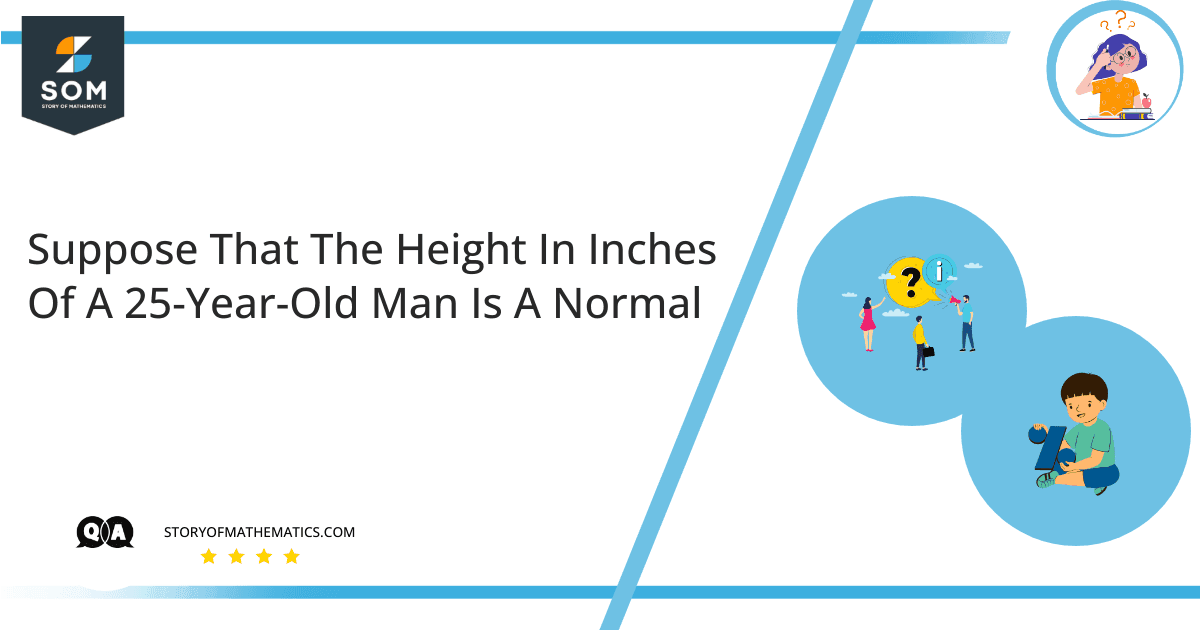
-a) What percentage of 25-year-old men are over $6$ feet, $2$ inches tall?
-b) What percentage of men in the $6$-footer club are over $6$ feet, $5$ inches?
This question aims to explain the mean, variance, standard deviation, and z-score.
The mean is the central or the most common value in a group of numbers. In statistics, it is a measure of the central trend of a probability distribution along mode and median. It is also directed as an expected value.
The term variance directs to a statistical stature of the distribution between numerals in a data set. More precisely, variance estimates how far each numeral in the set is from the mean average, and thus from every other numeral in the set. This symbol: $\sigma^2$ often expresses variance.
Standard deviation is a statistic that estimates the distribution of a dataset relative to its mean and is calculated as the square root of the variance. The standard deviation is computed as the square root of variance by defining each data point’s deviation as compared to the mean.
A Z-score is a numerical measure that defines a value’s connection to the mean of a cluster of values. Z-score is calculated in terms of standard deviations from the mean. If a Z-score is $0$, it indicates that the data point’s score is similar to the mean score.
Expert Answer
Given the mean $\mu$ and the variance, $\sigma^2$ of a $25$-year man is $71$ and $6.25$, respectively.
Part a
To find the percentage of $25$-year-old men that are over $6$ feet and $2$ inches we first calculate the probability of $P[X> 6 feet \space 2 \space inches]$.
$6$ feet and $2$ inches can be written as $74 \space in$.
We have to find the $P[X>74 \space in]$ and it is given as:
\[P[X>74]=P\left[\dfrac{X-\mu}{\sigma}>\dfrac{74-71}{2.5}\right]\]
That is:
\[=P[Z\leq 1.2] \]
\[1-\phi(1.2) \]
\[1-0.8849\]
\[0.1151\]
Part b
In this part, we have to find the height of a $25$-year-old man above $6$ feet $5$ inches given that he is $6$ feet.
$6$ feet and $5$ inches can be written as $77 \space in$.
We have to find the $P[X>77 \space in | 72 \space in]$ and it is given as:
\[ P[X>77 \space in | 72 \space in] = \dfrac{X>77 | X>72}{P[X>72]} \]
\[= \dfrac{P[X>77]}{P[X>2]} \]
\[= \dfrac{ P \left[ \dfrac{X-\mu}{\sigma} > \dfrac{77-71}{2.5} \right]} {P \left[ \dfrac{X-\mu}{\sigma} > \dfrac{72-71}{2.5} \right] } \]
\[ \dfrac{P[Z >2.4]}{P[Z >0.4]} \]
\[ \dfrac{1- P[Z >2.4]}{P[Z >0.4]} \]
\[ \dfrac{1- 0.9918}{1- 0.6554} \]
\[ \dfrac{0.0082}{0.3446} \]
\[ 0.0024\]
Numerical Results
Part a: The percentage of men above $6$ feet and $2$ inches is $11.5 \%$.
Part b: The percentage of 25-year-old men in the $6$-footer club that are above $6$ feet and $5$ inches is $2.4 \%$.
Example
The grades on a math final at school have a mean $\mu = 85$ and a standard deviation of $\sigma = 2$. John scored $86$ on the exam. Find the z-score for John’s exam grade.
\[z=\dfrac{X-\mu}{\sigma}\]
\[z=\dfrac{86-85}{2}\]
\[z=\dfrac{1}{2}\]
\[z=0.5\]
John’s z-score is $0.5$.
