$y=(x+C)(\dfrac{x+2}{x-2})$
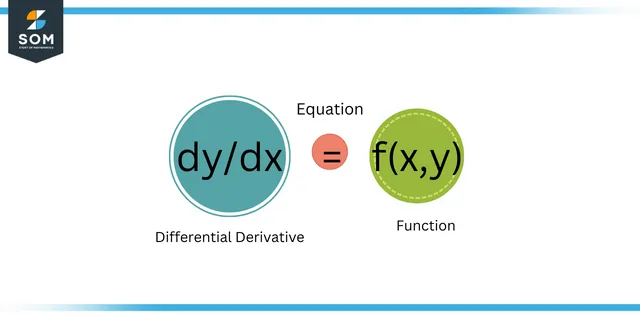
This article aims to find the transient terms from the general solution of the differential equation. In mathematics, a differential equation is defined as an equation that relates one or more unknown functions and their derivatives. In applications, functions generally represent physical quantities, derivatives represent their rates of change, and a differential equation defines the relationship between them. Such relationships are common; therefore, differential equations are essential in many disciplines, including engineering, physics, economics, and biology.
Example
In classical mechanics, the movement of a body is described by its position and velocity as the time value changes. Newton’s laws help these variables to be expressed dynamically (given position, velocity, acceleration, and various forces acting on the body) as a differential equation for the unknown position of the body as a function of time. In some cases, this differential equation (called the equation of motion) can be solved explicitly.
Differential Equation
Types of differential equations
There are three main types of differential equations.
- Ordinary differential equations
- Partial differential equations
- Non-linear differential equations
Ordinary differential equations
An ordinary differential equation (ODE) is an equation containing an unknown function of one real or complex variable $y$, its derivatives, and some given function of $x$. The unknown function is represented by a variable (often denoted $y$), which therefore depends on $x$. Therefore, $x$ is often called the independent variable of the equation. The term “ordinary” is used in contrast to the partial differential equation, which may concern more than one independent variable.
Partial differential equations
A partial differential equation (PDE) is an equation that contains unknown functions of multiple variables and their partial derivatives. (This contrasts ordinary differential equations, which deal with parts of one variable and its derivatives.) PDEs formulate problems involving functions of several variables and are either solved in closed form or used to create the appropriate computer.
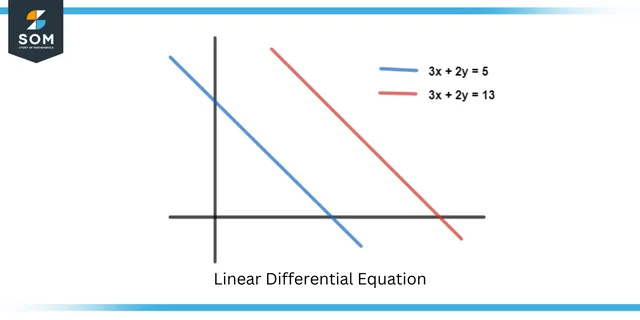
Non-linear differential equations
A non-linear differential equation is an equation that is not linear in the unknown function and its derivatives (linearity or nonlinearity in the arguments of the function is not considered here). There are very few methods for solving non-linear differential equations exactly; known ones typically depend on an equation with particular symmetries. Non-linear differential equations exhibit highly complex behavior in extended time intervals, characteristic of chaos.
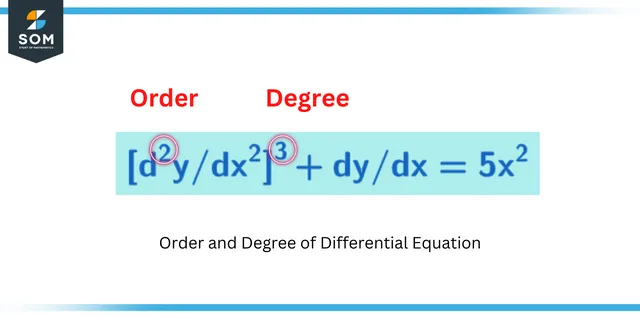
Order and degree of differential equation
Expert Answer
By solving the given equation:
\[y=(x+C)(\dfrac{x+2}{x-2})\]
\[(x+C)(\dfrac{x+2}{x-2})=\dfrac{x^{2}}{x-2}+\dfrac{(2+C)x}{x-2}+\dfrac{2C}{x-2}\]
Take the limits of each of three terms to $x\rightarrow\infty$ and observe which terms approaches zero.
All the three terms are rational expressions, so the term $\dfrac{2C}{x-2}$ is a transient term.
Numerical Result
The term $\dfrac{2C}{x-2}$ is a transient term.
Linear Differential Equation
Example
Find the transient terms in this general solution of the differential equation, if any.
$z=(y+C)(\dfrac{y+2}{y-2})$
Solution
By solving the given equation:
\[z=(y+C)(\dfrac{y+4}{y-4})\]
\[(y+C)(\dfrac{y+4}{y-4})=\dfrac{y^{2}}{y-4}+\dfrac{(2+C)y}{y-2}+\dfrac{2C}{y-2}\]
Take the limits of each of three terms to $x\rightarrow\infty$ and observe which terms approaches zero.
All the three terms are rational expressions, so the term $\dfrac{2C}{y-2}$ is a transient term.
The term $\dfrac{2C}{y-2}$ is a transient term.
