What Is 2i Equal To?

JUMP TO TOPIC

What Is 2i Equal To?
Is 2i a Real Number?
Complex and Imaginary Numbers
- Example
- Example
What Is i Equal To?
What Does -2i Equal in Math?
What Is 2i^2?
What Does i^3 Equal?
Powers of Imaginary Numbers
- Example
Conclusion

Solving what is 2i equal to in algebra requires some knowledge of imaginary and complex numbers. The expression $2i$ is an imaginary number that is one of the roots of the quadratic polynomial $x^2+4$ or the square root of $-4$.

This is because the square root of negative numbers does not exist in the real line. Let us learn more about the other forms of imaginary and complex numbers and what they are equal to in mathematics.

The number $2i$ is an imaginary number equal to the principal square root of $-4$. This means that it is a solution to the quadratic polynomial $x^2+4$. Note that the expression $x^2+4$ has no real solution, which means that we cannot find a real number that can satisfy the equation $x^2+4=0$. This means that $2i$ is equal to the square root of $-4$ because:

\begin{align*}
x^2+4&=0\\
\Rightarrow x^2&=-4\\
\Rightarrow \sqrt{x^2}&=\sqrt{(-4)}\\
\Rightarrow2i&=\sqrt{-4)}.
\end{align*}

Thus, in general, if we have the quadratic expression $x^2+a$ where $a$ is a positive number, then one of its roots is $\sqrt{a}i$. Moreover, it similarly means that $\sqrt{a}i$ is the square root of $-a$. That is:
\begin{align*}
\sqrt{-a}=\sqrt{a}i.
\end{align*}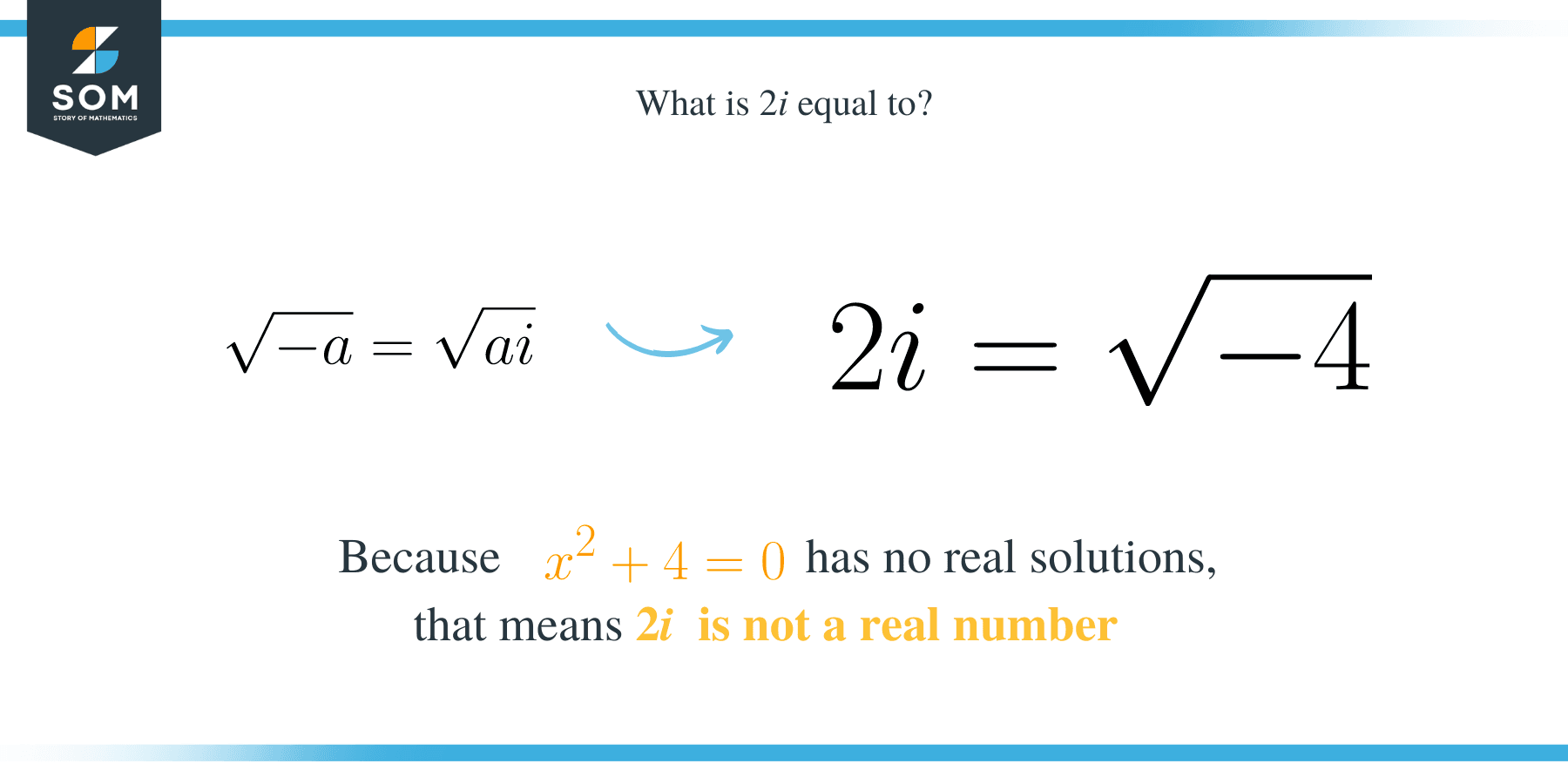

Read in the following sections what $2i$ is and what it represents mathematically.

Is 2i a Real Number?

No, $2i$ is not a real number. Since the equation $x^2+4=0$ has no real solutions, this implies that $2i$ is not a real number. Then what is $2i$ then? In this case, $2i$ is an imaginary number. The number $2i$ is an imaginary number because it has the form $bi$, where $b$ is a real number, and $i$ is the imaginary unit. Take note that $i$ is equal to the square root of $-1$.

The next section will discuss what complex and imaginary numbers are and what their values mean mathematically.

Complex and Imaginary Numbers

Imaginary numbers are numbers that are written as a product of a real number and the imaginary unit $i$. They do not exist in the real line, instead, they are elements of the complex number system.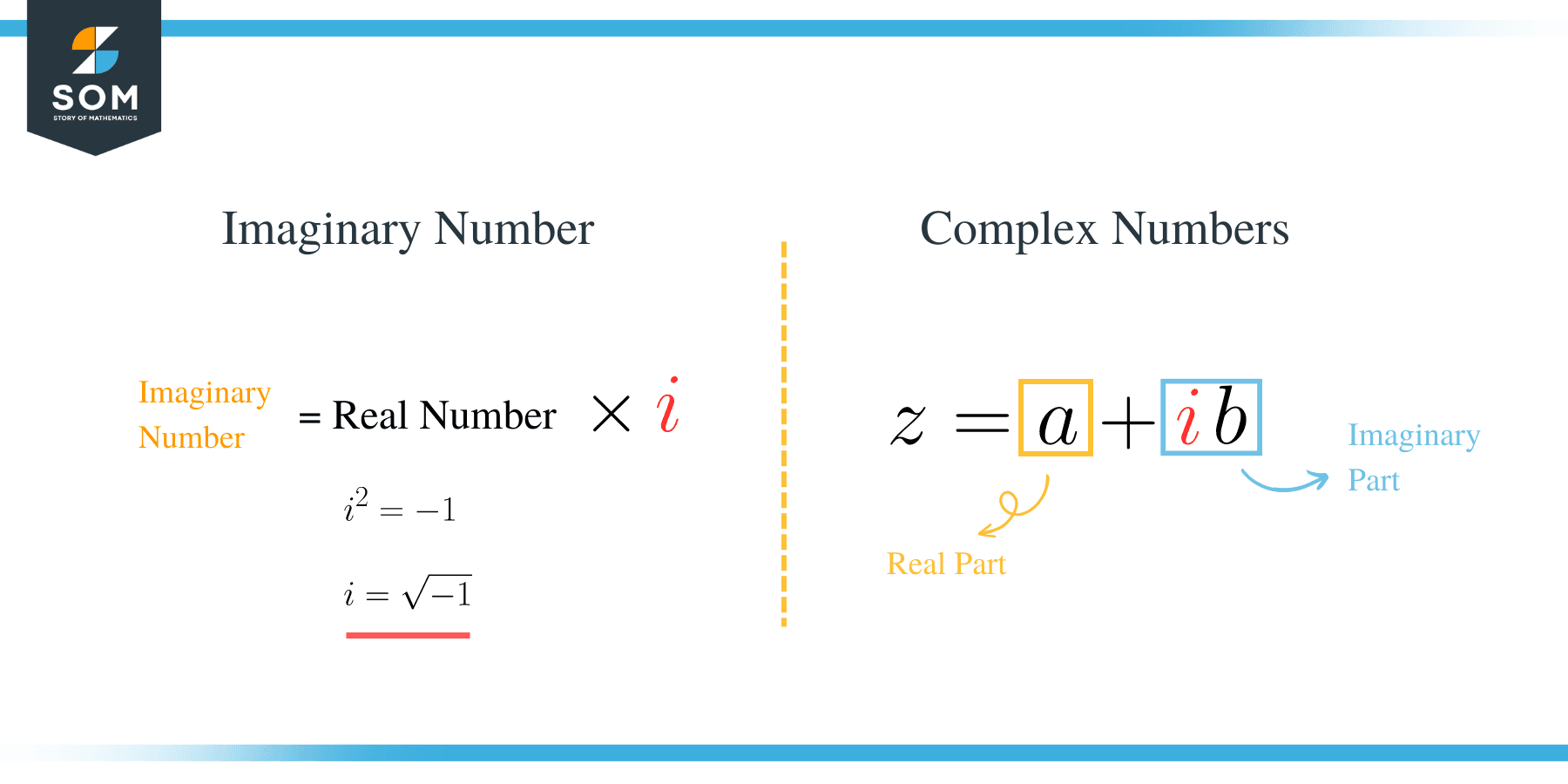

Example

$ei$ is an imaginary number. It is of the form $bi$ where $b=e$ and $e$ is an irrational number, which is also a real number.
$-i$ is also imaginary because it is a product of $-1$, which is real, and $i$. Moreover, the square of $-i$ is $-1$.
Another imaginary number is $\dfrac{i}{3}$. It is the product of $\dfrac{1}{3}$ and $i$.

In general, complex numbers are those numbers that are in the form of $a+bi$, where $a$ and $b$ are real numbers. The expression $a$ is considered the real part, while $bi$ is the imaginary part. Moreover, we can also conclude that imaginary numbers are complex numbers with no real part because: \begin{align*} a+bi&=bi\\ \Rightarrow a&=0. \end{align*}

Example

The imaginary number $ei$ is also a complex number composed only of the imaginary part, which means that it has no real part.
All imaginary numbers are complex numbers.
The number $2-3i$ is a complex number where $2$ is the real part, and $3i$ is the imaginary part.
Another example of complex number is $\dfrac{\sqrt{5}+2i}{3}$.

Even though they are defined as “imaginary,” such numbers are real in the fact that they are defined with a reason and existent in mathematics.

What Is i Equal To?

The imaginary number $i$ is equal to the $\sqrt{-1}$. It is also often termed as the imaginary unit. A real number multiplied by $i$ becomes an imaginary number. We also note that if we take the square of an imaginary number, we will always get a negative number. Thus, the square of $i$ is $-1$.

What Does -2i Equal in Math?

The number $-2i$ is also equal to the square root of $-4$. It is also one of the roots of the quadratic expression $x^2+4$. Note, however, that $2i$ is not equal to $-2i$, but they are both roots of the quadratic equation $x^2+4=0$. Thus, $-2i$ is also equal to $\sqrt-4$. Notice further that if we take the square of $-2i$, we will get $-4$.
\begin{align*}
(-2i)^2&=(-2)^2 (i)^2\\
&=4(-1)\\
&=-4
\end{align*}

What Is 2i^2?

Solving $2i^2$ gives us $-2$. This is because $i^2$ is always equal to $-1$. Thus, $2i^2$ is equal to $-2$. Take note also that $2i^2$ is not equal to or the same as $(2i)^2$. As mentioned earlier, $2i$ is a square root of $-4$ which means that the square of $2i$ is -4. \begin{align*} 2i^2&=2(i^2 )\\ &=2(-1)\\ &=-2 \end{align*}

What Does i^3 Equal?

The power $i^3$ is equal to $-i$. Since $i^2$ is equal to $-1$ and $i^3$ is $i^2$ multiplied by $i$, then this gives us $-i$. The step by step solution to this is as follows: \begin{align*} i^3&=i(i^2)\\ &=i(-1)\\ &=-i. \end{align*}We can make a generalization of the powers of the imaginary unit $i$ in the next section.

Powers of Imaginary Numbers

The powers of the imaginary unit $i$ give us the values $i, -i, 1,$ and $-1$. Let’s learn how it is possible that the powers of $i$ will only revolve within these values. Note that: \begin{align*} i^0 &= 1\\ i^1&=i\\ i^2&=-1 \end{align*} and from the previous section, we learn that: \begin{align*} i^3=-i. \end{align*}Solving the proceeding powers of $i$, we have: \begin{align*} i^4&=(i^2 )(i^2 )=(-1)(-1)=1\\ i^5&=(i^4 )(i)=(1)(i)=i\\ i^6&=(i^4 )(i^2 )=(1)(-1)=-1\\ i^7&=(i^4 )(i^3 )=(1)(-i)=-i\\ i^8&=(i^4 )^2=(1)^2=1\\ \vdots \end{align*}Notice that whenever the power of $i$ is odd, it gives us either $i$ or $-i$. Moreover, if the power of $i$ is even, the resulting number is either $1$ or $-1$. In general, we have this formula for determining the powers of $i$: \begin{align*} i^n = \left\{ \begin{array}{ll} 1 & \text{if }\, n\equiv0 \pmod{4}\\ i & \text{if }\, n\equiv1 \pmod{4}\\ -1 & \text{if }\, n\equiv2 \pmod{4}\\ -i & \text{if }\, n\equiv3 \pmod{4}\\ \end{array} \right. \end{align*}Recall that $n\equiv p \pmod{4}$ means that $p$ is the remainder whenever $n$ is divided by $4$.

Example

The expression $7i^7$ can be further simplified to $-7i$. This is because the exponent of $i$ is $7$, and
\begin{align*}
7\equiv3 \pmod{4}.
\end{align*}
Thus, $i^7=-i$. Hence, we have:
\begin{align*}
7i^7=7(-i)=-7i.
\end{align*}

The simplified expression for $(2i^2 )^4$ is $16$. This can be solved by expanding the expression, which gives us:
\begin{align*}
(2i^2 )^4&=2^4 i^8=16i^8.
\end{align*}
Moreover, note that the exponent of $i$ is $8$, and $8$ is divisible by $4$, which means that
\begin{align*}
8\equiv0 \pmod{4}.
\end{align*}
Thus, $i^8=1$. Therefore, $(2i^2 )^4=16(1)=16$.

The power of a complex number $(1-2i)^4$ can be simplified to a resulting complex number $-7+24i$. The resulting complex number is obtained using the following steps:
\begin{align*}
(1-2i)^4&=1-8i+24i^2-32i^3+16i^4\\
&=1-8i+24(-1)-32(-i)+16(1)\\
&=1-8i-24+32i+16\\
&=-7+24i.
\end{align*}

Conclusion

The importance of complex and imaginary numbers is to mainly serve as solutions to equations whose roots do not exist in the real line. Let’s take a moment to highlight some of the key ideas in this reading so that you can keep your mind clear after all of our discussions.

The imaginary number $2i$ is equal to $\sqrt{-4}$. It can also be understood as the root of the quadratic polynomial $x^2+4$.
Imaginary numbers are numbers that take the form $bi$, where $b$ is a real number and $i$ is the imaginary unit.
All imaginary numbers are complex numbers, and complex numbers are expressed in the form $a+bi$, where $a$ and $b$ are both real numbers. The real part of the complex number $a+bi$ is $a$, whereas $bi$ is the imaginary part.
The only possible values of the powers of the imaginary unit $i$ are $1,i,-1,$ and $-i$.

Everything you should understand about the structures of imaginary and complex numbers, their equivalence, and how they are used in mathematics has been covered in this article. This is crucial in the study of complex numbers, and the knowledge we grasped from this discussion can be extended to study other mathematical concepts in the study of numbers in the complex system.