- Home
- >
- 6 Times Table – Explanation & Examples
JUMP TO TOPIC
6 Times Table – Explanation & Examples
 The 6 times table is a bit tricky compared to the first five multiplication tables. However, if you have memorized the previous multiplication tables, then you already know the first five multiples of the number 6, which are:
The 6 times table is a bit tricky compared to the first five multiplication tables. However, if you have memorized the previous multiplication tables, then you already know the first five multiples of the number 6, which are:
- $1\times 6 =6 = 6 \times 1$
- $2\times 6 = 12 = 6 \times 2$
- $3\times 6 =18 = 6 \times 3$
- $4 \times 6 =24 = 6 \times 4$
- $5\times 6 = 30 = 6 \times 5$
6 times table is the table which contains multiples of the number 6.
Learning and understanding the 6 times table is essential for solving multiplication, division, and factorization problems. Like the previous tables, this one also follows some patterns that make it easier to understand. This topic will provide interesting tips and skills to help students master it faster.
You should refresh the following concepts to understand the material discussed here.
- Basics of addition and multiplication
- 1 times table
- 2 times table
- 3 times table
- 4 times table
- 5 times table
6 Multiplication Table
We can write the table of 6 as follows:
- $6\times1 = 6$
- $6 \times 2 = 12$
- $6 \times 3 = 18$
- $6 \times 4 =24$
- $6 \times 5 =30$
- $6 \times 6 =36$
- $6 \times 7 = 42$
- $6 \times 8 = 48$
- $6 \times 9 = 54$
- $6 \times 10 = 60$
Different Tips for the 6 Times Table
Let us look at some simple tips which can help us memorize the 6 times table.
Using the 5 Times table: One of the easiest ways for students to learn the 6 times table is by using the 5 times table. This method will help revise the 5 times table and learn the 6 times table simultaneously.
5 Times Table | Addition | (Answer) | 6 Times Table |
$5\times {\color{#cccc00}1} = {\color{green}5}$ | ${\color{green}5} + {\color{#cccc00}1}$ | ${\color{red}6}$ | $ 6 \times 1 = 6$ |
$5\times {\color{#cccc00}2} = {\color{green}10}$ | ${\color{green}10} + {\color{#cccc00}2}$ | ${\color{red}12}$ | $6 \times 2 = 12$ |
$5\times {\color{#cccc00}3} = {\color{green}15}$ | ${\color{green}15} + {\color{#cccc00}3}$ | ${\color{red}18}$ | $6 \times 3 = 18$ |
$5\times {\color{#cccc00}4} = {\color{green}20}$ | ${\color{green}20} + {\color{#cccc00}4}$ | ${\color{red}24}$ | $6 \times 4 =24$ |
$5\times {\color{#cccc00}5} = {\color{green}25}$ | ${\color{green}25} + {\color{#cccc00}5}$ | ${\color{red}30}$ | $6 \times 5 =30$ |
$5\times {\color{#cccc00}6} = {\color{green}30}$ | ${\color{green}30} + {\color{#cccc00}6}$ | ${\color{red}36}$ | $6 \times 6 =36$ |
$5\times {\color{#cccc00}7} = {\color{green}35}$ | ${\color{green}35} + {\color{#cccc00}7}$ | ${\color{red}42}$ | $6 \times 7 = 42$ |
$5\times {\color{#cccc00}8} = {\color{green}40}$ | ${\color{green}40} + {\color{#cccc00}8}$ | ${\color{red}48}$ | $6 \times 8 = 48$ |
$5\times {\color{#cccc00}9} = {\color{green}45}$ | ${\color{green}45} + {\color{#cccc00}9}$ | ${\color{red}54}$ | $6 \times 9 = 54$ |
$5\times {\color{#cccc00}10} = {\color{green}50}$ | ${\color{green}50} + {\color{#cccc00}10}$ | ${\color{red}60}$ | $6 \times 10 = 60$ |
In this method, if we add natural numbers in ascending order to the multiples of the number 5, as shown in the table above, the addition will correspond to the 6 times table. Note that the first multiple of the number 5 is added with the first natural number, which is 1. Similarly, the second multiple of the number 5 is added with the second natural number, 2, and so on.
- Digits pattern 1: The 6 times table follows a specific pattern of 6, 2, 8, 4, and 0. These are the last digits of the first five multiples of the number 6. This pattern continues: the last digits of the next five multiples of 6 are also 6,2,8,4, and 0.

The table above shows the pattern in green digits, and by memorizing this pattern, students can easily learn and memorize the 6 times table.
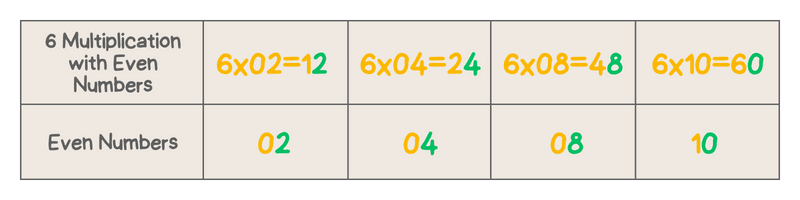
- Digits pattern 2: When the number 6 is multiplied by an even number, the last digit of the resulting outcome will be the same as the even number being multiplied. For example, when we multiply $6$ by $2$, we get $6\times 2 = 12$, so the last digit of the number $12$ is again $2$. Similarly, $6\times 8 = 48$; again, the last digit of $48$ is $8$, which is the even number we multiplied by $6$. The green digits in the picture below depict this pattern for the first four even numbers.
- Using the 3 times table: This method is quite simple and easy to understand. This method will also help students in the revision of the 3 times table. In this method, if all the outcomes of the 3 times table are doubled, i.e., multiplied by $2$, then we get the 6 times table, as shown below.
3 Times Table | Addition | (Answer) |
$3\times 1 = {\color{green}3}$ | ${\color{green}3} + {\color{green}3}$ | ${\color{red}6}$ |
$3\times 2 = {\color{green}6}$ | ${\color{green}6} + {\color{green}6}$ | ${\color{red}12}$ |
$3\times 3 = {\color{green}9}$ | ${\color{green}9} + {\color{green}9}$ | ${\color{red}18}$ |
$3\times 4 = {\color{green}12}$ | ${\color{green}12} + {\color{green}12}$ | ${\color{red}24}$ |
$3\times 5 = {\color{green}15}$ | ${\color{green}15} + {\color{green}15}$ | ${\color{red}30}$ |
$3\times 6 = {\color{green}18}$ | ${\color{green}18} + {\color{green}18}$ | ${\color{red}36}$ |
$3\times 7 = {\color{green}21}$ | ${\color{green}21} + {\color{green}21}$ | ${\color{red}42}$ |
$3\times 8 = {\color{green}24}$ | ${\color{green}24} + {\color{green}24}$ | ${\color{red}48}$ |
$3\times 9 = {\color{green}27}$ | ${\color{green}27} + {\color{green}27}$ | ${\color{red}54}$ |
| $3\times 10 = {\color{green}30}$ | ${\color{green}30} + {\color{green}30}$ | ${\color{red}60}$ |
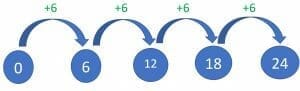
- Addition: Students who have difficulty understanding previous tips and tricks can use the addition method to learn the 6 times tables. It is an effective method, but it is lengthy and calculations may take time.This method is particularly effective if students want to learn the first 10 multiples of 6. As the name suggests, this method involves addition. For example, we start with digit 0, and if we add the number 6 to it, we get 6 as the answer. We can work out the next multiple of 6 by adding 6 to the current answer and so on.
- Recitation: Students who have difficulty grasping previous tips and tricks and find it hard to do the addition or multiplication can use this method. Students can recite the 6 times loudly and repeatedly. Once they have memorized it, it will be easier for them to develop their basic understanding. Recitation can be done like:6 times 1 is 6
6 times 2 is 12
6 times 3 is 18
6 Times 4 is 24
6 times 5 is 30
6 times 6 is 36
6 times 7 is 42
6 times 8 is 48
6 times 9 is 54
6 times 10 is 60
Table of 6 From 1 to 20:
We can write a complete table of 6 from 1 to 20 as:
Numerical Representation | Descriptive Representation | Product (Answer) |
$6 \times 1$ | Six times one | $6$ |
$6 \times 2$ | Six times two | $12$ |
$6 \times 3$ | Six times three | $18$ |
| $6 \times 4$ | Six times four | $24$ |
$6 \times 5$ | Six times five | $30$ |
$6 \times 6$ | Six times six | $36$ |
$6 \times 7$ | Six times seven | $42$ |
$6 \times 8$ | Six times eight | $48$ |
$6 \times 9$ | Six times nine | $54$ |
$6\times 10$ | Six times ten | $60$ |
$6\times 11$ | Six times eleven | $66$ |
$6\times 12$ | Six times twelve | $72$ |
$6 \times 13$ | Six times thirteen | $78$ |
$6 \times 14$ | Six times fourteen | $84$ |
$6 \times 15$ | Six times fifteen | $90$ |
$6 \times 16$ | Six times sixteen | $96$ |
$6 \times 17$ | Six times seventeen | $102$ |
$6 \times 18$ | Six times eighteen | $108$ |
$6 \times 19$ | Six times nineteen | $114$ |
$6 \times 20$ | Six times twenty | $120$ |
Example 1: Using the 6 times table, calculate 2 times 6 times 1 minus 4
Solution:
2 times 6 times 1 minus 4 can be written as:
$ 2 \times6 \times 1 – 4$
$ = 12 \times 1 – 4$
$ = 12 – 4$
$ = 8$
Example 2: What is the 9th multiple of 6?
Solution:
We know the first 10 multiples of 6 are 6, 12, 18, 24, 30, 36, 42, 48, 54, and 60.
So the 9th multiple is 54.
Practice Questions:
1). A teacher wants to distribute pens equally among the students of her class. How many pens should she give to each student if the class consists of 6 students and
- the total number of pens equals 18?
- the total number of pens is equal to 36?
2). Calculate 6 times 6 minus 30.
3). Find the value of “Y” if “Y x 6 = 36”.
4). From the given table, select the numbers which are multiples of 6.
| 24 | 21 | 36 | 40 | 45 |
| 18 | 19 | 22 | 12 | 10 |
| 19 | 11 | 13 | 17 | 15 |
| 30 | 37 | 15 | 16 | 29 |
| 31 | 63 | 10 | 25 | 21 |
| 32 | 14 | 15 | 29 | 80 |
| 46 | 32 | 71 | 74 | 65 |
| 37 | 37 | 54 | 72 | 71 |
| 41 | 42 | 72 | 51 | 65 |
| 44 | 48 | 59 | 49 | 60 |
Answer Key
1). The teacher wants to distribute the pens equally. So each student should have the same number of pens. As the class has 6 students, so we can use 6 times table to solve this problem
- When the total number of pens is 18, we know by 6 times table that $6\times 3 =18$, so each student would get 3 pens.
- When the total number of pens is 36, we know by 6 times table that $6\times 6 =36$, so each student would get 6 pens.
2). 6 times 6 minus 30 can be written as:
$ 6\times 6 – 30$
$ = 36 – 30$
$ = 6$
3). $ Y \times 6 = 36 $
We know $6\times 6 =36$
$ Y = 6 $
4)
| 24 | 21 | 36 | 40 | 45 |
| 18 | 19 | 22 | 12 | 10 |
| 19 | 11 | 13 | 17 | 15 |
| 30 | 37 | 15 | 16 | 29 |
| 31 | 63 | 10 | 25 | 21 |
| 32 | 14 | 15 | 29 | 80 |
| 46 | 32 | 71 | 74 | 65 |
| 37 | 77 | 54 | 72 | 71 |
| 41 | 42 | 72 | 51 | 65 |
| 44 | 48 | 59 | 49 | 60 |
