• Home
  • >
  • Equivalent Fractions – Explanation & Examples

Equivalent Fractions – Explanation & Examples

Equivalent FractionsIn mathematics, equivalent fractions are simply fractions with different numerators and denominators but represent the same proportion of a whole. Equivalent fractions seem to be different at a look, but they have similar or equal value.

For example, the equivalent fractions for 1/4 are:

2/8, 3/12, 4/16, etc.

The equivalent fractions have an equal amount or value after simplification of both their numerator and denominators. The fractions will generate the same value if cancellation by a common factor is made on both the numerator and denominator.

What are Equivalent Fractions?

Equivalent fractions are two or more fractions that result in the same value after simplification. Suppose a/b and c/d are two fractions, then the fractions are equivalent only if the simplification of each fraction results in e/f.

In other words,

a/b = c/d = e/f.

For example, a fraction 1/3 has an equivalent of 5/15 because of simplification of 5/15 results in the same value as 1/3.

Now the question arises for why these fractions are equal despite having different numbers. The answer to this query is that the fractions contain numerators and denominators that are not co-prime numbers. Therefore they have a common multiple which on division produces the same value.

Let’s take an example:

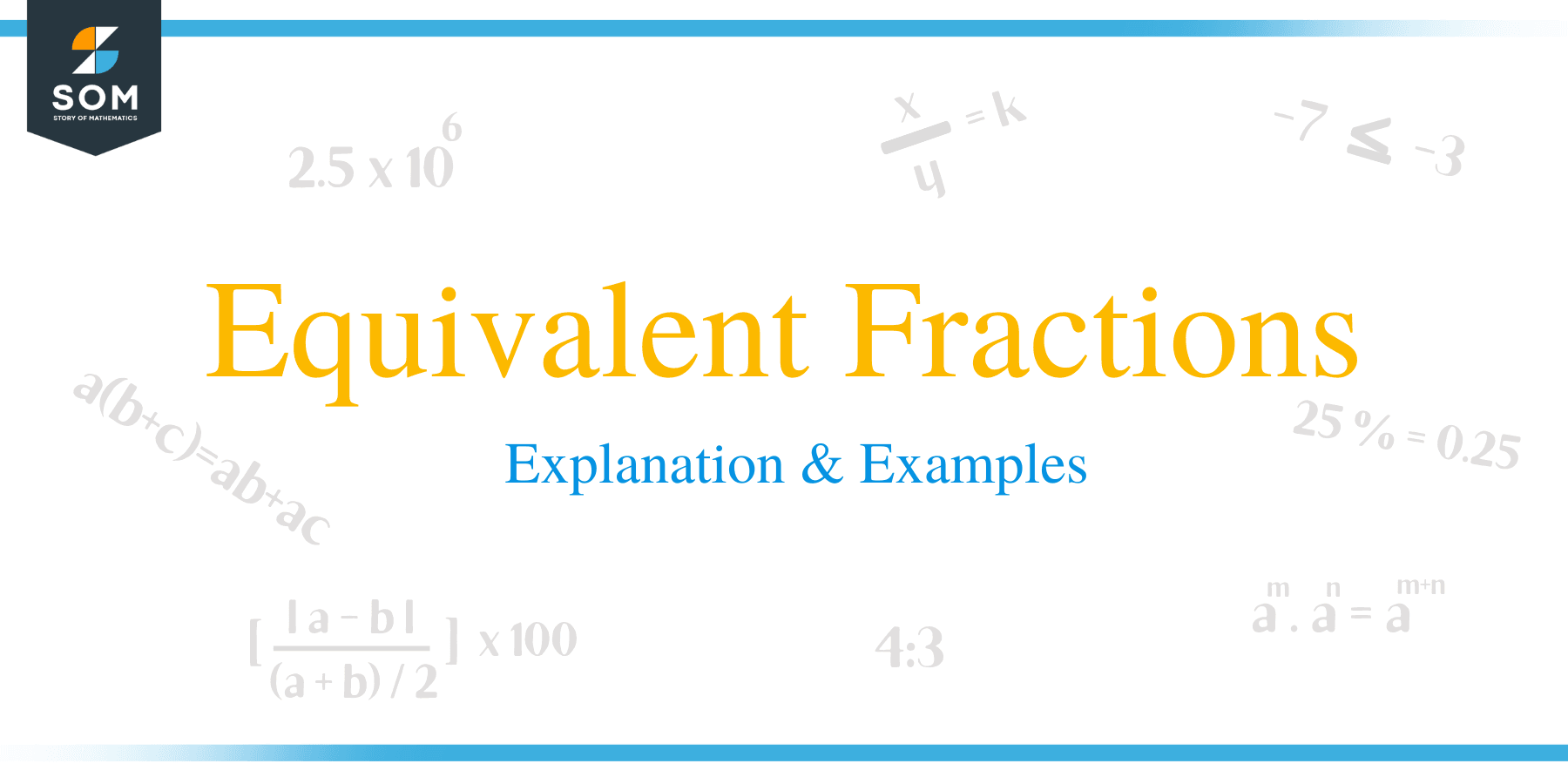
1/2 = 2/4 = 4/8

You can notice that yet the above two factions have different integers, but after dividing both the numerator and denominator by a common factor, the result is:

(4 ÷ 4)/(8 ÷ 4)

=1/2

In this case, if we simplify 2/4, the result 1/2 again.

(2 ÷ 2)/(4 ÷ 2)

= 1/2What are Equivalent Fractions?

It has been shown that either dividing the denominator or multiplying the numerator with the same factor does not alter the value of the fraction. And therefore, equivalent fractions have an equal value when simplified.

How do you find equivalent fractions?

Consider a case with the fraction 1/5.

Multiplying both the numerator and denominator with 2, 3 and 4 gives:

1/5 x 2/2 = 2/10

1/5 x 3/3 = 3/15

1/5 x 4/4 = 4/20

Therefore, it can be concluded that:

1/5 = 2/10 = 3/15 = 4/20

The equivalent fraction can only be generated by multiplication or division by a common factor. Carrying out addition or subtraction on the fraction only changes the value of a fraction.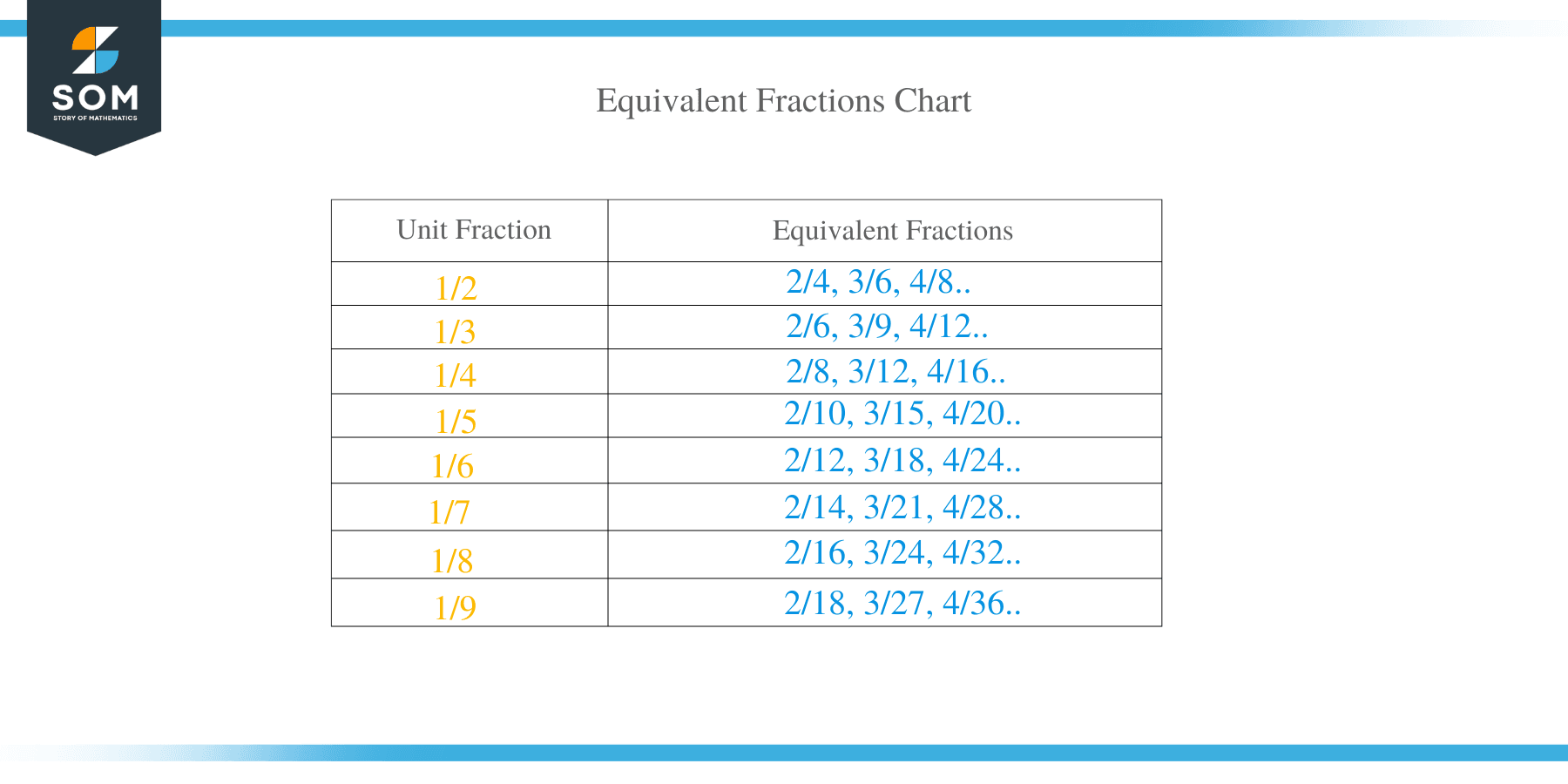How do you find equivalent fractions?

Example 1:

Given that the fractions 5/16 and x/12 are equivalent calculate the value of x.

Solution

Given that:

5/16 = x/12

x = (5 x 12)/16

x = 60/16

x =15/4

And thus, the value of x is 15/4.

Example 2:

Find the value of x if the fractions 3/5 and 4/x are equivalent.

Solution

Given that,

3/5 = 4/x

x = (4 x 5)/3

x = 20/3

 

Practice Questions

1. Which of the following is not equivalent to $\dfrac{3}{4}$?

2. Which of the following is not equivalent to $\dfrac{4}{5}$?

3. Which of the following is not equivalent to $\dfrac{6}{7}$?

4. Which of the following is not equivalent to $\dfrac{2}{9}$?

5. What is the equivalent fraction of $\dfrac{1}{2}$ so that it has a denominator of $12$?

6. What is the equivalent fraction of $\dfrac{1}{3}$ so that it has a denominator of $18$?

7. What is the equivalent fraction of $\dfrac{3}{4}$ so that it has a denominator of $20$?

8. What is the equivalent fraction of $\dfrac{5}{6}$ so that it has a denominator of $30$?

9. What is the equivalent fraction of $\dfrac{6}{12}$ so that it has a numerator of $24$?

10. What is the equivalent fraction of $\dfrac{3}{8}$ so that it has a numerator of $24$?

11. What is the equivalent fraction of $\dfrac{2}{6}$ so that it has a numerator of $48$?

12. What is the equivalent fraction of $\dfrac{4}{6}$ so that it has a numerator of $12$?

13. Which of the following pairs of fractions are not equivalent fractions?

14. I’m thinking of an equivalent fraction to $\dfrac{10}{15}$ with $2$ as the numerator. What fraction am I thinking of?

15. Erick notices that either $\dfrac{3}{5}$ or $\dfrac{3}{4}$ is equal to the fraction $\dfrac{12}{20}$. Which fraction is equal to $\dfrac{12}{20}$?

16. Peter gave $\dfrac{1}{4}$ and $\dfrac{3}{12}$ of orange to Donald and Pedro, respectively. Did he give out an equivalent fraction of an orange?


 

Previous Lesson | Main Page | Next Lesson