This problem aims to familiarize us with different methods to solve a differential. The concept required to cater to this problem mostly relates to ordinary differential equations. We define an ordinary differential equation or most commonly known as ODE, as an equation that has one or additional functions of a single independent variable given with their derivatives. On the other hand, an equation that includes a function more than a single derivative is known as a differential equation. But as we speak of ODE, the term ordinary is employed for the derivative of one independent variable.
The rules that are going to use in this problem are the product rule, quotient rule, and chain rule.
Whenever a function contains another function within it, we differentiate that function with the help of the chain rule. It is given as:
\[ f(g(x)) \]
The derivative can then be taken as:
\[ \dfrac{d}{dx}(f(g(x)) = f'(g(x))\cdot g'(x) \]
\[ \dfrac{dy}{dx} = \dfrac{dy}{du}\cdot \dfrac{du}{dx} \]
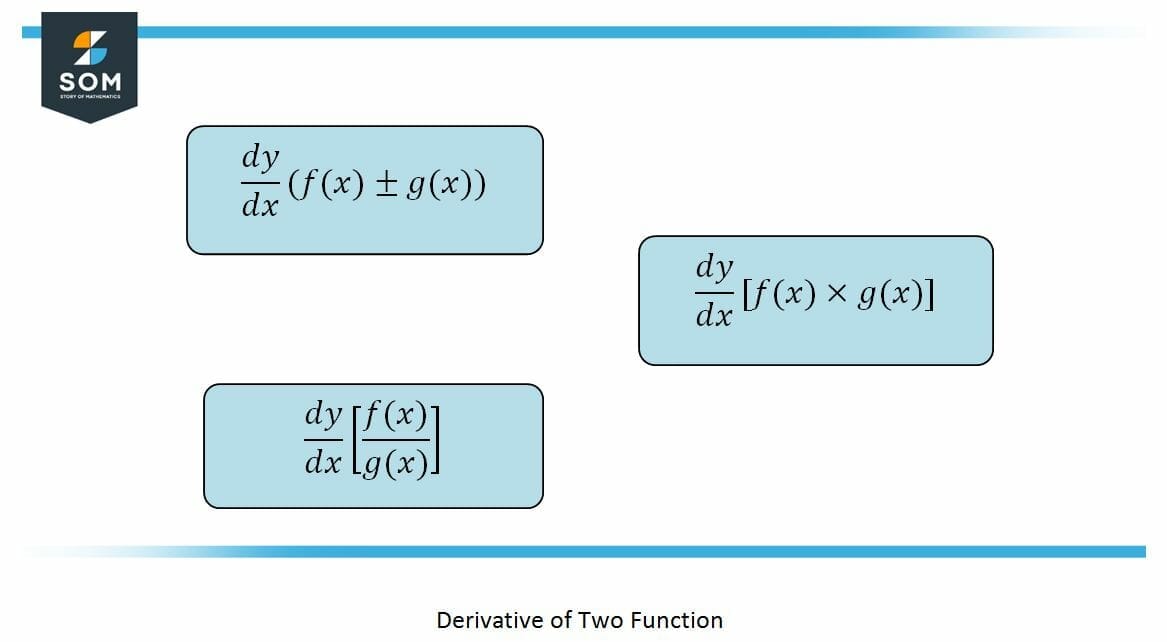The product rule as it says is the derivative of two functions that are arithmetically being multiplied, given as:
\[ \dfrac{d}{dx}(f \cdot g) = f\cdot \dfrac{dg}{dx} + g\cdot \dfrac{df}{dx} \]
Whereas the quotient rule applies to the functions that are in the form of a fraction, given as:
\[ \dfrac{d}{dx} \{\dfrac{f(x)}{g(x)}\} = \dfrac{g\cdot \dfrac{df}{dx} – f\cdot \dfrac{dg}{dx}}{g^2}\]
Expert Answer
We are given the following information:
\[ f(5) = 1,\space f'(5) = 6\]
\[ g(5) = -3,\space g'(5) = 2\]
First, we are going to find $(f(x)\cdot g(x))$ using the product rule:
\[ \dfrac{d}{dx}(f\cdot g) = f\dfrac{dg}{dx} + g\dfrac{df}{dx} \]
\[ \dfrac{d}{dx}(f(5)g(5)) = f(5)g'(5) + g(5)f'(5) \]
\[ \dfrac{d}{dx}(f(5)g(5)) = 1\times 2 + (-3)\times 6 \]
\[ \dfrac{d}{dx}(f(5)g(5)) = -16 \]
Next, we are going to find $(\dfrac{f(x)}{g(x)})’$ using the quotient rule:
\[ \dfrac{d}{dx} \{\dfrac{f(5)}{g(5)}\} = \dfrac{g(5)f'(5) – f(5)g'(5)}{g(5)^2} \]
\[ (\dfrac{f(5)}{g(5)})’ = \dfrac{(-3)\times 6 – 1\times 2}{(-3)^2} \]
\[ (\dfrac{f(5)}{g(5)})’ = \dfrac{-18 – 2}{9} \]
\[ (\dfrac{f(5)}{g(5)})’ = \dfrac{-20}{9} \]
And finally, we are going to find $(\dfrac{g(x)}{f(x)})’$ using the quotient rule:
\[ \dfrac{d}{dx} \{\dfrac{g(5)}{f(5)}\} = \dfrac{f(5)g'(5) – g(5)f'(5)}{f(5)^2} \]
\[ (\dfrac{g(5)}{f(5)})’ = \dfrac{1\times 2 – (-3)\times 6}{1^2} \]
\[ (\dfrac{g(5)}{f(5)})’ = \dfrac{2 + 20}{1} \]
\[ (\dfrac{g(5)}{f(5)})’ = 20 \]
Numerical Result
Part a: $\dfrac{d}{dx}(f(5)g(5)) = -16$
Part b: $(\dfrac{f(5)}{g(5)})’ = \dfrac{-20}{9}$
Part c: $(\dfrac{g(5)}{f(5)})’ = 20$
Example
Given that $f(3)=1$, $f'(3)=8$, $g(3)=-6$, and $g'(3)=2$. Find the following differentials, $(fg)'(3)$, $(f/g)'(3)$ and $(g/f)'(3)$.
According to the statement, we are given:
\[ f(3) = 1,\space f'(3) = 8\]
\[ g(3) = -6,\space g'(3) = 2\]
First, finding $(f(x)\cdot g(x))$:
\[ \dfrac{d}{dx}(f\cdot g) = f\dfrac{dg}{dx} + g\dfrac{df}{dx}\]
\[ \dfrac{d}{dx}(f(3)g(3)) = f(3)g'(3) + g(3)f'(3) \]
\[ (f(3)g(3))’ = 1\times 2 + (-6)\times 8 \]
\[ (f(3)g(3))’ = -46 \]
Next, finding $(\dfrac{f(x)}{g(x)})’$:
\[ \dfrac{d}{dx} \{\dfrac{f(3)}{g(3)}\} = \dfrac{g(3)f'(3) – f(3)g'(3)}{g(3)^2} \]
\[ (\dfrac{f(3)}{g(3)})’ = \dfrac{(-6)\times 8 – 1\times 2}{(-6)^2} \]
\[ (\dfrac{f(3)}{g(3)})’ = \dfrac{-48 – 2}{36} \]
\[ (\dfrac{f(3)}{g(3)})’ = \dfrac{-25}{18} \]
And finally, $(\dfrac{g(x)}{f(x)})’$:
\[ \dfrac{d}{dx} \{\dfrac{g(3)}{f(3)}\} = \dfrac{f(3)g'(3) – g(3)f'(3)}{f(3)^2} \]
\[ (\dfrac{g(3)}{f(3)})’ = \dfrac{1\times 2 – (-6)\times 8}{1^2} \]
\[ (\dfrac{g(5)}{f(5)})’ = \dfrac{2 + 48}{1} \]
\[ (\dfrac{g(5)}{f(5)})’ = 50 \]