- Home
- >
- Zero Exponents – Explanation & Examples
Zero Exponents – Explanation & Examples
 An exponential number is a function that is expressed in the form x ª, where x represents a constant, known as the base, and ‘a’, the exponent of this function, and can be any number.
An exponential number is a function that is expressed in the form x ª, where x represents a constant, known as the base, and ‘a’, the exponent of this function, and can be any number.
The exponent is hitched onto the upper right shoulder of the base. It defines the number of times the base is multiplied by itself. For example, 4 3 represents an operation; 4 x 4 x 4 = 64. On the other hand, a fractional power represents the root of the base, for example, (81)1/2 give 9.
Zero Exponent Rule
Considering several ways in which we can define an exponential number, we can derive the zero-exponent rule by considering the following:
- x 2/x 2 = 1. Considering the division rule, when we divide numbers with same base, we subtract the exponents.
x2/x 2 = x 2 – 2 = x 0 but we already know that x2/x2 = 1; therefore x 0= 1
Hence, we can conclude that any number, except zero raised to the zero power is 1.
- Verification of the zero-exponent rule
Let the number 8 0 be an exponential term. In this case 8 is the base and zero is the exponent.
But since we know that multiplication of one and any exponential number is equivalent to the exponential number itself.
⟹⟹ 8 0 = 1× 8 0 = 1×1
Now, we write the number 1 and the base number 8 zero times.
⟹⟹ 8 0 = 1
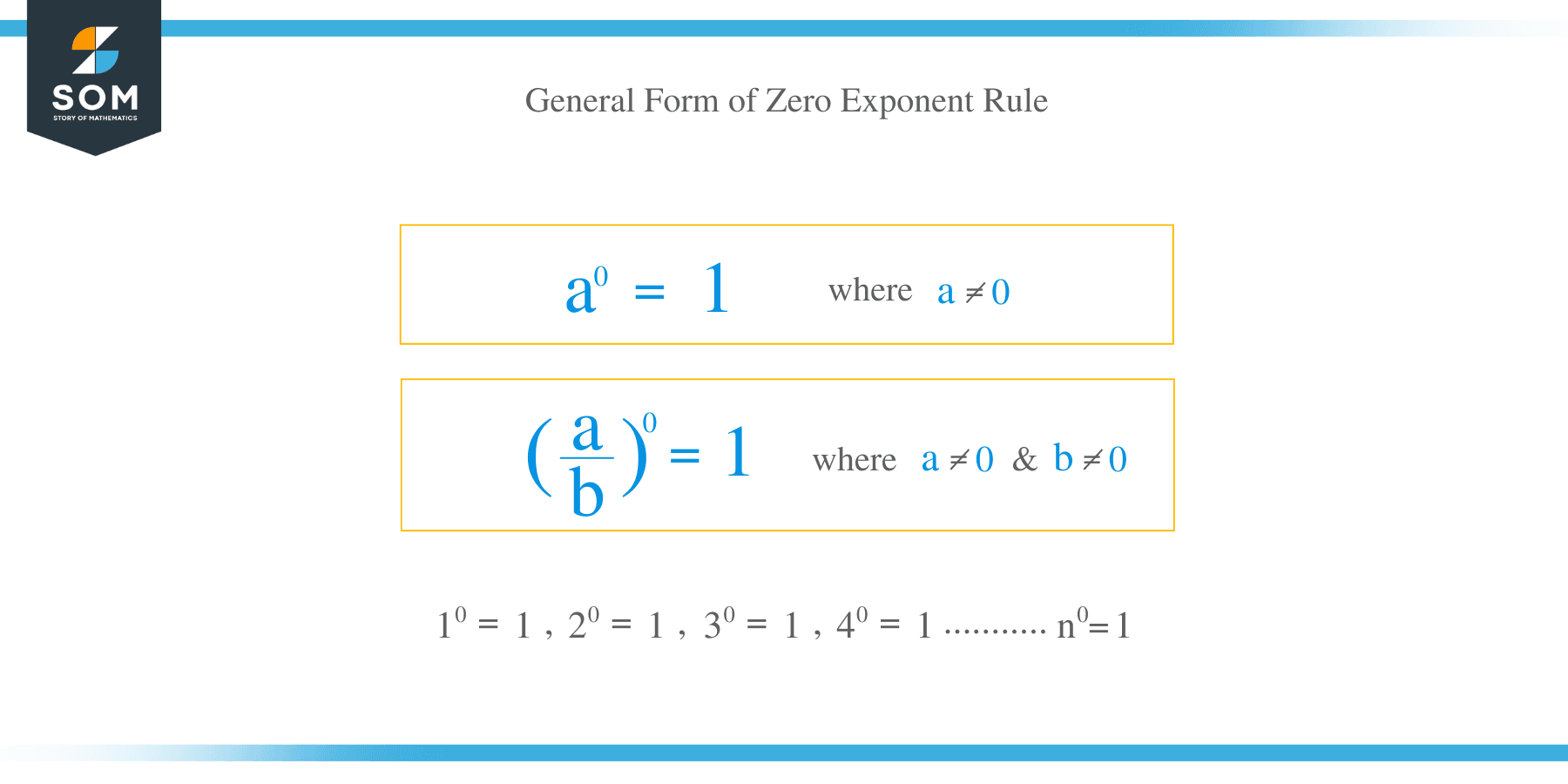
Therefore, it is proven that any number or expression raised to the power of zero is always equal to 1. In other words, if the exponent is zero then the result is 1. The general form of zero exponent rule is given by: a 0 = 1 and (a/b) 0 = 1.
Example 1
(-3) 0 = 1
(2/3) 0 = 1
0° = undefined. This is similar to dividing a number by zero.
Therefore, we can write the rule as a° =1. Alternatively, the zero-exponent rule can be proved by considering following cases.
Example 2
31 = 3 = 3
32 = 3*3 = 9
33 = 3*3*3 = 27
34 = 3*3*3*3 = 81
And so on.
You can note that, 33= (34)/3, 32 = (33)/3, 31= (32)/3
3(n-1) = (3n)/3
So 30= (31)/3=3/3=1
This formula will work for any number but not the number 0.
Now let’s generalize the formula by calling any number x:
x(n-1) =x n/x
So x0 = x (1-1) = x1/x = x/x = 1
And hence proven.
Example 3
Consider another case of:
52 * 54 = 5(2+4) = 56 = 15625
In this formula, change one of the exponents to negative:
52 * 5-4 = 5(2-4) = 5-2 = 0.04
What if the exponents are have the same magnitude:
52 * 5-2 = 5(2-2) = 50
Recall that, a negative exponent means, one divided by the number to the exponent:
5-2 = 1/52 = 0.04
And so write, 52 * 5-2 in a different way:
52 * 5-2 = 52 * 1/52 = 52/52 = 25/25
Since any number divided by itself is always 1 therefore;
52 * 5-2 = 52 * 1/52 = 52/52 = 25/25 = 1
52*5-2 = 5(2-2) = 50
52 * 5-2 = 52/52 = 1
This implies that 50 = 1. Hence the zero-exponent rule is proven.
Example 4
Consider another case:
x a * x b = x (a + b)
If we change one of the exponents to a negative: x a * x-b = x(a-b)
And if the exponents have equal magnitudes, x a * x-b = x a * x-a = x(a-a) = x0
Now recall, a negative exponent implies that one is divided by the number to the exponent:
x-a = 1/x a
Rewrite x a * x-a in a different way:
x a * x-a = x a * 1/x a = x a/x a
And since a number divided by itself is always 1 so:
x a * x-a = x a * 1/x a = x a/x a = 1:
x a * x-a = x(a-a) = x0
and
x a * x-a = x a * 1/x a:
This implies that any number x0 = 1. Hence the zero-exponent rule is proven.
