Cosine – Explanation & Examples

When we deal with right-angled triangles in trigonometry, we can define the cosine function or any other trigonometric function by simply determining the ratio of one side to the other. We only need to check the appropriate labeling of the sides (opposite, adjacent, and the hypotenuse) of the right triangle from the perspective of the reference angle we are using. So, how can we determine the cosine function from a right-angled triangle?

The cosine function is defined by determining the ratio of the length of the side right next to a reference angle (acute angle) to the length of the hypotenuse of a right triangle.

After studying this lesson, we are expected to learn the concepts driven by these questions and be qualified to address accurate, specific, and consistent answers to these questions.

• What is a cosine function?
• How can we determine the formula for cosine function from a right-angled triangle?
• How can we solve actual problems using trigonometric functions?

This lesson aims to clear up any confusion you might have about the concepts involving the cosine function.

What is cosine?

In the context of a right triangle, the cosine function is the ratio of the adjacent side to the hypotenuse. For an angle $\alpha$, the cosine function is denoted by $\sin \alpha$. In other words, cosine is a trigonometric function of any given angle.

The following figure 4-1 represents a typical right triangle. The lengths of the three legs (sides) of the right triangle are named $a$, $b$, and $c$. The angles opposite the legs of lengths $a$, $b$, and $c$ are named $\alpha$, $\beta$, and $\gamma$. The tiny square with the angle $\gamma$ shows that it is a right angle.

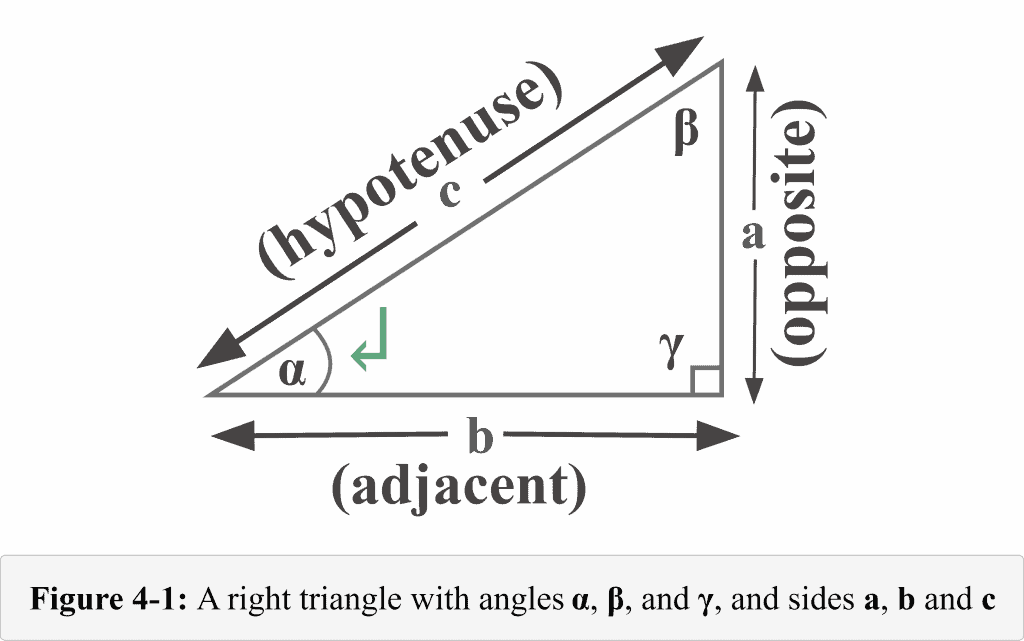
Use the diagram in Figure 4-1 to determine the cosine function from the perspective of the angle α.

Looking at Figure 4-1, we can determine the cosine function from the right-angled triangle if we divide the length of the adjacent to a reference angle α (acute angle) by the length of the hypotenuse.

The following figure 4-2 represents a cosine function.

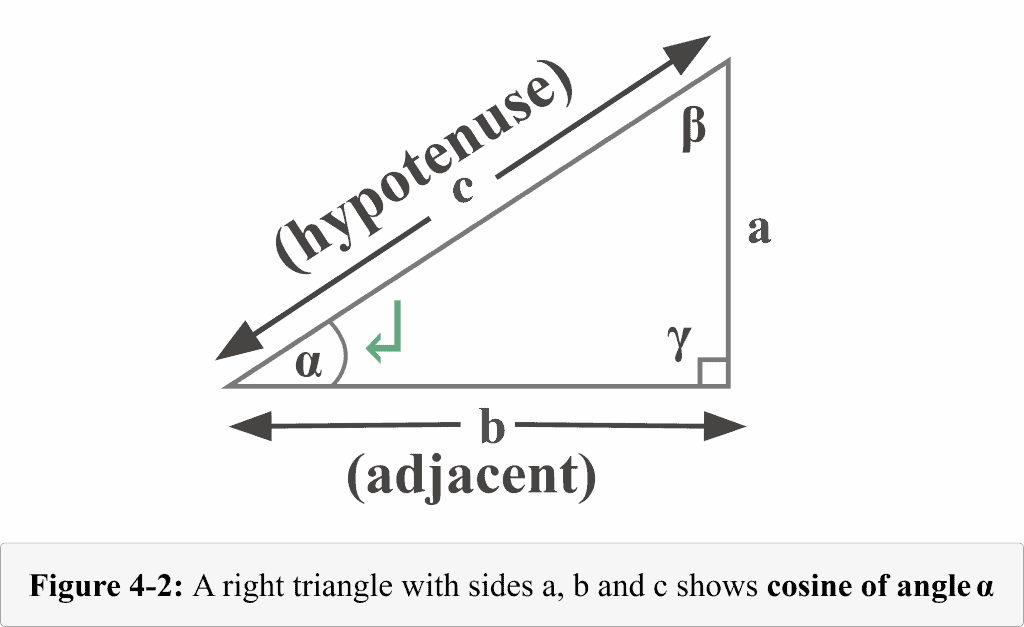
Looking at Figure 4-2, we can identify that the side of length $b$ is the adjacent side that lies right next tothe reference angle $\alpha$, and the side of length $c$ is the hypotenuse. Thus,

Adjacent = $b$

Hypotenuse = $c$

Therefore, the cosine of an angle $\alpha$ is

${\displaystyle \cos \alpha ={\frac {b}{c}}}$

Therefore, we conclude that the cosine function is the ratio of the adjacent side to the hypotenuse.

Cosine function from the perspective of the angle $\beta$

We should be cautious when we apply the terms opposite and adjacent because the meaning of these terms is dependent on the reference angle we are using.

The following figure 4-3 represents a typical right triangle from the perspective of the angle $\beta$.

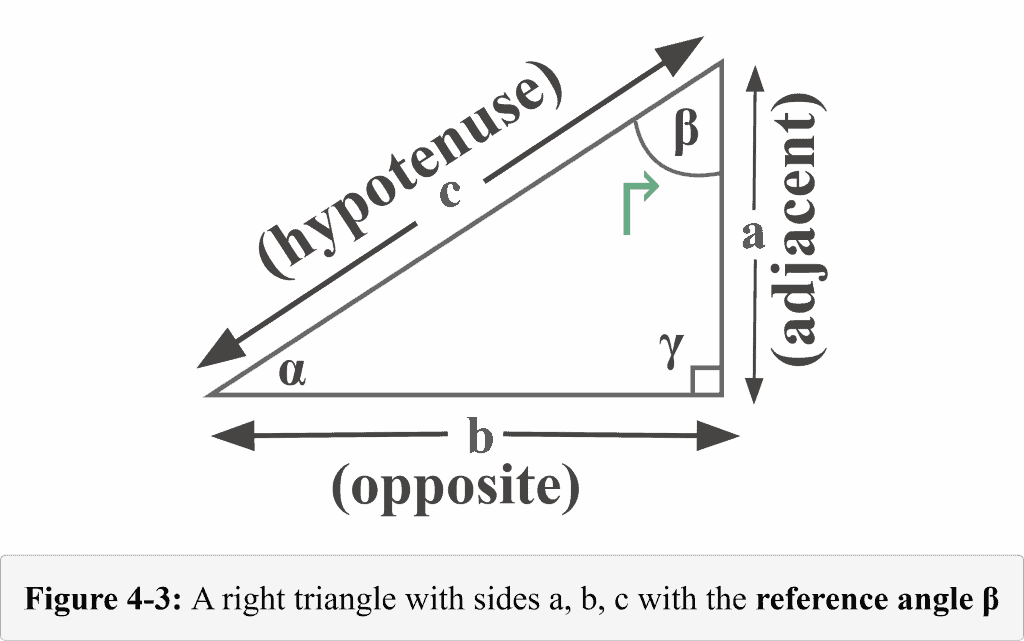
You can observe that now the roles of the sides have been shifted.

Looking at Figure 4-3, it is clear now the length of the side $a$ is right next to the reference angle $\beta$, and the length of the side $b$  lies exactly opposite the reference angle $\beta$. Thus, in relation to the angle measuring $\beta$, now we have

Adjacent = $a$

Opposite = $b$

While the hypotenuse $c$ remains the same. This is why the hypotenuse is very special in a right-angled triangle.

The following figure 4-4 represents a cosine function from the perspective of the angle $\beta$.

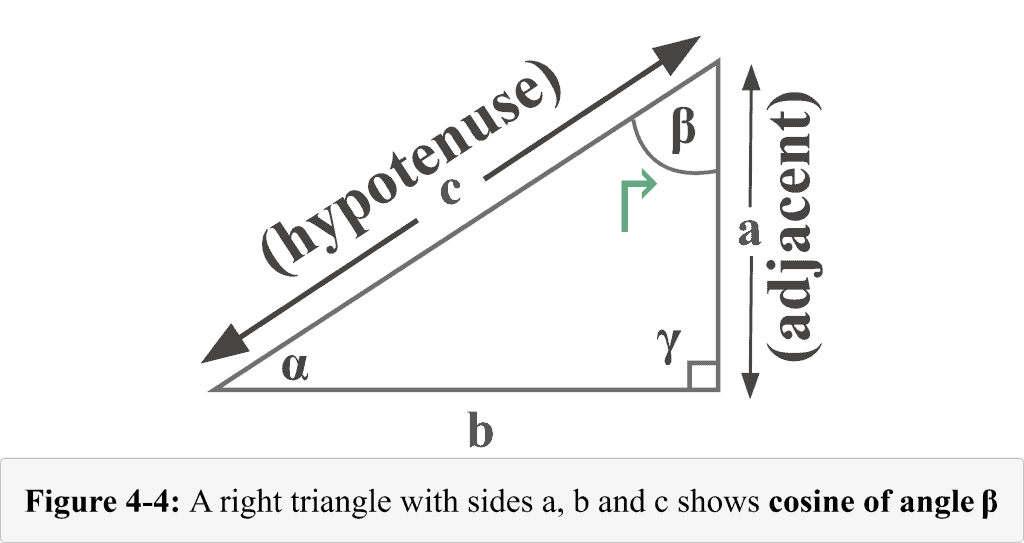
Looking at Figure 4-4, we can identify that the side of length $a$ is the adjacent side that lies right next to the reference angle $\beta$, and the side of length $c$ is the hypotenuse. Thus,

Adjacent = $a$

Hypotenuse = $c$

We know that the cosine function is the ratio of the adjacent side to the hypotenuse.

Therefore, the cosine of an angle $\beta$ is

${\displaystyle \cos \beta ={\frac {a}{c}}}$

What is the formula for cosine?

The following figure 4-5 illustrates a clear comparison of how we determined the ratios of cosine function from the perspective of both the angles$\alpha$ and $\beta$.

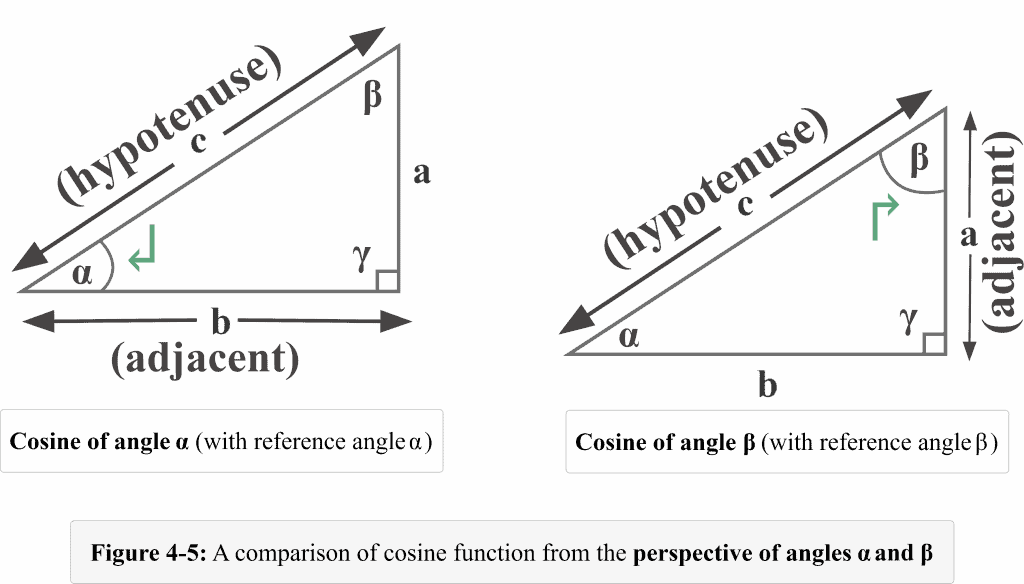
The comparison clearly indicates that the cosine function is the ratio obtained when we divide the length of the side adjacent to a reference angle by the hypotenuse’s length.

Here:

${\displaystyle \cos \alpha ={\frac {b}{c}}}$

Thus,

Similarly,

${\displaystyle \cos \beta ={\frac {a}{c}}}$

Thus,

Therefore, we conclude that the cosine function is the ratio of the adjacent side to the hypotenuse.

How to remember the formula of the cosine function?

We created the SOH – CAH – TOA table in previous lessons to remember the formulae of the trigonometric functions. You must memorize the second portion — CAH — of the code-word SOH – CAH – TOA to remember the formula of the cosine function.

Here is the table:

                                                   ↑

                                   YOU ARE HERE!

Example $1$

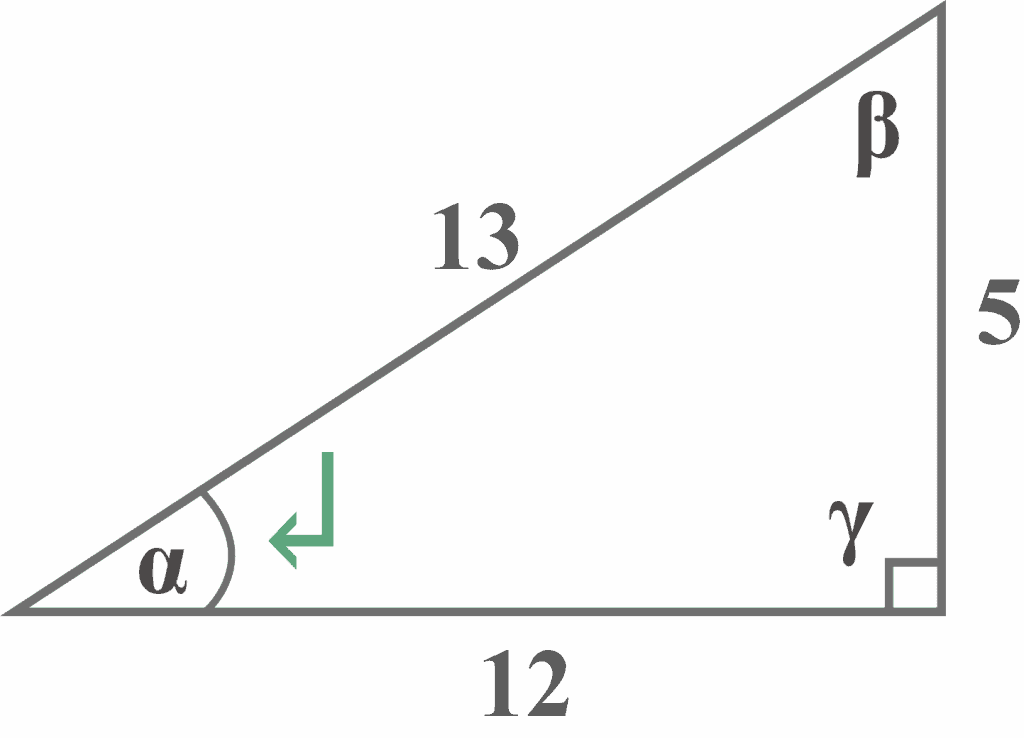
Given a right-angled triangle with the reference angle $\alpha$. What is the cosine of angle $\alpha$?

Solution:

Looking at the diagram, it is clear that the side of length $12$ is the adjacent side that lies right next to the reference angle $\alpha$, and the side of length $13$ is the hypotenuse. Thus,

Adjacent = $12$

Hypotenuse = $13$

We know that the cosine function is the ratio of the adjacent side to the hypotenuse.

${\displaystyle \cos \alpha ={\frac {\mathrm {adjacent} }{\mathrm {hypotenuse} }}}$

Therefore, the cosine of angle $\alpha$ is:

Example 2

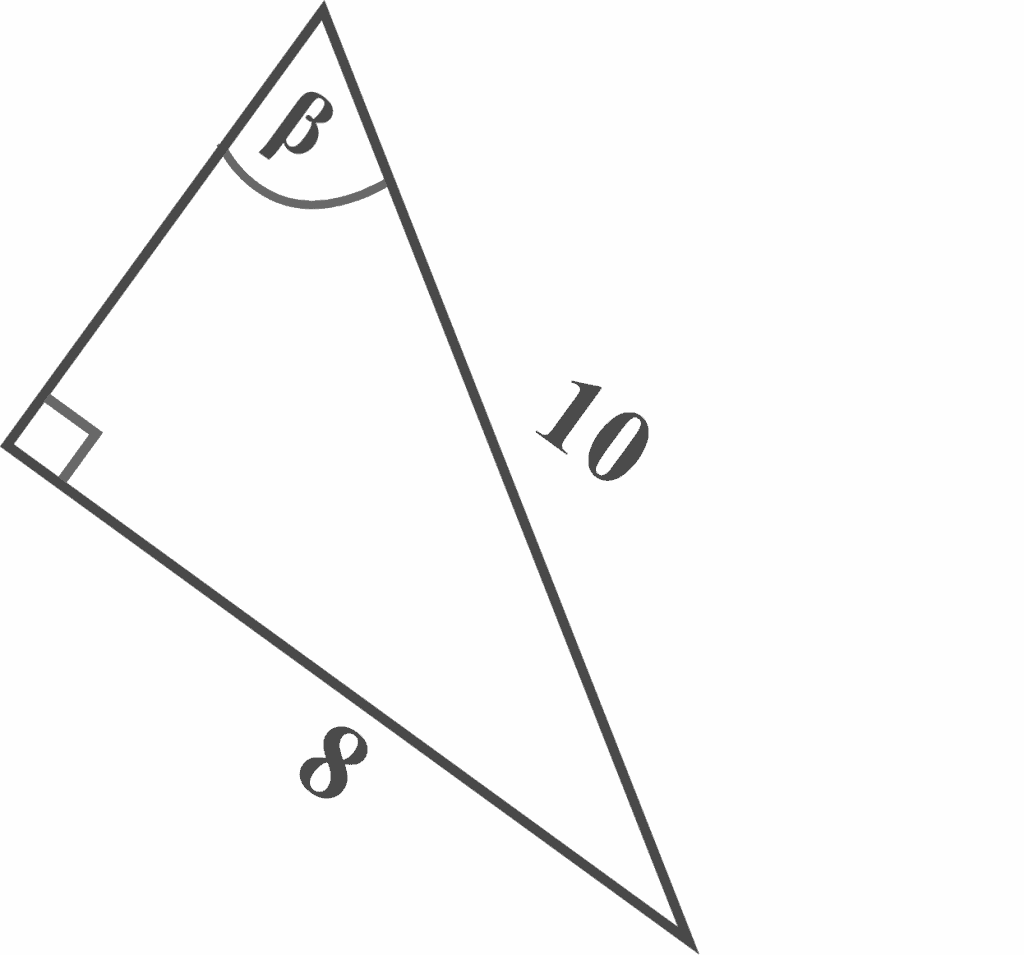
Given a right-angled triangle with the reference angle $\beta$. What is the cosine of angle $\beta$?

Solution:

Looking at the diagram, it is clear that the side of length $8$ is the opposite side that lies exactly opposite the reference angle $\beta$. Also, the length of the side $10$ is the hypotenuse.

We have to determine the cosine of angle $\beta$. All right, here comes the tricky part.

We know that the cosine function is the ratio of the adjacent side to the hypotenuse, but the adjacent side’s length is missing. What should we do?

Step 1: Determine the unknown but relevant side — the adjacent.

 To determine the adjacent side, we need to use the Pythagoras theorem,

$c^{2}=a^{2}+b^{2}$

looking at the diagram, we have:

Opposite $b = 8$

Hypotenuse $c = 10$

Adjacent $a =$?

Substitute $b = 8$ and $c = 10$ in the formula

$10^{2}=a^{2}+8^{2}$

$100=a^{2}+64$

$a^{2}=36$

$a = 6$ units

Thus, the length of the adjacent is $6$ units.

Step 2: Determine the cosine of angle $\beta$.

Now, we have:

Adjacent $= 6$

Hypotenuse $= 10$

Using the formula of the cosine function

${\displaystyle \cos \beta ={\frac {\mathrm {adjacent} }{\mathrm {hypotenuse} }}}$

Therefore, the cosine of angle $\beta$ is:

${\displaystyle \cos \beta ={\frac {6}{10}}}$

Example 3

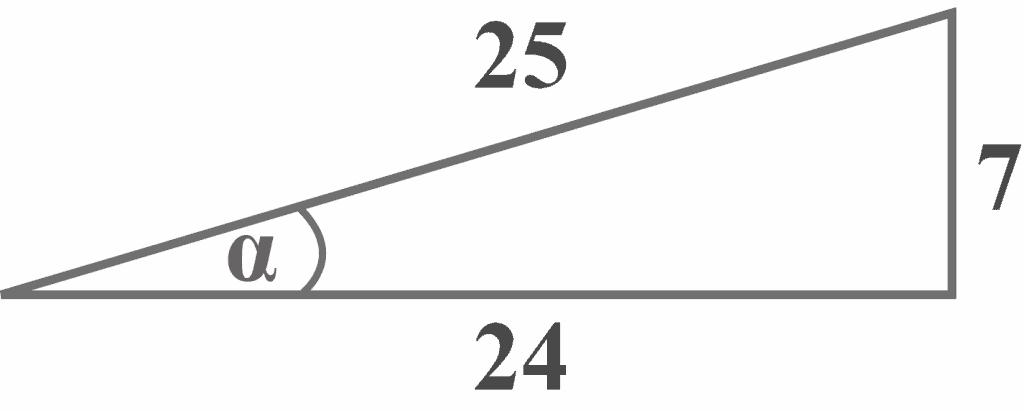
Given a right-angled triangle with the reference angle $\alpha$. Which option represents the trigonometric ratio of ${\frac {24}{25}}$?

       a) $\sin \alpha$

       b) $\cos \alpha$

       c) $\tan \alpha$

       d) $\cot \alpha$

Solution:

Looking at the diagram, it is clear that the side of length $24$ is the adjacent side that lies right next to the reference angle $\alpha$, and the side of length $25$ is the hypotenuse.

Thus,

Adjacent = $24$

Hypotenuse = $25$

We know that formula of the cosine function is

${\displaystyle \cos \alpha ={\frac {\mathrm {adjacent} }{\mathrm {hypotenuse} }}}$

Thus,

Therefore, option b) is the true choice.

Example 4

A right-angled triangle is shown in the figure. Determine the length of the adjacent to angle $\beta$? Please note that you must have to use the cosine of angle $\beta$ to determine the answer.

Solution:

We have to determine the length of the adjacent to angle $\beta$, so we need to find the measure of angle β.

Looking at the diagram, we have:

$\alpha = 30^{\circ }$

$\gamma = 90^{\circ }$

We know that the sum of angles in a triangle is $180^{\circ }$.

$\alpha + \beta + \gamma = 180^{\circ }$

substitute $\alpha = 30^{\circ }$, $\gamma = 90^{\circ }$ in the formula

$30^{\circ } + \beta + 90^{\circ } = 180^{\circ }$

$120^{\circ } + \beta = 180^{\circ }$

$\beta = 180^{\circ } – 120^{\circ }$

$\beta = 60^{\circ }$

Also, the adjacent side is missing. Let the length of the adjacent to the reference angle $\beta$ be $x$.

Now, we have:

Adjacent to the reference angle $\beta = x$

Hypotenuse = $8$

$\beta = 60^{\circ }$

We know that formula of the cosine function is

${\displaystyle \cos \beta ={\frac {\mathrm {adjacent} }{\mathrm {hypotenuse} }}}$

substitute adjacent = $x$, hypotenuse = $8$, and $\beta = 60^{\circ }$

${\displaystyle \cos 60^{\circ } ={\frac {x}{8}}}$

As

$\cos 60^{\circ } = 0.5$ (check using a calculator)

Thus,

$0.5 = {\frac {x}{8}}$

$x = (0.5)(8)$

$x = 4$ units

Therefore, the length of the adjacent to the angle β is 4 units.