- Home
- >
- Factor by Grouping – Methods & Examples
JUMP TO TOPIC
Factor by Grouping – Methods & Examples
 Now that you have learned how to factor polynomials by using different methods such as; Greatest Common Factor (GCF, Sum or difference in two cubes; Difference in two squares method; and Trinomial method.
Now that you have learned how to factor polynomials by using different methods such as; Greatest Common Factor (GCF, Sum or difference in two cubes; Difference in two squares method; and Trinomial method.
Which method do you find simplest among these?
All these methods of factoring polynomials are as easy as ABC, only if they are applied correctly.
In this article, we will learn another simplest method known as factoring by Grouping, but before getting into this topic of factoring by grouping, let’s discuss what factoring a polynomial is.
A polynomial is an algebraic expression with one or more terms in which an addition or a subtraction sign separates a constant and a variable.
The general form of a polynomial is axn + bxn-1 + cxn-2 + …. + kx + l, where each variable has a constant accompanying it as its coefficient. The different types of polynomials include; binomials, trinomials, and quadrinomial.
Examples of polynomials are; 12x + 15, 6x2 + 3xy – 2ax – ay, 6x2 + 3x + 20x + 10 etc.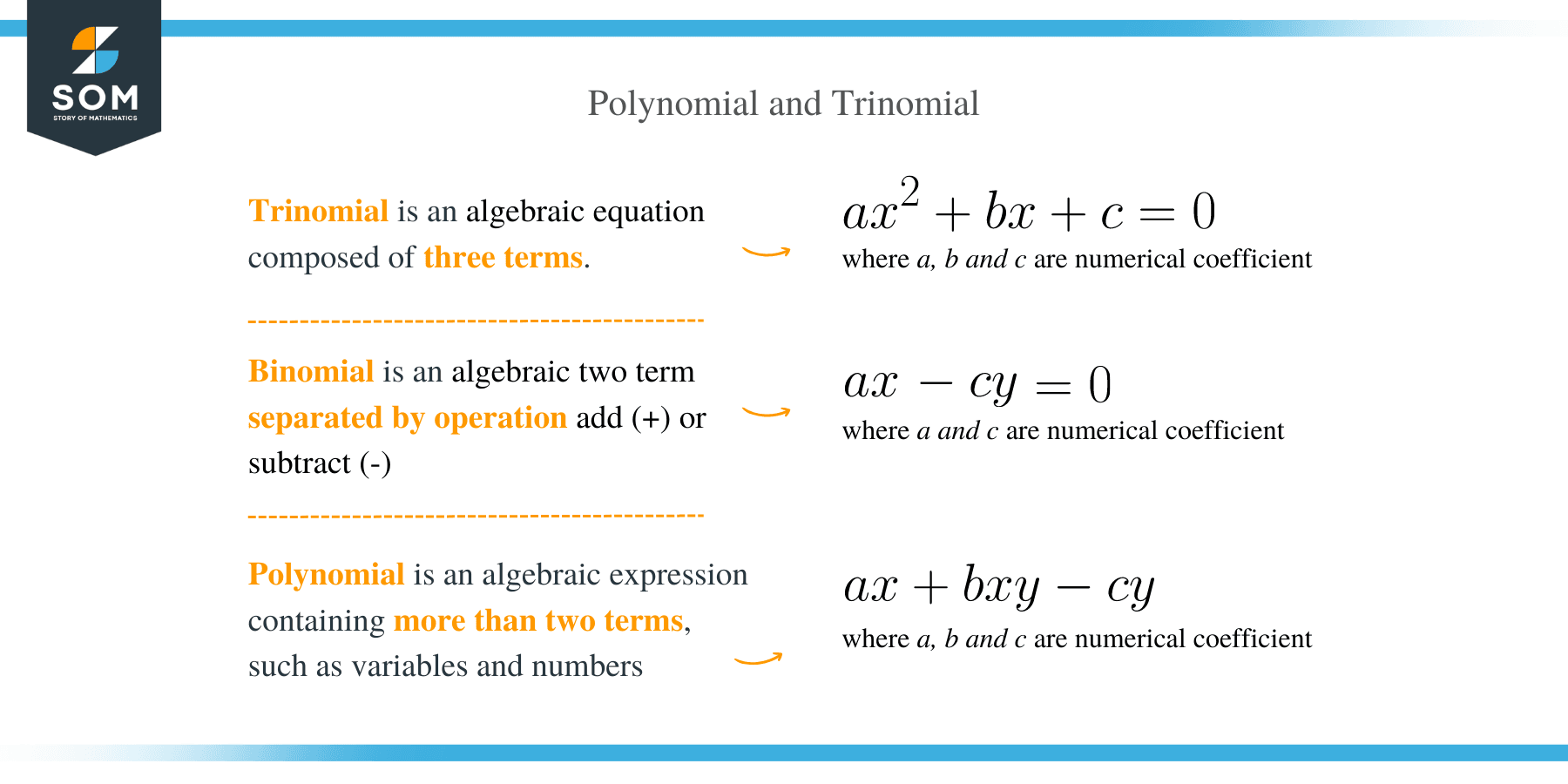
How to Factor by Grouping?
Factor by Grouping is useful when there is no common factor among the terms, and you split the expression into two pairs and factor each of them separately.
Factoring polynomials is the reverse operation of multiplication because it expresses a polynomial product of two or more factors. You can factor polynomials to find the roots or solutions of an expression.
How to factor trinomials by grouping?
To factor a trinomial of the form ax2 + bx + c by grouping, we carry out the procedure as shown below:
- Find the product of the leading coefficient “a” and the constant “c.”
⟹ a * c = ac
- Look for the factors of the “ac” that add to coefficient “b.”
- Rewrite bx as a sum or difference of the factors of ac that add to b.
⟹ ax2 + bx + c = ax2 + (a + c) x + c
⟹ ax2 + ax + cx + c
- Now factor by grouping.
⟹ ax (x + 1) + c (x + 1)
⟹ (ax + c) (x + 1)
Example 1
Factor x2 – 15x + 50
Solution
Find the two numbers whose sum is -15 and product is 50.
⟹ (-5) + (-10) = -15
⟹ (-5) x (-10) = 50
Rewrite the given polynomial as;
x2-15x + 50⟹ x2-5x – 10x + 50
Factorize each set of groups;
⟹ x(x – 5) – 10(x – 5)
⟹ (x – 5) (x – 10)
Example 2
Factor the trinomial 6y2 + 11y + 4 by grouping.
Solution
6y2 + 11y + 4 ⟹ 6y2 + 3y + y + 4
⟹ (6y2 + 3y) + (8y + 4)
⟹ 3y (2y + 1) + 4(2y + 1)
= (2y + 1) (3y + 4)
Example 3
Factor 2x2 – 5x – 12.
Solution
2x2 – 5x – 12
= 2x2 + 3x – 8x – 12
= x (2x + 3) – 4(2x + 3)
= (2x + 3) (x – 4)
Example 4
Factor 3y2 + 14y + 8
Solution
3y2 + 14y + 8 ⟹ 3y2 + 12y + 2y + 8
⟹ (3y2 + 12y) + (2y + 8)
= 3y (y + 4) + 2(y + 4)
Hence,
3y2 + 14y + 8 = (y + 4) (3y + 2)
Example 5
Factor 6x2– 26x + 28
Solution
Multiply the leading coefficient by the last term.
⟹ 6 * 28 = 168
Find two numbers whose sum is product is 168 and sum is -26
⟹ -14 + -12 = -26 and -14 * -12 = 168
Write the expression by replacing bx with the two numbers.
⟹ 6x2– 26x + 28 = 6x2 + -14x + -12x + 28
6x2 + -14x + -12x + 28 = (6x2 + -14x) + (-12x + 28)
= 2x (3x + -7) + -4(3x + -7)
Therefore, 6x2– 26x + 28 = (3x -7) (2x – 4)
How to factor binomials by grouping?
A binomial is an expression with two terms combined by either addition or subtraction sign. To factor a binomial, the following four rules are applied:
- ab + ac = a (b + c)
- a2– b2 = (a – b) (a + b)
- a3– b3 = (a – b) (a2 +ab + b2)
- a3+ b3 = (a + b) (a2 – ab + b2)
Example 6
Factor xyz – x2z
Solution
xyz – x2z = xz (y – x)
Example 7
Factor 6a2b + 4bc
Solution
6a2b + 4bc = 2b (3a2 + 2c)
Example 8
Factor completely: x6 – 64
Solution
x6 – 64 = (x3)2 – 82
= (x3 + 8) (x3 – 8) = (x+2) (x2 − 2x + 4) (x − 2) (x2 + 2x + 4)
Example 9
Factor: x6 – y6.
Solution
x6 – y6 = (x + y) (x2 – xy + y2) (x − y) (x2 + xy + y2)
How to factor polynomials by grouping?
As the name suggests, factoring by grouping is simply the process of grouping terms with common factors before factoring.
To factor a polynomial by grouping, here are the steps:
- Check whether the terms of the polynomial have the Greatest Common Factor(GCF). If so, factor it out and remember to include it in your final answer.
- Break up the polynomial into sets of two.
- Factor out the GCF of each set.
- Finally determine if the remaining expressions can be factored any further.
 Example 10
Example 10
Factorize 2ax + ay + 2bx + by
Solution
2ax + ay + 2bx + by
= a (2x + y) + b (2x + y)
= (2x + y) (a + b)
Example 11
Factor ax2 – bx2 + ay2 – by2 + az2 – bz2
Solution
ax2 – bx2 + ay2 – by2 + az2 – bz2
= x2(a – b) + y2(a – b) + z2(a – b)
= (a – b) (x2 + y2 + z2)
Example 12
Factor 6x2 + 3xy – 2ax – ay
Solution
6x2 + 3xy – 2ax – ay
= 3x (2x + y) – a (2x + y)
= (2x + y) (3x – a)
Example 13
x3 + 3x2 + x + 3
Solution
x3 + 3x2 + x + 3
= (x3 + 3x2) + (x + 3)
= x2(x + 3) + 1(x + 3)
= (x + 3) (x2 + 1)
Example 14
6x + 3xy + y + 2
Solution
6x + 3xy + y + 2
= (6x + 3xy) + (y + 2)
= 3x (2 + y) + 1(2 + y)
= 3x (y + 2) + 1(y + 2)
= (y + 2) (3x + 1)
= (3x + 1) (y + 2)
Example 15
ax2 – bx2 + ay2 – by2 + az2 – bz2
Solution
ax2 – bx2 + ay2 – by2 + az2 – bz2
Factor out GCF in each group of the two terms
⟹ x2(a – b) + y2(a – b) + z2(a – b)
= (a – b) (x2 + y2 + z2)
Example 16
Factor 6x2 + 3x + 20x + 10.
Solution
Factor out the GCF in each set of two terms.
⟹ 3x (2x + 1) + 10(2x + 1)
= (3x + 10) (2x + 1)
