Integrals of Inverse Trig Functions – Definition, Formulas, and Examples

Integrals of inverse trig functions will make complex rational expressions easier to integrate. In this discussion, we’ll focus on integrating expressions that result in inverse trigonometric functions.

Integrating functions with denominators of the forms,$\boldsymbol{\sqrt{a^2 – u^2}}$, $\boldsymbol{a^2 + u^2}$, and $\boldsymbol{u\sqrt{u^2 – a^2}}$, will result in inverse trig functions. Integrals resulting in inverse trig functions are normally challenging to integrate without the formulas derived from the derivative of inverse functions.

In the past, we’ve learned how inverse trigonometric functions can help us find unknown angles and solve word problems involving right triangles. We’ve expanded our understanding of inverse trigonometric functions by learning how to differentiate them. This time, we’ll learn how inverse trigonometric functions can help us in integrating rational expressions with complex denominators.

What are the integrals the result in an inverse trig function?

Establishing the integral formulas that lead to inverse trig functions will definitely be a lifesaver when integrating rational expressions such as the ones shown below.

\begin{aligned}{\color{Teal} \dfrac{dx}{\sqrt{1 – 25x^2}}}, \phantom{x}{\color{DarkOrange} \dfrac{dx}{4x^2 + 9}}, \phantom{x}{\color{Orchid} \dfrac{dx}{x\sqrt{16x^2 – 25}}}\end{aligned}

Integral formulas involving inverse trigonometric functions can be derived from the derivatives of inverse trigonometric functions. For example, let’s work with the derivative identity, $\dfrac{d}{dx} \sin^{-1}x = \dfrac{1}{\sqrt{1 – x^2}}$. We can apply the fundamental theorem of calculus to derive the integral formula involving the inverse sine function.

\begin{aligned}\dfrac{d}{dx} \sin^{-1}x &= \dfrac{1}{\sqrt{1 – x^2}}\\ \int\dfrac{d}{dx} (\sin^{-1}x) \phantom{x}dx &= \int \dfrac{1}{\sqrt{1 – x^2}}\phantom{x}dx\\ \sin^{-1}x + C &= \int \dfrac{1}{\sqrt{1-x^2}} \phantom{x}dx\\\int \dfrac{1}{\sqrt{1-x^2}} \phantom{x}dx &= \sin^{-1}x + C\end{aligned}

We’ll show you the rest of the integral rules involving inverse trigonometric functions. This is a simpler version of the rules because we’re deriving them from the derivative rules we’ve learned in the past.

Noticed how each pair of cofunctions ($\sin x \phantom{x}\&\phantom{x} \cos x$, $\sec x \phantom{x}\&\phantom{x} \csc x$, and $\tan x \phantom{x}\&\phantom{x} \cot x$) have derivatives that only differ by sign? This is why we only focus on three integral rules involving trigonometric functions.

The table below shows the three important integral rules to keep in mind. Take note of the denominator’s forms closely since they will immediately tell you the integral rule we need to apply.

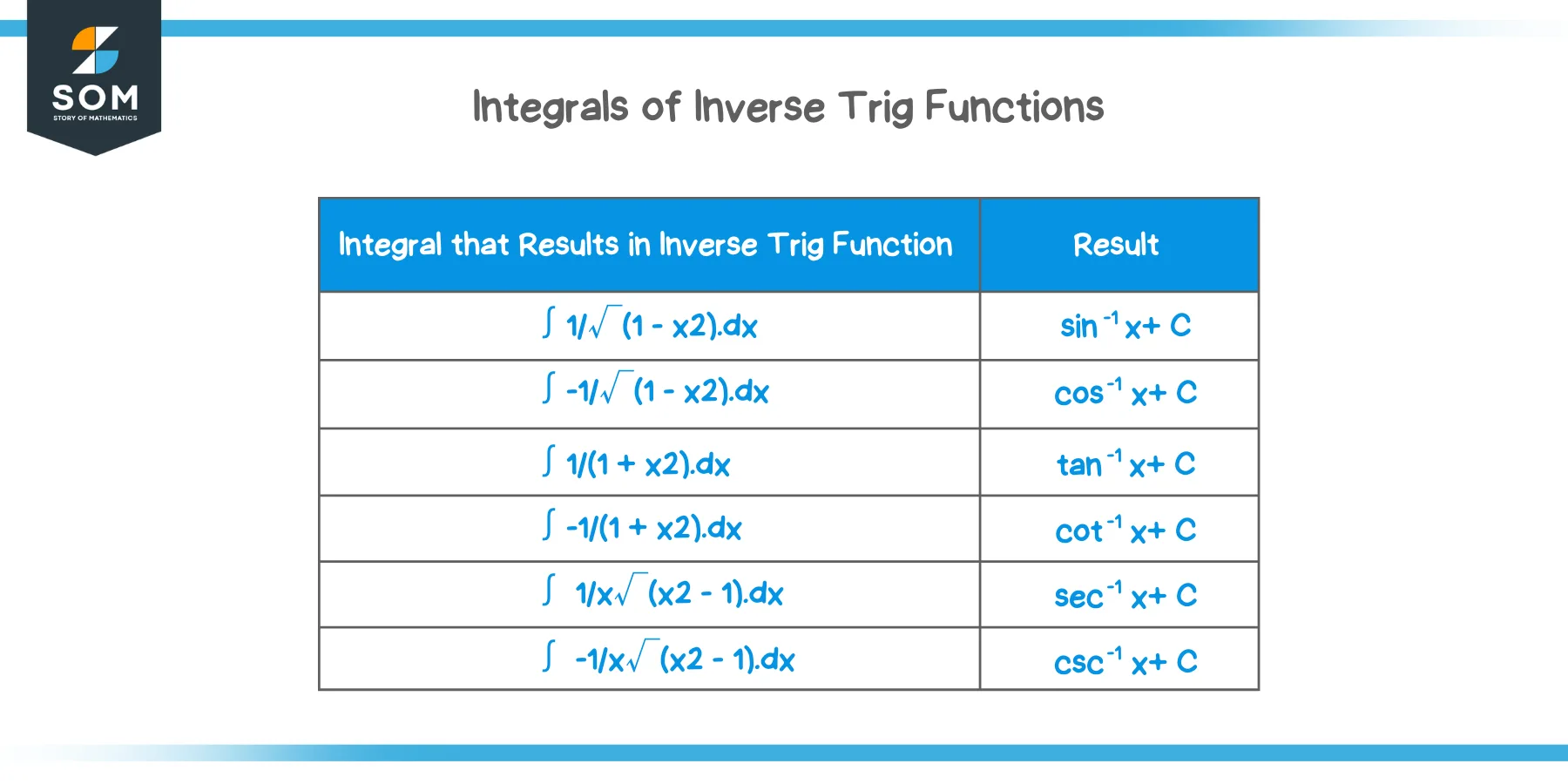Keep in mind that $a$ is a positive constant and $u$ represents the variable that we’re working on. In the next section, we’ll show you the different cases that we’ll encounter when integrating functions with inverse trig functions as their antiderivative. There are instances when we’ll have to use other integration techniques such as the substitution method. Keep your notes handy in case you need a refresher.

How to integrate functions resulting in inverse trig functions?

We can group functions into three groups: 1) integrals that result in inverse sine function, 2) functions with an inverse secant function as its antiderivative, and 3) functions returning an inverse tangent function when integrated.

Below are guidelines in integrating functions that result in having inverse trigonometric functions as their antiderivative:

• Identify the denominator’s form to help you determine which of the three formulas apply.

\begin{aligned}\int\dfrac{dx}{\color{Teal}\sqrt{a^2 – u^2}} &\Rightarrow \color{Teal} \sin^{-1}\dfrac{u}{a} + C\\ \int\dfrac{dx}{\color{DarkOrange} a^2 + u^2} &\Rightarrow \color{DarkOrange}\dfrac{1}{a} \tan^{-1}\dfrac{u}{a} + C\\\int\dfrac{dx}{\color{Orchid} u\sqrt{u^2 – a^2}} &\Rightarrow \color{Orchid}\dfrac{1}{a} \sec^{-1}\dfrac{u}{a} + C\end{aligned}

• Determine the values of $a$ and $u$ from the given expression.
• Apply the substitution method whenever necessary. If the substitution method does not apply, see if we can integrate the expression by parts instead.
• When the expression is simplified and we can now use the appropriate antiderivative formulas.

These are just key pointers to remember and steps may vary depending on the given integrand. Learning how to integrate functions that result in inverse trigonometric functions requires practice. This is why the best way to learn the process is by working on functions and mastering each of the three formulas.

Let’s go back to the three integrands we’ve shown from the earlier section:

\begin{aligned}{\color{Teal} \dfrac{dx}{\sqrt{1 – 25x^2}}}, \phantom{x}{\color{DarkOrange} \dfrac{dx}{4x^2 + 9}}, \phantom{x}{\color{Orchid} \dfrac{dx}{x\sqrt{16x^2 – 25}}}\end{aligned}

In the past, we will have a difficult time integrating these three functions. We’ll show you how to use the formulas for the integrals involving inverse trigonometric functions using these three functions.

Applying the formula: $\boldsymbol{\int \dfrac{du}{\sqrt{a^2 – u^2}} = \sin^{-1} \dfrac{u}{a} + C }$

Let’s start by showing you how we can use the integral formula and return a sine inverse function when integrated.

\begin{aligned} \color{Teal}\int \dfrac{dx}{\sqrt{1 – 25x^2}}\end{aligned}

Inspecting the denominator, we have $\sqrt{1^2 – (5x)^2}$, so the best formula to use for our function is $\int \dfrac{du}{\sqrt{a^2 – u^2}} = \sin^{-1} \dfrac{u}{a} + C$, where $a =5$ and $u = 5x$. Whenever you see the square root of the difference between a perfect square constant and function, keep the inverse sine function formula in mind right away.

For us to apply the formula, we’ll need to use the substitution method and rewrite the integrand as shown below.

\begin{aligned} u &= 5x\\du &= 5\phantom{x}dx\\ \dfrac{1}{5}\phantom{x}du &= dx\\\\\int \dfrac{dx}{\sqrt{1 – 25x^2}} &= \int \dfrac{\dfrac{1}{5}du}{\sqrt{1 – u^2}}\\ &= \dfrac{1}{5}\int \dfrac{du}{\sqrt{1 – u^2}}\end{aligned}

We now have a denominator with $u^2$ in its second term inside the radical, so let’s apply the appropriate formula that will return a sine inverse function.

\begin{aligned} \int \dfrac{du}{\sqrt{a^2 – u^2}} &= \sin^{-1} \dfrac{u}{a} + C\\\\\dfrac{1}{5}\int \dfrac{du}{\sqrt{1 – u^2}} &= \dfrac{1}{5}\sin^{-1} \dfrac{u}{1} + C\\&= \dfrac{1}{5}\sin^{-1} u + C\end{aligned}

Since we earlier assigned $u$ to be $5x$, we substitute this expression back so we have an antiderivative that is in terms of the original variable, $x$.

This example shows us how from a rational expression that contains a radical denominator, we’ve integrated the expression and returned a sine inverse function instead. What was once challenging or even impossible for us to integrate, we now have three solid strategies all thanks to inverse trig functions.

Applying the formula: $\boldsymbol{\int \dfrac{du}{a^2  + u^2 } = \dfrac{1}{a}\tan^{-1} \dfrac{u}{a} + C }$

We’ve seen how we can use the integral formula that involves the sine inverse function, so now, let’s see how we end up with a tangent inverse function when integrating functions with a similar form like the one shown below.

\begin{aligned} {\color{DarkOrange} \int \dfrac{dx}{4x^2 + 9}}\end{aligned}

When you see a denominator that is the sum of two perfect squares, this is a great indicator that we’re expecting an inverse tangent function as its antiderivative.

Since the function we’re working with has a form of $\dfrac{du}{a^2  +u^2 }$, use the formula that results to an inverse tangent function: $\int \dfrac{du}{a^2  + u^2 } \dfrac{1}{a}\tan^{-1} \dfrac{u}{a} + C $, where $a =3$ and $u = 2x$.

As with our previous example, since we have a coefficient before $x^2$, let’s apply the substitution method to rewrite the integrand.

\begin{aligned} u &= 2x \\du &= 2\phantom{x}dx\\ \dfrac{1}{2} \phantom{x}du &= dx\\\\\int \dfrac{dx}{4x^2 + 9} &= \int \dfrac{\dfrac{1}{2}\phantom{x}du}{u^2 + 9}\\ &=\dfrac{1}{2}\int \dfrac{du}{u^2 + 9}\end{aligned}

Apply the appropriate integral properties and formulas to evaluate our new expression.

\begin{aligned} \dfrac{1}{2}\int \dfrac{du}{u^2 + 9} &=\dfrac{1}{2}\int \dfrac{du}{3^2 + u^2}\\&= \dfrac{1}{2}\left[\dfrac{1}{3} \tan^{-1}\dfrac{u}{3} \right ] + C\\&= \dfrac{1}{6} \tan^{-1}\dfrac{u}{3} + C\end{aligned}

Since we used the substitution method earlier, make sure to replace $u$ with $2x$ back to return an integral in terms of $x$.

Apply a similar process when integrating functions with a similar form. Here’s another tip to remember: when given a definite integral, just focus on integrating the expression first then evaluate the antiderivatives later.

Applying the formula: $\boldsymbol{\dfrac{du}{u\sqrt{u^2 – a^2}} = \dfrac{1}{a}\sec^{-1} \dfrac{u}{a} + C }$

We’ll now work on the third possible outcome: integrating the functions and getting an inverse secant function as a result.

\begin{aligned} {\color{Orchid} \int \dfrac{dx}{x\sqrt{16x^2 – 25}}}\end{aligned}

The integrand has the form, $\dfrac{du}{x\sqrt{u^2  -a^2}}$, so apply the formula that returns an inverse secant function: $\int \dfrac{du}{ x\sqrt{u^2  -a^2}} \dfrac{1}{a}\sec^{-1} \dfrac{u}{a} + C $, where $a =5$ and $u = 4x$. What makes this form unique is that aside from the radical expression, we see a second factor in the denominator. If the second factor remains after simplifying the integrand, then expect an inverse secant function for its antiderivative.

Since we still have a coefficient before the variable inside the radical, use the substation method and use $u = 4x$ and $u^2 = 16x^2$.

\begin{aligned} u &= 4x\\\dfrac{1}{4}u &= x\\\dfrac{1}{4}\phantom{x}du &= dx\\\\\int \dfrac{dx}{x\sqrt{16x^2 – 25}} &= \int \dfrac{\dfrac{1}{4}\phantom{x}du}{\dfrac{1}{4}u \sqrt{u^2 – 25}}\\&= \int \dfrac{du}{u\sqrt{u^2 – 25}} \end{aligned}

Now that we’ve rewritten the integrand into a form where the inverse secant function formula applies, let’s now integrate the expression as shown below.

\begin{aligned} \int \dfrac{du}{u\sqrt{u^2 – 25}} &= \int \dfrac{du}{u\sqrt{u^2 – 5^2}}\\&= \dfrac{1}{5} \sec^{-1}\dfrac{u}{5} +C  \end{aligned}

Since we applied the substitution method in the earlier step, substitute $u = 4x$ back into the resulting expression.

In the past, integrating functions such as $\dfrac{1}{x\sqrt{16x^2 – 25}}$ is very intimidating, but with the help of integrals involving inverse trigonometric functions, we now have three key tools to use to integrate complex rational expressions.

This is why we’ve allotted a special section for you to continue practicing this new technique. When you’re ready, head over to the next section to try out more integrals and apply the three formulas you’ve just learned!

Example 1

Evaluate the indefinite integral, $\int \dfrac{dx}{\sqrt{36 – x^2}} $.

Solution

From the denominator, we can see that it’s the square root of the difference between $36 = 6^2$ and $x^2$. With this form, we’re expecting the antiderivative to be an inverse sine function.

Apply the first integral formula, $\int \dfrac{du}{\sqrt{a^2 – u^2}} = \sin^{-1} \dfrac{u}{a} + C$, where $a = 6$ and $u = x$.

\begin{aligned}\int \dfrac{dx}{\sqrt{36 – x^2}} &= \sin^{-1}\dfrac{x}{6} +C \end{aligned}

Hence, we have $\int \dfrac{dx}{\sqrt{36 – x^2}}=  \sin^{-1}\dfrac{x}{6} +C$.

This is the simplest form for this type of function, so head over to our first practice question if you want to practice on simpler functions first. When ready, move on to the second problem.

Example 2

Calculate the definite integral, $\int_{0}^{\sqrt{3}/2} \dfrac{dx}{25x^2 + 4}$.

Solution

Let’s disregard the lower and upper limits first and integrate $\int \dfrac{dx}{25x^2 + 4}$. As we have mentioned in our discussion, it’s best to focus on integrating the function first then simply evaluating the values at the lower and upper limits afterward.

The denominator is a sum of two perfect squares: $(5x)^2$ and $2^2$.

\begin{aligned} \int \dfrac{dx}{25x^2 + 4} &= \int \dfrac{dx}{(5x)^2 + 2^2}\end{aligned}

This means that we can integrate the expression by using the integral formula that results to an inverse tangent function: $\int \dfrac{du}{a^2  + u^2 } \dfrac{1}{a}\tan^{-1} \dfrac{u}{a} + C$, where $a = 2$ and $u = 5x$. Since we’re working with $u =5x$, apply the substitution method first as shown below.

 \begin{aligned} u &= 5x\\du &= 5\phantom{x}dx\\\dfrac{1}{5}\phantom{x}du &= dx\\\\\int \dfrac{dx}{25x^2 + 4} &= \int \dfrac{\dfrac{1}{5}\phantom{x}du}{u^2 + 4}\\&= \dfrac{1}{5}\int \dfrac{du}{u^2 + 4}\end{aligned}

Integrate the resulting expression then substitute $u = 5x$ back into the resulting integral.

\begin{aligned} \dfrac{1}{5}\int \dfrac{du}{u^2 + 4} &= \dfrac{1}{5}\left[\dfrac{1}{2}\tan^{-1}\dfrac{u}{2} + C \right ]\\&= \dfrac{1}{10} \tan^{-1}\dfrac{5x}{2} + C\end{aligned}

Now that we have $\int \dfrac{dx}{25x^2 + 4} = \dfrac{1}{10} \tan^{-1}\dfrac{5x}{2} + C$. Evaluate the expression at $x = \dfrac{\sqrt{3}}{2}$ and $x = 0$ then subtract the result.

\begin{aligned}\int_{0}^{\sqrt{3}/2} \dfrac{dx}{25x^2 + 4} &= \left[\dfrac{1}{10} \tan^{-1}\dfrac{5x}{2} \right ]_{0}^{\sqrt{3}/2}\\&= \dfrac{1}{10}\left[\left(\tan^{-1}\dfrac{5 \cdot \sqrt{3}/2}{2}\right) -\left(\tan^{-1}\dfrac{5 \cdot 0}{2}\right) \right ]\\&= \dfrac{1}{10}\tan^{-1}\dfrac{5\sqrt{3}}{4} \end{aligned}

Hence, we have $\int_{0}^{\sqrt{3}/2} \dfrac{dx}{25x^2 + 4} = \dfrac{1}{10} \tan^{-1}\dfrac{5\sqrt{3}}{4} $.

Example 3

Evaluate the indefinite integral, $\int \dfrac{3}{2x\sqrt{16x^4 – 9}} \phantom{x}dx$.

Solution

Factor out $\dfrac{3}{2}$ from the integral expression.

\begin{aligned}\int \dfrac{3}{2x\sqrt{16x^4 – 9}} \phantom{x}dx &= \dfrac{3}{2} \int \dfrac{dx}{x\sqrt{16x^4 – 9}} \end{aligned}

We can see that the integrand’s denominator is a product of a variable and a radical expression: $x$ and $\sqrt{16x^4 – 9}$. When this happens, we can use the third formula returning an inverse secant function: $\int \dfrac{du}{a^2  + u^2 } \dfrac{1}{a}\tan^{-1} \dfrac{u}{a} + C$, where $a = 3$ and $u = 4x^2$.

Apply the substitution method by using $u = 4x^2$, $\dfrac{u}{4} = x^2$, and $u^2 = 16x^4$ as shown below.

\begin{aligned}u &= 4x^2\\du &= 8x \phantom{x}dx\\\dfrac{1}{8x}\phantom{x}du&= dx\\\\\dfrac{3}{2} \int \dfrac{dx}{x\sqrt{16x^4 – 9}} &= \dfrac{3}{2}\int\dfrac{\dfrac{1}{8x}\phantom{x} du}{x\sqrt{u^2 – 9}}\\&= \dfrac{3}{16}\int \dfrac{du}{x^2\sqrt{u^2 – 9}}\\&= \dfrac{3}{16}\int \dfrac{du}{{\color{Teal}\dfrac{u}{4}}\sqrt{u^2 – 9}}, \phantom{x}\color{Teal} \dfrac{u}{4} = x^2\\&= \dfrac{3}{4}\int \dfrac{du}{u\sqrt{u^2 – 9}} \end{aligned}

Now that we have the integrand in the right form for the inverse secant function, let’s apply the integral formula.

\begin{aligned}\dfrac{3}{4}\int \dfrac{du}{u\sqrt{u^2 – 9}}&= \dfrac{3}{4} \left[ \dfrac{1}{3} \sec^{-1}\dfrac{u}{3} +C\right]\\&= \dfrac{1}{4}\sec^{-1} \dfrac{u}{3} +C \end{aligned}

Substitute $u = 4x^2$ back into the expression and we have the antiderivative in terms of $x$.

\begin{aligned}\dfrac{1}{4}\sec^{-1} \dfrac{u}{3} +C &= \dfrac{1}{4}\sec^{-1} \dfrac{4x^2}{3} +C\end{aligned}

Hence, we have $\int \dfrac{3}{2x\sqrt{16x^4 – 9}} \phantom{x}dx = \dfrac{1}{4}\sec^{-1} \dfrac{4x^2}{3} +C $.

Example 4

Evaluate the indefinite integral, $\int \dfrac{dx}{x^2 + 4x + 13}$.

Solution

At first glance, it may appear that this integrand may not benefit from integrals involving inverse trigonometric functions. Let’s go ahead and express the denominator as the sum of a perfect square trinomial and a constant and see what we have.

\begin{aligned}\int \dfrac{dx}{x^2 + 4x + 13} &= \int \dfrac{dx}{(x^2 + 4x + 4) + 9}\\&= \int \dfrac{dx}{(x + 2)^2 + 9}\end{aligned}

In this form, we can see that the integrand’s denominator is a sum of two perfect squares. This means that we can use the integral formula, $\int \dfrac{du}{a^2  + u^2 } \dfrac{1}{a}\tan^{-1} \dfrac{u}{a} + C $, where $a =3$ and $u = x + 2$. But first, let’s apply the substitution method to rewrite the integrand as shown below.

\begin{aligned}u &= x + 2\\ du &= dx\\\\\int \dfrac{dx}{(x + 2)^2 + 9} &= \int \dfrac{du}{u^2 + 9}\end{aligned}

Apply the integral formula now then substitute $u= x+2$ back into the resulting antiderivative.

\begin{aligned}\int \dfrac{du}{u^2 + 9} &= \dfrac{1}{3} \tan^{-1}\dfrac{u}{3} + C\\&= \dfrac{1}{3} \tan^{-1} \dfrac{x + 2}{3} + C\end{aligned}

Hence, we have $\int \dfrac{dx}{x^2 + 4x + 13} = \dfrac{1}{3} \tan^{-1} \dfrac{x + 2}{3} + C $.

This example shows us that there are instances when we have to rewrite the denominators before we can apply one of the three integral formulas that involve inverse trigonometric functions.

We have prepared more practice questions for you, so when you need to work on more problems, check the problems below and master using the three formulas we’ve just learned!

Practice Questions

1. Evaluate the following indefinite integrals:
a. $\int \dfrac{dx}{\sqrt{81 – x^2}} $
b. $\int \dfrac{dx}{x^2 + 16} $
c. $\int \dfrac{dx}{x\sqrt{x^2 – 15}} $

2. Calculate the following definite integrals:
a. $\int_{0}^{\sqrt{2}/2} \dfrac{dx}{\sqrt{16 – 9x^2}} $
b. $\int_{0}^{1} \dfrac{dx}{25x^2 + 81} $
c. $\int_{\sqrt{2}}^{\sqrt{3}} \dfrac{dx}{x\sqrt{x^2 – 1}} $

3. Evaluate the following indefinite integrals:
a. $\int \dfrac{dx}{x^2 – 6x + 18} $
b. $\int \dfrac{4\phantom{x} dx}{5x \sqrt{9x^4 – 4}} $
c. $\int \dfrac{6 \phantom{x}dx}{\sqrt{81 – 16x^2}} $

4. Calculate the following definite integrals:
a. $\int_{2}^{6} \dfrac{dx}{x^2 – 14x + 50} $
b. $\int_{0}^{2} \dfrac{2e^{-2x}}{\sqrt{ 1 – e^{-4x}}}\phantom{x} dx $
c. $\int_{1}^{5} \dfrac{dx}{x\sqrt{25x^2 – 6}} $

Answer Key

1.
a. $\int \dfrac{dx}{\sqrt{81 – x^2}} =\sin^{-1}\dfrac{x}{9} + C$
b. $\int \dfrac{dx}{x^2 + 16} = \dfrac{1}{4}\tan^{-1} \dfrac{x}{4} + C$
c. $\int \dfrac{dx}{x\sqrt{x^2 – 15}} = \dfrac{1}{\sqrt{15}} \sec^{-1} \dfrac{x}{\sqrt{15}} + C$

2.
a. $\int_{0}^{\sqrt{2}/2} \dfrac{dx}{\sqrt{16 – 9x^2}} = \dfrac{1}{3} \sin^{-1}\dfrac{3\sqrt{2}}{8}$
b. $\int_{0}^{1} \dfrac{dx}{25x^2 + 81} = \dfrac{1}{5} \tan^{-1} \dfrac{5}{9}$
c. $\int_{\sqrt{2}}^{\sqrt{3}} \dfrac{dx}{x\sqrt{x^2 – 1}} = \tan^{-1} \sqrt{2} – \dfrac{\pi}{4}$

3.
a. $\int \dfrac{dx}{x^2 – 6x + 18} = \dfrac{1}{3} \tan^{-1} \dfrac{x – 3}{3} +C$
b. $\int \dfrac{4\phantom{x} dx}{5x \sqrt{9x^4 – 4}} = \dfrac{1}{5}\sec^{-1} \dfrac{3x^2}{2} +C $
c. $\int \dfrac{6 \phantom{x}dx}{\sqrt{81 – 16x^2}} = \dfrac{3}{2}\sin^{-1} \dfrac{4x}{9} + C$

4.
a. $\int_{2}^{6} \dfrac{dx}{x^2 – 14x + 50} = -\dfrac{\pi}{4} + \tan^{-1}5$
b. $\int_{0}^{2} \dfrac{2e^{-2x}}{\sqrt{ 1 – e^{-4x}}}\phantom{x} dx = \dfrac{\pi}{2} – \sin^{-1} \dfrac{1}{e^4}$
c. $\int_{1}^{5} \dfrac{dx}{x\sqrt{25x^2 – 16}} = \dfrac{1}{4} \sec^{-1}\dfrac{25}{4} – \dfrac{1}{4} \sec^{-1}\dfrac{5}{4}$