- Home
- >
- Partial Fraction Decomposition – Explanation & Examples
JUMP TO TOPIC
Partial Fraction Decomposition – Explanation & Examples
 What is Partial Fraction Decomposition?
What is Partial Fraction Decomposition?
When adding or subtracting rational expressions, we combine two or more fractions into a single fraction.
For example:
- Add 6/ (x – 5) + (x + 2)/ (x – 5)
Solution
6/ (x – 5) + (x + 2)/ (x – 5) = (6 + x + 2)/ (x -5)
Combine the like terms
= (8 + x)/ (x – 5)
- Subtract 4/ (x2 – 9) – 3/ (x2 + 6x + 9)
Solution
Factor the denominator of each fraction to get the LCD.
4/ (x2 – 9) – 3/ (x2 + 6x + 9) ⟹ 4/ (x -3) (x + 3) – 3/ (x + 3) (x + 3)
Multiply each fraction by LCD (x -3) (x + 3) (x + 3) to get;
[4(x + 3) – 3(x – 3)]/ (x -3) (x + 3) (x + 3)
Remove the parentheses in the numerator.
⟹ 4x +12 – 3x + 9/ (x -3) (x + 3) (x + 3)
⟹ x + 21/ (x -3) (x + 3) (x + 3)
In the above two examples, we have combined the fractions into a single fraction by adding and subtracting. Now the reverse procedure of adding or subtracting fractions is what is called partial fraction decomposition.
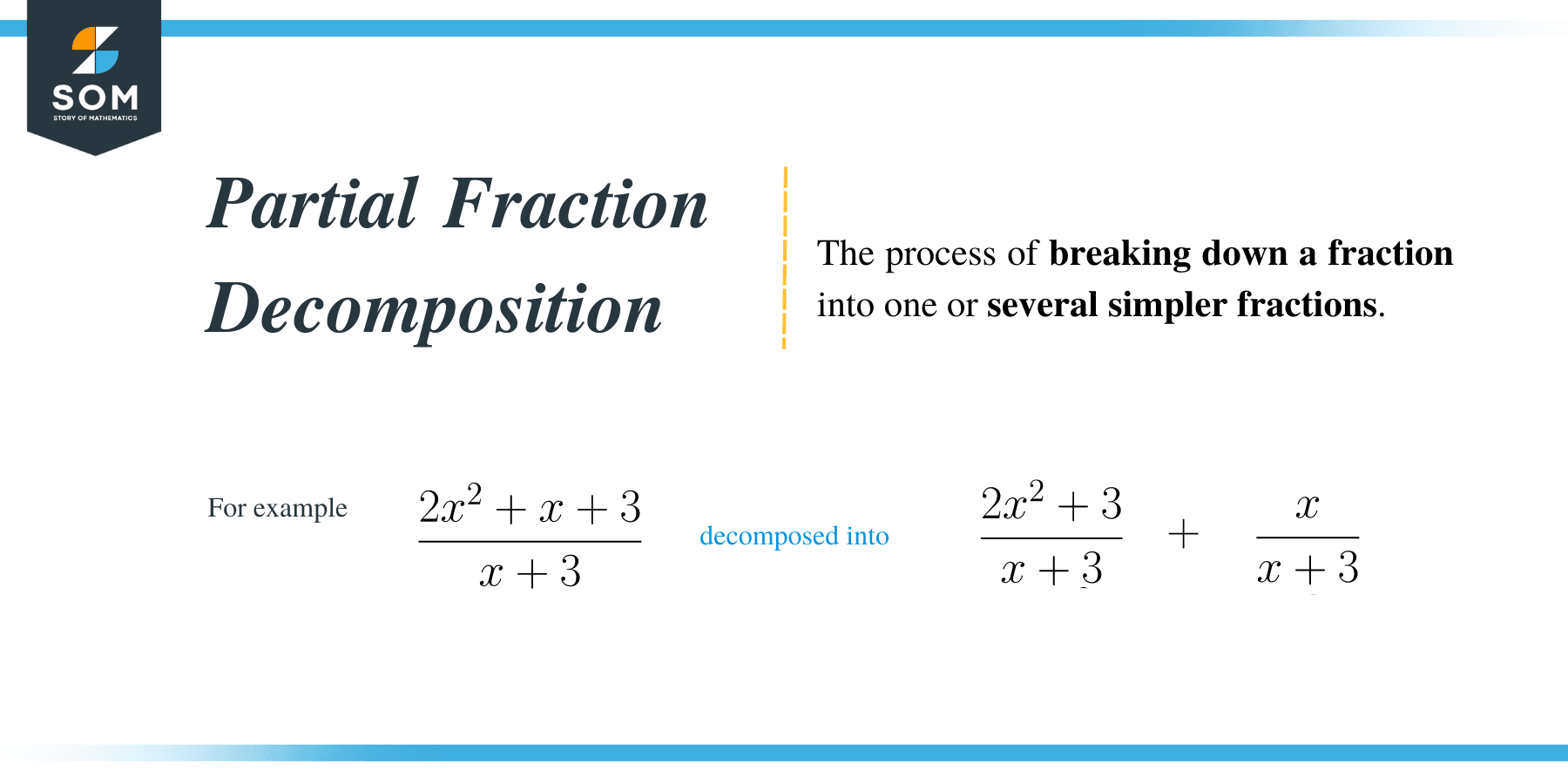
In algebra, partial fraction decomposition is defined as the process of breaking down a fraction into one or several simpler fractions.
 Here are the steps for performing partial fraction decomposition:
Here are the steps for performing partial fraction decomposition:
How to do Partial Fraction Decomposition?
- In case of a proper rational expression, factor the denominator. And if the fraction is improper (the degree of the numerator is greater than the degree of the denominator), do the division first, and then factor the denominator.
- Use the partial fraction decomposition formula (all formulas are mentioned in the table below) to write out a partial fraction for each factor and exponent.
- Multiply through by the bottom and solve for coefficients by equating their factors to zero.
- Finally, write your answer by inserting the obtained coefficients in the partial fraction.
Partial Fraction Decomposition Formula
The table below shows a list of partial decomposition formulas to help in writing out partial fractions. The second row shows how to decompose into partial fractions the factors with exponents.
| Polynomial function | Partial fractions |
| [p(x) + q]/ (x – a) (x – b) | A/ (x- a) + B/ (x – b) |
| [p(x) + q]/ (x – a)2 | A1/ (x – a) + A2/ (x – a)2 |
| (px2 + qx + r)/ (x – a) (x – b) (x – c) | A/ (x – a) + B/ (x – a) + C/ (x – c) |
| [px2 +q(x) + r]/ (x – a)2 (x – b) | A1/ (x – a) + A2/ (x – a)2 + B/(x – b) |
| (px2 + qx + r)/ (x – a) (x2 + bx + c) | A/ (x – a) + (Bx + C)/ (x2 + bx + c) |
Example 1
Decompose 1/ (x2 − a2)
Solution
Factor the denominator and rewrite the fraction.
1/ (x2 − a2) = A/ (x – a) + B/ (x + a)
Multiply through by (x2 − a2)
1/ (x2– a2) = [A (x + a) + B (x – a)]
⟹ 1 = A (x + a) + B (x – a)
When x = -a
1 = B (-a – a)
1 = B(-2a)
B = -1/2a
And when x = a
1 = A (a +a)
1 = A(2a)
A = 1/2a
Now substitute the values of A and B.
= 1/ (x2 − a2) ⟹ [1/2a (x + a)] + [1/2a (x – a)]
Example 2
Decompose: (3x + 1)/ (x – 2) (x + 1)
Solution
(3x + 1)/ (x – 2) (x + 1) = A/ (x – 2) + B/ (x + 1)
By multiplying through by (x – 2) (x + 1), we get;
⟹ 3x + 1 = [A (x + 1) + B (x – 2)]
When x + 1 = 0
x = -1
Substitute x = -1 in the equation 3x + 1 = A (x + 1) + B (x – 2)
3(-1) + 1 = B (-1 -2)
-3 + 1= B (-3)
-2 = – 3B
B =2/3
And when x – 2 =0
x = 2
Substitute x = 2 in the equation 3x + 1 = A (x + 1) + B (x – 2)
3(2) + 1 = A (2 + 1)
6 + 1 = A (3)
7 = 3A
A = 7/3
Hence, (3x + 1)/ (x – 2) (x + 1) = 7/3(x – 2) + 2/ 3(x + 1)
Example 3
Resolve the following rational expressions into partial fractions:
(x2 + 15)/(x + 3)2 (x2 + 3)
Solution
Since the expression (x + 3)2 contains an exponent of 2, it will contain two terms
⟹ (A1 and A2).
(x2 + 3) is a quadratic expression, so it will contain: Bx + C
⟹ (x2 + 15)/(x + 3)2(x2 + 3) = A1/(x + 3) + A2/(x + 3)2 + (Bx + C)/(x2 + 3)
Multiply each fraction by (x + 3)2(x2 + 3).
⟹ x2 + 15 = (x + 3) (x2 + 3) A1 + (x2 + 3) A2 + (x + 3)2(Bx + C)
Starting with x + 3, we get that x + 3 = 0 at x = -3
(−3)2 + 15 = 0 + ((−3)2 + 3) A2 + 0
24 = 12A2
A2=2
Substitute A2 = 2:
= x2 + 15 ⟹ (x + 3) (x2 + 3) A1 + 2x2 + 6 + (x + 3)2 (Bx + C)
Now expand the expressions.
= x2 + 15 ⟹ [(x3 + 3x + 3x2 + 9) A1 + 2x2 + 6 + (x3 + 6x2 + 9x) B + (x2 + 6x + 9) C]
⟹ x2 + 15 = x3(A1 + B) +x2 (3A1 + 6B + C + 2) + x (3A1 + 9B + 6C) + (9A1 + 6 + 9C)
x3 ⟹ 0 = A1 + B
x2 ⟹ 1 = 3A1 + 6B + C + 2
x ⟹ 3A1 + 9B + 6C
The constants ⟹ 15 = 9A1 + 6 + 9C
Now arrange the equations and solve
0 = A1 + B
−1 = 3A1 + 6B + C
0 = 3A1 + 9B + 6C
1 = A1 + C
0 = A1 + B
−2 = 2A1 + 6B
0 = 3A1 + 9B + 6C
1 = A1 + C
On solving, we get;
B = − (1/2), A1 = (1/2) and C = (1/2).
Therefore, x2 + 15/ (x + 3)2(x2 + 3) = 1/ [2(x + 3)] + 2/ (x + 3)2 + (-x + 12)/ (x2 + 3)
Example 4
Decompose x/ (x2 + 1) (x – 1) (x + 2)
Solution
x/ [(x2 + 1) (x – 1) (x + 2)] = [A/ (x – 2)] + [B/ (x + 2)] + [(Cx + D)/ (x2 + 1)]
Multiply through by (x2 + 1) (x – 1) (x + 2)
x = A(x+2) (x2+1) + B(x2+1) (x-1) + (Cx + D) (x-1) (x+2)
When x – 1 = 0
x = 1
Substitute;
1 = A (3)(2)
6A= 1
A=1/6
When x + 2 = 0
x = -2
Substitute;
-2 = B (5) (-3)
-2 = -15B
B = 2/15
When x = 0
x = A(x + 2) (x2 + 1) + B(x2 + 1) (x – 1) + (Cx + D) (x – 1) (x + 2)
⟹ 0 = A (2)(1) + B (1) (-1) + D (-1) (2)
⟹ 0 = 2A – B – 2D
= (1/3) – (2/15) – 2D
2D = 3/15
D = 1/10
When x = -1
-1 = A (1)(2) + B (2) (-2) + (-C+D) (-2) (1)
-1 = 2A – 4B + 2C – 2D
Substitute A, B and D
-1 = (1/3) – (8/15) + 2C – (1/5)
-1 = ((5 – 8 – 3)/15) + 2C
-1 = -6/15 + 2C
-1 + (2/5) = 2 C⟹ -3/5 = 2C ⟹ C = -3/10
Therefore, the answer is;
⟹ [1/6(x – 1)] + [2/15(x + 2)] + [(-3x + 1)/10(x2 + 1)]
