Solving Quadratic Inequalities – Explanation & Examples

Like equations have different forms, inequalities also exist in different forms, and quadratic inequality is one of them.

A quadratic inequality is an equation of second degree that uses an inequality sign instead of an equal sign.

The solutions to quadratic inequality always give the two roots. The nature of the roots may differ and can be determined by discriminant (b² – 4ac).

The general forms of the quadratic inequalities are:

ax² + bx + c < 0

ax² + bx + c ≤ 0

ax² + bx + c > 0

ax² + bx + c ≥ 0

Examples of quadratic inequalities are:

x² – 6x – 16 ≤ 0, 2x² – 11x + 12 > 0, x² + 4 > 0, x² – 3x + 2 ≤ 0 etc.

How to Solve Quadratic Inequalities?

A quadratic inequality is an equation of second degree that uses an inequality sign instead of an equal sign.

Examples of quadratic inequalities are: x² – 6x – 16 ≤ 0, 2x² – 11x + 12 > 0, x² + 4 > 0, x² – 3x + 2 ≤ 0 etc.

Solving a quadratic inequality in Algebra is similar to solving a quadratic equation. The only exception is that, with quadratic equations, you equate the expressions to zero, but with inequalities, you’re interested in knowing what’s on either side of the zero i.e. negatives and positives.

Quadratic equations can be solved by either the factorization method or by use of the quadratic formula. Before we can learn how to solve quadratic inequalities, let’s recall how quadratic equations are solved by handling a few examples.

How Quadratic Equations are Solved by Factorization Method?

Since we know we can similarly solve quadratic inequalities as quadratic equations, it is useful to understand how to factorize the given equation or inequality.

Let’s see a few examples here.

1. 6x²– 7x + 2 = 0

Solution

⟹ 6x² – 4x – 3x + 2 = 0

Factorize the expression;

⟹ 2x (3x – 2) – 1(3x – 2) = 0

⟹ (3x – 2) (2x – 1) = 0

⟹ 3x – 2 = 0 or 2x – 1 = 0

⟹ 3x = 2 or 2x = 1

⟹ x = 2/3 or x = 1/2

Therefore, x = 2/3, ½

2. Solve 3x²– 6x + 4x – 8 = 0

Solution

Factorize the expression on the left-hand side.

⟹ 3x² – 6x + 4x – 8 = 0

⟹ 3x (x – 2) + 4(x – 2) = 0

⟹ (x – 2) (3x + 4) = 0

⟹ x – 2 = 0 or 3x + 4 = 0

⟹ x = 2 or x = -4/3

Therefore, the roots of the quadratic equation are, x = 2, -4/3.

3. Solve 2(x²+ 1) = 5x

Solution

2x² + 2 = 5x

⟹ 2x² – 5x + 2 = 0

⟹ 2x ² – 4x – x + 2 = 0

⟹ 2x (x – 2) – 1(x – 2) = 0

⟹ (x – 2) (2x – 1) = 0

⟹ x – 2 = 0 or 2x – 1 = 0

⟹ x = 2 or x = 1/2

Therefore, the solutions are x = 2, 1/2.

4. (2x – 3)²= 25

Solution

Expand and factorize the expression.

(2x – 3)² = 25

⟹ 4x² – 12x + 9 – 25 = 0

⟹ 4x² – 12x – 16 = 0

⟹ x² – 3x – 4 = 0

⟹ (x – 4) (x + 1) = 0

⟹ x = 4 or x = -1

5. Solve x²+ (4 – 3y) x – 12y = 0

Solution

Expand the equation;

x² + 4x – 3xy – 12y = 0

Factorize;

⟹ x (x + 4) – 3y (x + 4) = 0

x + 4) (x – 3y) = 0

⟹ x + 4 = 0 or x – 3y = 0

⟹ x = -4 or x = 3y

Thus, x = -4 or x = 3y

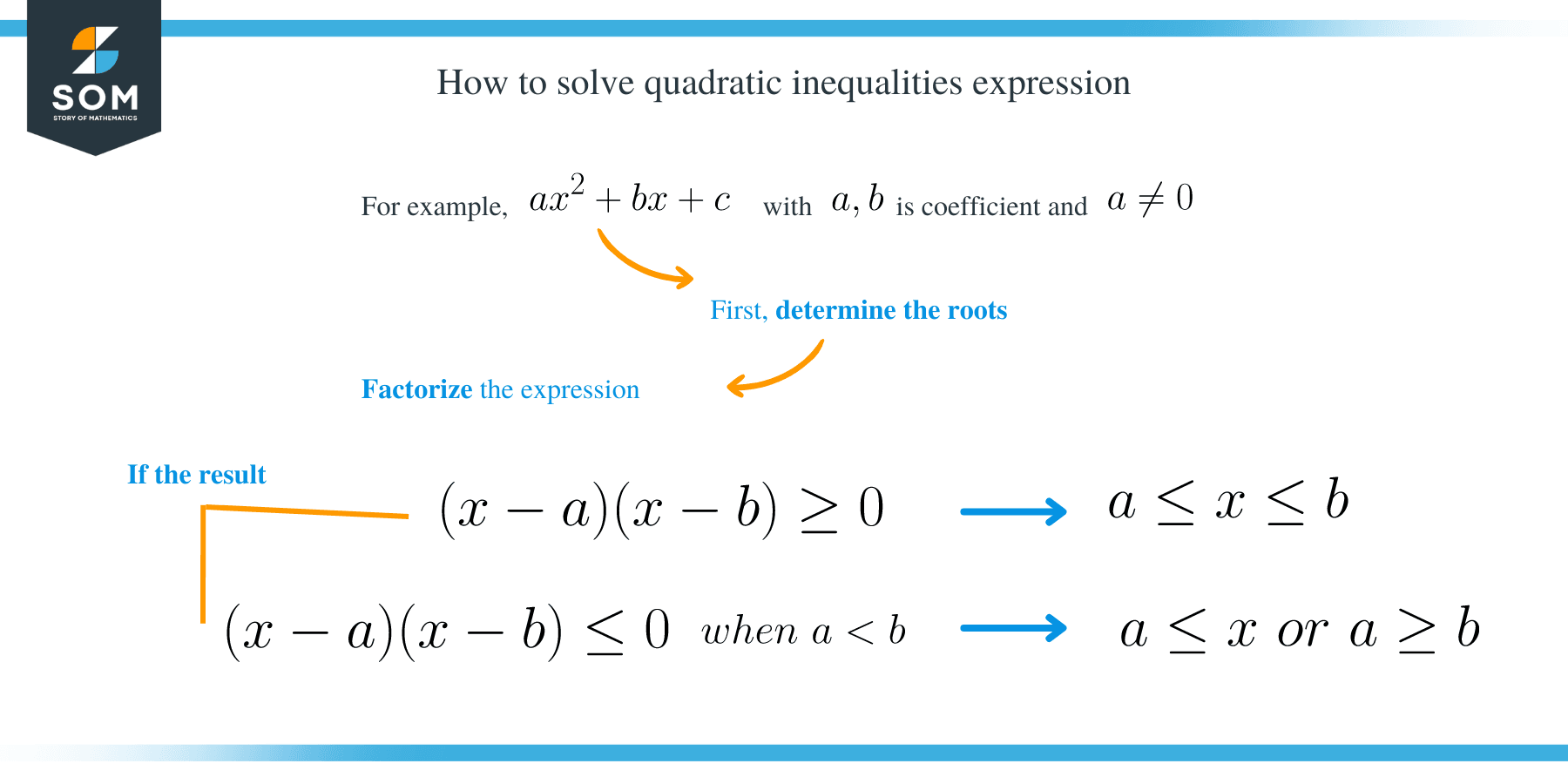
To solve a quadratic inequality, we also apply the same method as illustrated in the procedure below:

• Write the quadratic inequality in standard form: ax² + bx + c where a, b and are coefficients and a ≠ 0
• Determine the roots of the inequality.
• Write the solution in inequality notation or interval notation.
• If the quadratic inequality is in the form: (x – a) (x – b) ≥ 0, then a ≤ x ≤ b, and if it is in the form :(x – a) (x – b) ≤ 0, when a < b then a ≤ x or x ≥ b.

Example 1

Solve the inequality x² – 4x > –3

Solution

First, make one side one side of the inequality zero by adding both sides by 3.

x² – 4x > –3 ⟹ x² – 4x + 3 > 0

Factor the left side of the inequality.

x² – 4x + 3 > 0 ⟹ (x – 3) (x – 1) > 0

Solve for all the zeroes for the inequality;

For, (x – 1) > 0 ⟹ x > 1 and for, (x – 3) > 0 ⟹ x>3

Since y is positive, we therefore choose the values of x which the curve will be above the x-axis.
x < 1 or x > 3

Example 2

Solve the inequality x² – x > 12.

Solution

To write the inequality in standard form, subtract both sides of the inequality by 12.

x² – x > 12 ⟹ x² – x – 12 > 0.

Factorize the quadratic inequality to get to;

(x – 4) (x + 3) > 0

Solve for all the zeroes for the inequality;

For, (x + 3) > 0 ⟹ x > -3

For x – 4 > 0 ⟹ x > 4

The values x < –3 or x > 4 are therefore the solution of this quadratic inequality.

Example 3

Solve 2x² < 9x + 5

Solution

Write the inequality in standard form by making one side of the inequality zero.

2x² < 9x + 5 ⟹ 2x² – 9x – 5 < 0

Factor the left side of the quadratic inequality.

2x² – 9x – 5 < 0 ⟹ (2x + 1) (x – 5) < 0

Solve for all the zeroes for the inequality

For, (x – 5) < 0 ⟹ x < 5 and for (2x + 1) < 0 ⟹ x < -1/2

Since y is negative for the equation 2x² – 9x – 5 < 0, we therefore choose the values of x which the curve will be below the x axis.

Therefore, the solution is   -1/2 < x < 5

Example 4

Solve – x ² + 4 < 0.

Solution

Since the inequality is already in standard form, we therefore factor the expression.

-x ² + 4 < 0 ⟹ (x + 2) (x – 2) < 0

Solve for all the zeroes for the inequality

For, (x + 2) < 0 ⟹ x < -2 and for, (x – 2) < 0 ⟹ x < 2

The y for –x ² + 4 < 0 is negative; therefore, we choose the values of x in which the curve will below the x- axis: –2 < x > 2

Example 5

Solve 2x² + x − 15 ≤ 0.

Solution

Factor the quadratic equation.

2x² + x − 15 = 0

2x² + 6x – 5x− 15 = 0

2x (x + 3) – 5(x + 3) = 0

(2x – 5) (x + 3) = 0

For, 2x – 5 = 0 ⟹ x= 5/2 and for, x + 3= 0 ⟹ x = -3

Since the y for 2x² + x − 15 ≤ 0 is negative, the we choose the values of x in which the curve will be below the x axis. Therefore, x ≤ -3 or x ≥5/2 is the solution.

Example 6

Solve – x² + 3x − 2 ≥ 0

Solution

Multiply the quadratic equation by -1 and remember to change the sign.

x² – 3x + 2 = 0

x² – 1x – 2x + 2 = 0

x (x – 1) – 2(x – 1) = 0

(x – 2) (x – 1) = 0

For, x – 2 = 0 ⟹ x = 2 and for, x – 1= 0 ⟹x=1

Therefore, the solution to the quadratic inequality is 1 ≤ x ≤ 2

Example 7

Solve x² − 3x + 2 > 0

Solution

Factorize the expression to get;

x² − 3x + 2 > 0 ⟹ (x − 2) (x − 1) > 0

Now solve for the roots of the inequality as;

(x − 2) > 0 ⟹ x > 2

(x − 1) > 0 ⟹x > 1

The curve for x² − 3x + 2 > 0 has positive y, therefore which choose the values of x in which the curve will be above the x-axis. The solution is hence, x < 1 or x > 2.

Example 8

Solve −2x² + 5x + 12 ≥ 0

Solution

Multiply the entire expression by -1 and change the inequality sign

−2x² + 5x + 12 ≥ 0 ⟹2x² − 5x − 12 ≤ 0

Factorize the expression to get;

(2x + 3) (x − 4) ≤ 0.

Solve the roots;

(2x + 3) ≤ 0 ⟹ x ≤ -3/2.

(x − 4) ≤ 0 ⟹ x ≤ 4.

By applying the rule; (x – a) (x – b) ≥ 0, then a ≤ x ≤ b, we can comfortably write the solutions of this quadratic inequality as:

-3/2 ≤ x ≤ 4.

Example 9

x² − x − 6 < 0

Solution

Factorize x² − x − 6 to get;

(x + 2) (x − 3) < 0

Find the roots of the equation as;

(x + 2) (x − 3) = 0

x = −2 or x = +3
Because y is negative for x² − x − 6 < 0, then we choose an interval in which the curve will be below the x axis. Therefore, -2 < x < 3 is the solution.