Special Right Triangles – Explanation & Examples
Now you know a triangle is a two-dimensional polygon with 3 sides, 3 angles, and 3 vertices. In this article, we are going to learn other types of triangles known as special right triangles. Before we can start, let‘s recall about a right triangle.
What is a Right triangle?
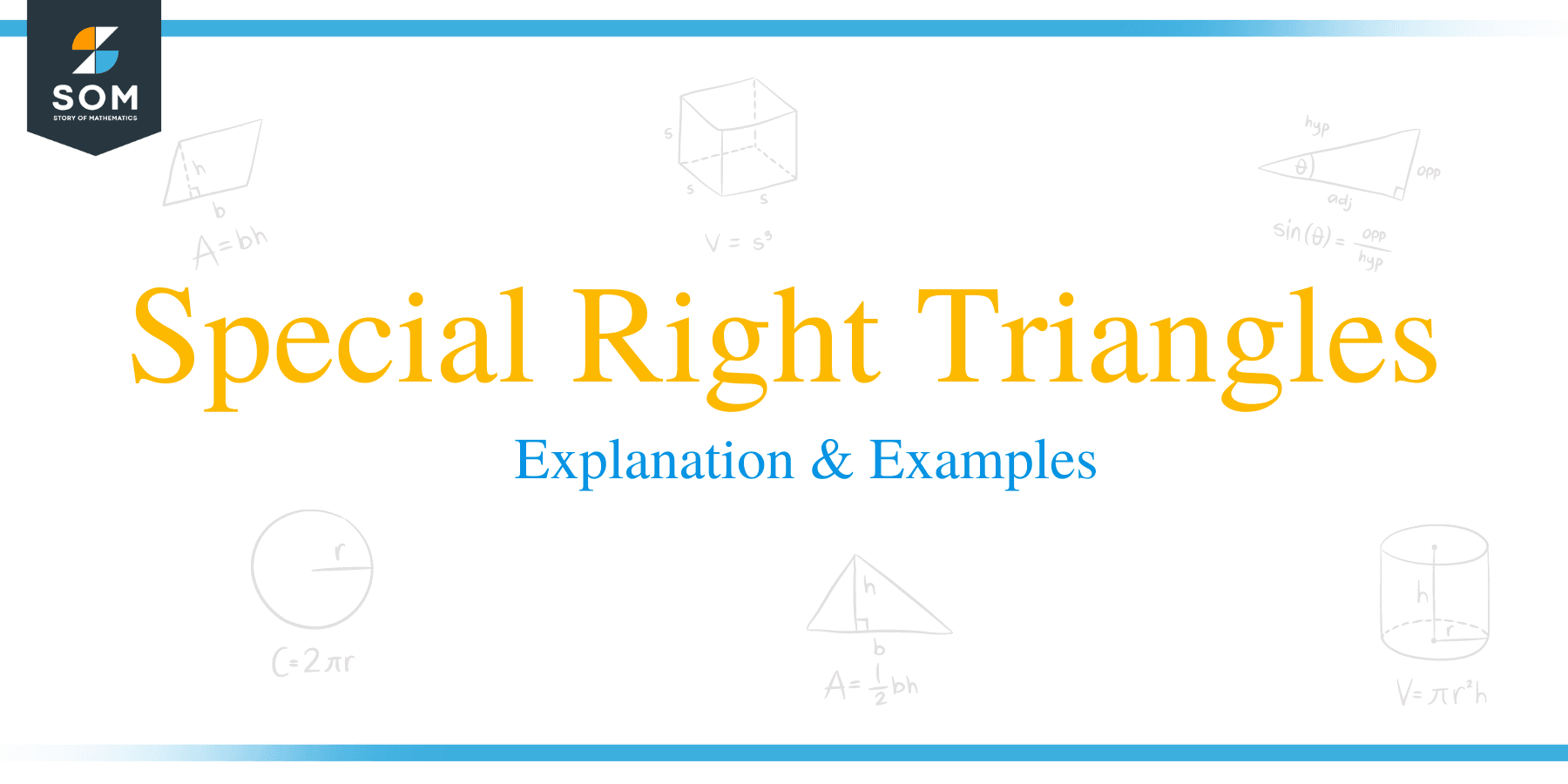
The term “right” refers to the Latin word “rectus,” meaning upright. Therefore, a right triangle is a triangle whose one angle is 90 degrees (right angle). Right triangles are indicated with a box at the location of the right angle.
The longest side of the right triangle on the opposite side of the right angle is known as the hypotenuse. The other two sides of the triangle are known as legs. The horizontal leg is the base, and the vertical leg is the height of a right triangle.
Illustration:
What is a Special Right Triangle?
Special right triangles are triangles whose sides are in a particular ratio, known as Pythagorean Triples. In geometry, the Pythagorean Theorem is a statement that shows the relationship of the sides of a right triangle.
The equation of a right triangle is given by a2 + b2 = c2, where either a or b is the height and base of the triangle and c is the hypotenuse. Using the Pythagorean Theorem, finding the missing side of a triangle is pretty simple and easy.
The two special right triangles include:
- 45°; 45°; 90° Triangle
- 30°; 60°; 90° Triangle
Let’s have a brief overview of these special right triangles as we will see them in detail in the next articles.
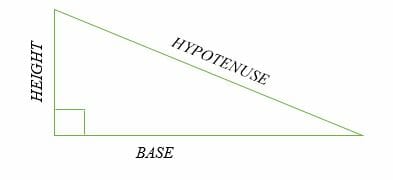
The 45°; 45°; 90° Triangle
This is a special right triangle whose angles are 45°, 45°, and 90°. The base to height ratio to the hypotenuse of this triangle is 1: 1: √2.
Base: Height: Hypotenuse = x: x: x√2 = 1: 1: √2.
In other words, a 45°; 45°; 90° triangle can also be isosceles. An isosceles triangle is a triangle in which two the lengths of its two sides are equal and also the two of its angles are equal.
By using the equation of a right triangle a2 + b2 = c2, we can calculate the hypotenuse of, a 45°; 45°; 90° triangle as follows:
Since, a 45°; 45°; 90° triangle is also an isosceles triangle;
let a = b = x;
x2 + x2 = 2x2
Find the square root of each term in the equation
√x2 + √x2 = √(2x2)
x + x = x √2
Therefore, the hypotenuse of a 45°; 45°; 90° triangle is x √2
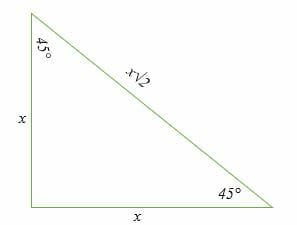
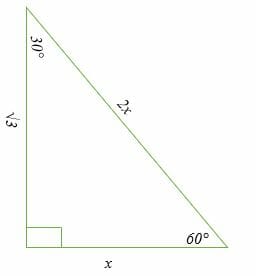
The 30°; 60°; 90° Triangle
This is a special type of right triangle whose angles are 30°; 60°; 90°. The ratio of the lengths of the sides is x: x√3: 2x.
How to Solve Special Right Triangles?
Solving special right triangles means finding the missing lengths of the sides. Instead of using the Pythagorean Theorem, we can use the special right triangle ratios to perform calculations.
Let’s work out a couple of examples.
Example 1
The longer side of a 30°; 60°; 90° right triangle is given by 8√3 cm. What is the measure of its height and hypotenuse?
Solution
The best way to solve these kinds of problems is to sketch the triangles:
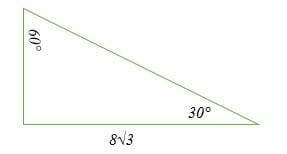
The ratio of a 30°; 60°; 90° right triangle is x: x√3: 2x. In this case, x and x√3 are the shorter and longer sides, respectively, while 2x is the hypotenuse.
Therefore, x√3 = 8√3 cm
Square both sides of the equation.
⇒ (x√3)2 = (8√3)2
⇒ 3x2 = 64 * 3
⇒ x 2 = 64
Find the square of both sides.
√x2 = √64
x = 8cm
Substitute.
2x = 2 * 8 = 16cm.
Therefore, the shorter side is 8cm, and the hypotenuse is 16cm.
Example 2
The hypotenuse of a 45°; 45°; 90° triangle is 6√2 mm. Calculate the length of its base and height.
Solution
Ratio of a 45°; 45°; 90° triangle is x: x: x√2. So, we have;
⇒x√2 = 6√2 mm
Square both sides of the equation.
⇒(x√2)2 = (6√2)2 mm
⇒ 2x2 = 36 * 2
⇒ 2x2 = 72
x2 = 36
Find the square root.
x = 6mm
Substitute x = 6mm in the ratio.
Hence, the base and height of the right triangle are 6mm each.
Example 3
If the diagonal of a right triangle is 8 cm, find the other two sides of the triangle’s lengths given that one of its angles is 30 degrees.
Solution
This is a 30°-60°-90° triangle. Therefore, we use the ratio of x: x√3:2x.
Given, the diagonal = hypotenuse = 8cm.
⇒2x = 8 cm
⇒ x = 4cm
Substitute.
x√3 = 4√3 cm
The shorter side of the right triangle is 4cm, and the longer side is 4√3 cm.
Example 4
Find the hypotenuse of a 30°- 60°- 90° triangle whose longer side is 6 inches.
Solution
Ratio = x: x√3:2x.
⇒ x√3 = 6 inches.
Square both sides
⇒ (x√3)2 = 36
⇒ 3x2 = 36
x2 = 12
x = 2√3 inches.
Example 5
A ladder leaning against a wall makes an angle of 30 degrees with the ground. If the length of the ladder is 9 m, find;
- The height of the wall.
- Calculate the length between the foot of the ladder and the wall.
Solution
Given that one angle is 30 degrees, this must be a 60°- 60°- 90°right triangle.
Ratio = x: x√3:2x.
⇒ 2x = 9
⇒ x = 9/2
= 4.5
Substitute.
- The height of the wall = 4.5m
- x√3 = 4.5√3m
