Law of Sines – Explanation & Examples

The Law of Sines simply relates the lengths of the legs of any triangle to the sines of its corresponding angles. Using the law of sines, we get the flexibility to solve the oblique triangles.

This lesson aims to clear up any confusion you might have about the concepts involving the Law of Sines. We will be also able to answer the following questions.

• What is the law of sines?
• When to use the law of sines?
• How can we use the law of sines?

What Is Law of Sines?

The Law of Sines is the special relationship between the lengths of the legs of any triangle to the sines of its corresponding angles. This can be used to determine the missing parts of oblique triangles. 

Consider a triangle $△ABC$ with the sides $a$, $b$, and $c$ facing the angles $\alpha$, $\beta$, and $\gamma$, respectively, as shown in Figure 13-1.

Note that the side ${\displaystyle {\overline {AC}}}$ of the triangle is along the positive direction of the x-axis with vertex $A$ at the origin, so $∠BAC$ is in the standard position. Thus,

${\displaystyle {\overline {AB}}} = c$

$m∠BAC = \alpha$

Therefore, the coordinates of the point $B$ will be $(c \cos \alpha, c \sin \alpha)%%EDITORCONTENT%%nbsp;

Let us shift the origin from $A$ to $C$, as shown in Figure 13-2.

Looking at the above diagram, $∠BCX$ is in the standard position now. Thus,

$BC = a$

$m∠BCX = 180^{\circ } – \gamma$

Therefore, the coordinates of $B$ are $[a \cos (180^{\circ } – \gamma), a \sin (180^{\circ } – \gamma)]$

As the y-coordinate of B remains the same in both cases.

$a \sin (180^{\circ } – \gamma) = c \sin \alpha$

$a \sin \gamma = c \sin \alpha$

$\frac{a}{\sin\:\alpha\:}=\:\frac{c}{\sin\:\gamma}%%EDITORCONTENT%%nbsp;    — [1]

Similarly, if the side $AB$ is along the positive x-axis, we can determine that:

$\frac{a}{\sin\:\alpha\:}=\:\frac{b}{\sin\:\beta}%%EDITORCONTENT%%nbsp;    — [2]

From $[1]$ and $[2]$, we have

These equal ratios are known as the Law of Sines.

Therefore, the ratio of the length of a leg of a triangle to the sine of the angle opposite that leg remains the same for all legs and angles in a given triangle.

We may come across various situations when we may be asked to solve a triangle other than the right-angled triangle, and the Law of Sines is very capable of dealing with these situations.

When to Use Law of Sines?

We can use the Law of Sines when we are given two angles and the included side to determine a missing side, when we are given two angles and the non-included side to determine a missing side, or when we are given two sides and the non-included angle to determine a missing angle.

We already determined that if $ABC$ with the sides $a$, $b$, and $c$ facing the angles $\alpha$, $\beta$ and $\gamma$, respectively, then

$\frac{a}{\sin\:\alpha\:}=\:\frac{b}{\sin\:\beta} = \frac{c}{\sin\:\gamma\:}$

It indicates that the Law of Sines works with at least two legs and two corresponding angles at a time. Thus we can apply the Law of Sines only if the following conditions are met.

1. When we are given two angles and the included side to determine a missing side — ASA.
2. When we are given two angles and the non-included side to determine a missing side — AAS.
3. When we are given two sides and the non-included angle to determine a missing angle — SSA.

Take note that the third condition is known as the Ambiguous case, and we will explain it in detail in one of our future Trigonometry lessons.

We will only discuss the first two conditions for the moment.

How To Use Law of Sines?

We can use the Law of Sines to find the unknown side or angle by solving for it using the given measurements of the other sides/angles. The following examples will help us determine how we can use the Law of Sines.

When two angles and the included side are given

Example 1

In triangle $△ABC$, $m∠A = 47^{\circ }$, $m∠C = 53^{\circ }$, and $b = 125$. What is the length of side $a$ to the nearest integer?

Solution:

Given:

$m∠A = 47^{\circ }$

$m∠C = 53^{\circ }$

$b = 125$

To find:

length of side $a =$?

First, we need to determine the measure of $∠B$.

We know that the sum of angles in a triangle is $m∠C = 180^{\circ }$.

$m∠A + m∠B + m∠C = 180^{\circ }$

$m∠B = 180^{\circ } \:- \:m∠A\: -\: m∠C$

$m∠B = 180^{\circ }\: – \:47^{\circ }\: – \:53^{\circ }$

$m∠B = 80^{\circ }$

By using Law of Sines, we have

$\frac{a}{\sin\:A\:}=\:\frac{b}{\sin\:B}$

$a = b\:\frac{\sin\:A}{\sin\:B}$

$a = (125)\:\frac{\sin\:47^{\circ }}{\sin\:80^{\circ }}$

substitute $\sin 47^{\circ } = 0.7313537$ and $\sin 80^{\circ } = 0.98480775$

$a = 125×\frac{0.7313537}{0.98480775}$

$a = 125 × 0.74263601$

$a = 92.8295015$

$a ≈ 93$ units

Thus, the length of side $a$ to the nearest integer is approximately $93$ units.

Take note that the length of side $a$ is opposite a smaller angle. What does it indicate? It indicates that the smaller side is opposite a smaller angle.

When two angles and the non-included side are given

Example 2

In triangle $△ABC$, $m∠A = 35^{\circ }$, $m∠B = 45^{\circ }$ and $b = 42$. What is the length of side $c$ to the nearest integer?

Solution:

Given:

$m∠A = 35^{\circ }$

$m∠B = 45^{\circ }$

$b = 42$

To find:

length of side $c =$?

First, we need to determine the measure of $∠C$.

We know that the sum of angles in a triangle is $m∠C = 180^{\circ }$.

$m∠A + m∠B + m∠C = 180^{\circ }$

$m∠C = 180^{\circ } \:- \:m∠A\: -\: m∠B$

$m∠C = 180^{\circ }\: – \:35^{\circ }\: – \:45^{\circ }$

$m∠C = 180^{\circ }\: – \:80^{\circ }$

$m∠C = 100^{\circ }$

By Law of Sines, we have

$\frac{c}{\sin\:C\:}=\:\frac{b}{\sin\:B}$

$c = b\:\frac{\sin\:C}{\sin\:B}$

$c = (42)\:\frac{\sin\:100^{\circ }}{\sin\:45^{\circ }}$

substitute $\sin 100^{\circ } = 0.98480775$ and $\sin 45^{\circ } = 0.70710678$

$c = 42×\frac{0.98480775}{0.70710678}$

$a = 42 × 1.39272848$

$c = 58.4945961$

$a ≈ 58$ units (Rounded to the nearest integer)

Thus, the length of side $c$ is $58$ units.

Take note that the length of side ‘$c$’ is opposite a larger angle. It indicates that the largest side is opposite the largest angle.

We can also solve real-world applications using the law of sines.

Example 3

Mr. David is building a garden in his newly built farmhouse. The garden is in the shape of an isosceles triangle. The base of the garden is $50$ feet. The vertex angle of the garden is $40^{\circ }$. Determine the perimeter of the triangular garden to the nearest foot.

Solution:

First of all, we need to create a clearly labeled diagram showing the known measurements and depicting the scenario as shown below.

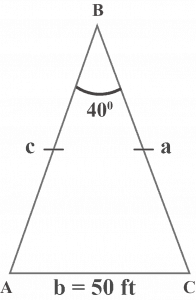
Before solving this problem, here are some of the facts about an isosceles triangle:

• The isosceles triangle contains two equal sides and angles.
• The two equal sides are marked with short lines, as shown in the diagram. So the lengths of sides $a$ and $c$ are equal.
• The two angles opposite the two marked sides are congruent, so angles $A$ and $C$ are congruent.

Looking at the diagram,

$m∠B = 40^{\circ }$

We know that the sum of angles in a triangle is $180^{\circ }$, and angles $A$ and $C$ are equal. Thus,

$m∠A = m∠C = 70^{\circ }$

By using the Law of Sines, we have

$\frac{c}{\sin\:C\:}=\:\frac{b}{\sin\:B}$

$c = b\:\frac{\sin\:C}{\sin\:B}$

$c = (50)\:\frac{\sin\:70^{\circ }}{\sin\:40^{\circ }}$

substitute $\sin 70^{\circ } = 0.93969262$ and $\sin 40^{\circ } = 0.64278761$

$c = 50×\frac{0.93969262}{0.64278761}$

$c = (50) × (1.4619022)$

$c = 73.0951099$

$c ≈ 73$ feet (Rounded to the nearest foot)

Thus, the length of side $c$ is $73$ feet.

as

$a = c$

so

$a = 73$ feet

Since the perimeter of any triangle is the sum of all its three legs. Thus,

Perimeter of the triangular garden $= a + b + c$

                                                           $= 73 + 50 + 73$

                                                           $= 196$ feet

Therefore, the perimeter of the triangular garden is $196$ feet.