Conic Sections – Types, Properties, and Examples

Conic sections are important mathematical concepts – even the ancient Greek mathematicians were fascinated with these three special curves. Conic sections have numerous applications in science and technology, including optics, astronomy, and even architecture.

Conic sections are the result of intersecting the surfaces of a cone (normally, a double cone) and a plane. The three common conic sections are parabola, ellipse, and hyperbola.

In this article, we’ll learn the following concepts about conic sections:

• Understanding how these conic sections were formed.
• Identifying conic sections based on their shapes and equations.
• Learning the common parts shared by the conic sections.
• Exploring the graphs of conic sections and learning how to graph them.

Let’s go ahead and begin by understanding what makes up conic sections.

What is a conic section?                

A conic section is what we call the section formed by the intersection between a right cone and a plane. Hence, we have the word “conic” in its name.

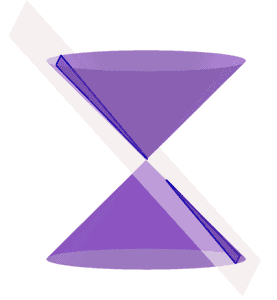
The image above illustrates how a double cone and a plane intersect, forming what we now know as conic sections.

There are three types of conic sections that we’ll be discussing in this article: the parabola, ellipse (its special type would be the circle), and hyperbola. We’ll learn more about these three conic sections in the next section.

How is a conic section obtained?                

As we have mentioned in the previous section, these three conic sections are formed by finding the section intersected by a plane and a double cone.

The figures below show how the three conic sections are formed:

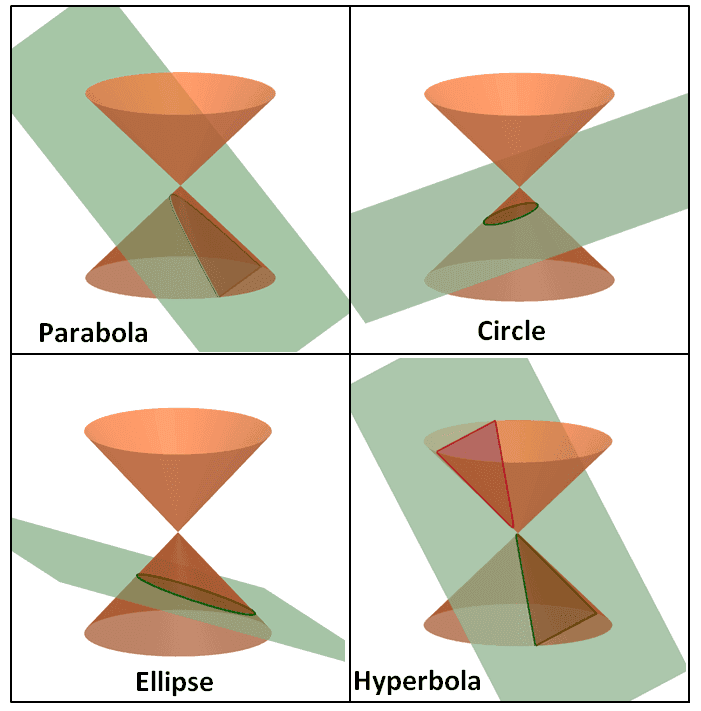
The images above show us how these conic sections or conics are formed when the plane intersects the cone’s vertex.

• If the cone’s plane intersects is parallel to the cone’s slant height, the section formed will be a parabola.
• We can see that the ellipse is the result of a tilted plane intersecting with the double cone. Circles are special types of ellipses and are formed when the cone is intersected by the horizontal plane.
• Hyperbolas are the result of the intersection between the vertical plane and the double cone.

These conics are called degenerate conics, and each of these is expected to contain a point, a line, and intersecting lines.

What are the common parts of the conic section?

Now that we know how to construct the three conic sections, we must learn the parts that they share in common.

Each conic section will look different from the other, but they still share common parts that can be used to identify them, such as their focus, directrix, and eccentricity.

• The focus of a conic refers to the point where the conic section is constructed and is defined.
• The directrix of a conic refers to the line that we use to construct a particular conic section.
• Given a point lying on the conic, the eccentricity reflects the ratio between the distance of the point and the focus and the distance of the point and the directrix.

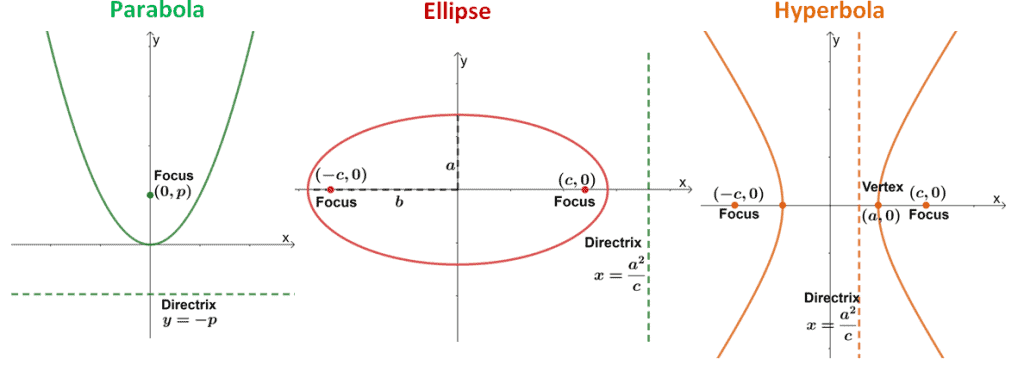
Take a look at these three conics and notice how the directrix and foci (plural of focus) behave in a parabola, ellipse, and hyperbola.

We’ll learn more about each of this conic in the section below, but it helps to know how the focus and directrix would appear in each conic.

How to identify the conic section?

There are three ways to identify a conic section: using its graph’s shape, its eccentricity, or using the coefficients of the equation representing the conic section.

Remember these examples of conic sections as shown below to identify the conic sections, given their graphs easily.

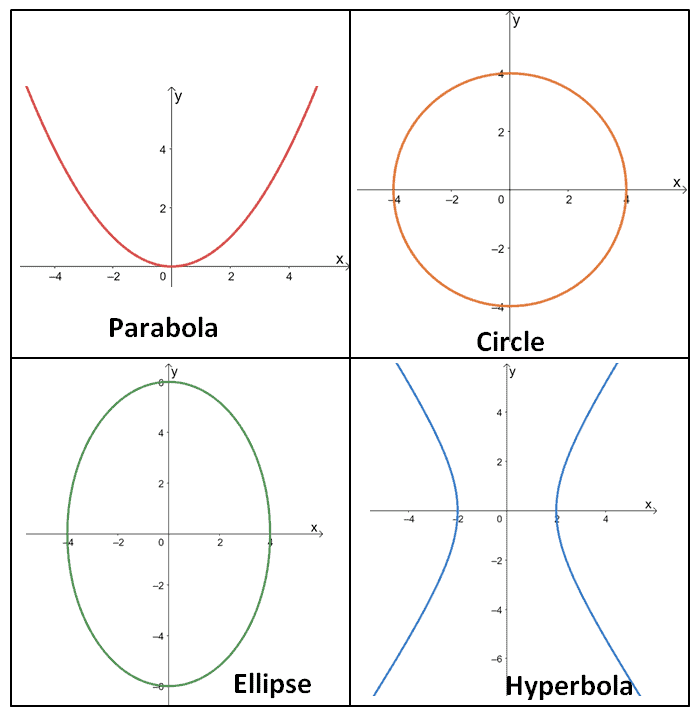
• Parabolas are U-shaped graphs that open either upward or downward.
• Ellipses are oval in shape, with the circle as a special ellipse where the distances vertically and horizontally from the center are equal.
• Hyperbolas’ graphs will always come in pairs – these parabola-like graphs either open vertically or horizontally.

Let’s go ahead and review how we define and identify important parts of these conics and, consequently, learn how to identify these conics when given their graphs or expressions.

Understanding the three special conic sections

If we want to explore the different ways to identify these three special conic sections, it will be best that we have a quick refresher for each conic. Why don’t we begin with the one we’re most familiar with – the parabola?

Parabola

Let’s review our knowledge of the different parts of parabolas and their general forms.

• Parabolas are U-shaped graphs that can either open vertically or horizontally.
• They contain all points where each point will have the same distance between the focus and the directrix.
• This means that the eccentricity of all parabolas is equal to $1$.

Given the parabola’s vertex, the distance between the focus and the vertex will be the same as the vertex’s distance with the directrix. Here are the four possible orientations of a parabola:

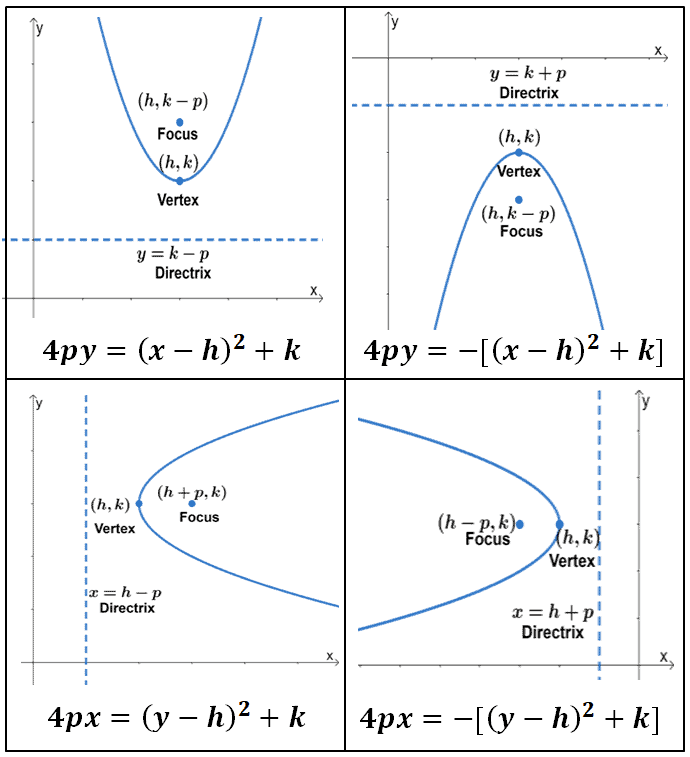
It may not be evident at first since we see at least four variables, but these pointers can help with parabolas.

• The distance, $p$, between the vertex and the focus will be equal to the distance of the vertex and the directrix.
• This means that both the focus and directrix are $p$ units away from the vertex.
• The focus will be placed inside the opening, and the directrix is a dashed line right above, below, to the right, or the left of the vertex.

Still, want to learn more about parabolas? Check out this article we wrote that thoroughly discusses this conic.

Ellipse

Let’s now focus on ellipses – these are oval in shape and contain a set of points wherein the sum of each of their distances from the two foci will always be equal. Here are some other fun facts about ellipses:

• These conics will have two foci and two directrices.
• Unlike circles (which are special cases of ellipses), the longer axis will be the major axis, and the shorter is called the minor axis.
• Ellipses will have eccentricities that are less than $1$.

Here are some variations of the common ellipses that you’ll encounter – the first two will have a center at the origin, while the bottom graphs show ellipses with centers at $(h, k)$.

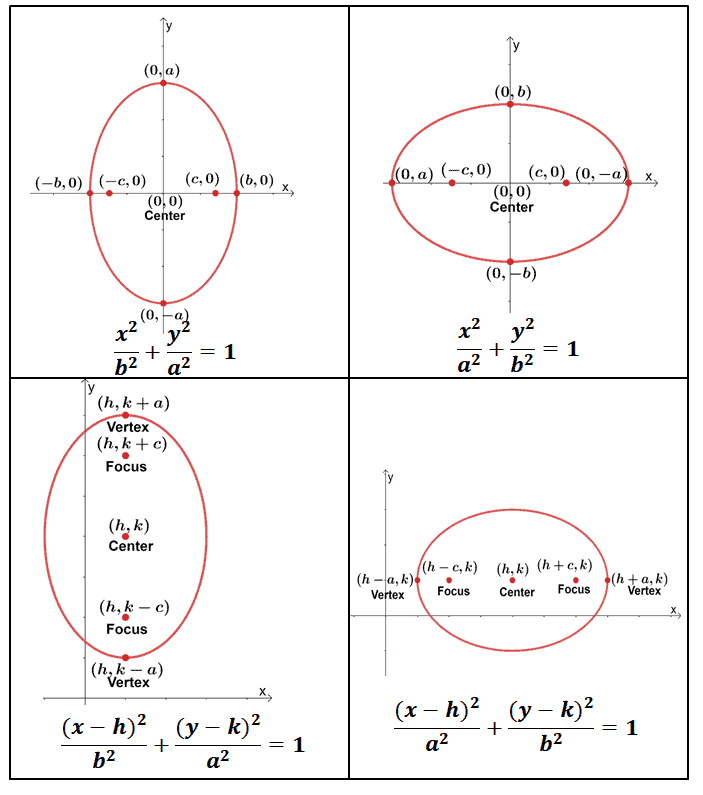
Let’s simplify what these variations mean to better understand ellipses and the foci and directrix’s relationship.

• The ellipses shown above will have a major axis with a length of $2a$ and a major axis that is $2b$ long.
• When the major axis lies vertically, the denominator below $y$ or $y – k$ will contain $a^2$.
• Similarly, when the major axis lies horizontally, the denominator below $x$ or $x – h$ will contain $a^2$ instead.
• We can find the length of the focus using the formula, $c^2 = a^2 – b^2$.

If you want to learn more about ellipses, check out the article we wrote that focuses on ellipses’ special properties.

Hyperbola

Hyperbolas, on the other hand, look like two parabolas facing each other vertically or horizontally. The points contained by a hyperbola’s curve will share the same distance from the foci.

• Hyperbolas will have two foci, and we also rely on the curves’ vertices.
• These conics also reflect two linear asymptotes that are of the form, $y = mx + b$.
• Hyperbolas’ eccentricities will always be greater than 1.

Here are some variations of hyperbolas you’ll encounter, and familiarizing yourselves with these four can help you identify and graph similar hyperbolas easily.

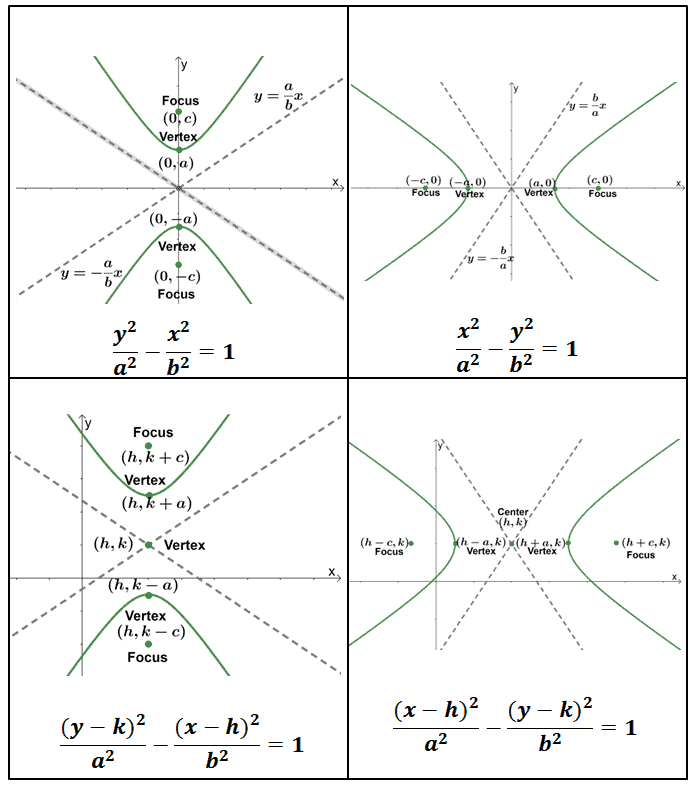
Here are some important pointers to keep in mind about hyperbolas:

• When the curves are parallel to the $x$-axis, the denominator below $x$ or $x – h$ will contain $a^2$.
• When the curves are parallel to the $y$-axis, the denominator below $y$ or $y – k$ will contain $a^2$.
• We can find the length of the focus using the Pythagorean theorem, $c^2 = a^2 +b^2$.
• The asymptotes will rely on the values of $a$ and $b$.

We’ve written a separate article about hyperbolas if you want to learn more about this particular conic.

We’ve discussed each of the three conics briefly. These pointers should help identify a given conic based on their graph, eccentricity, and even properties and elements that they contain.

How to identify conic sections from a general equation?

Let’s say we’re an equation of the form as shown below; there will be two ways for us to identify conic sections by inspecting the coefficients’ values.

\begin{aligned} Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0\end{aligned}

Method 1: Rearrange the Equation

We can rearrange the given equation and see if it can be manipulated to be similar to the standard form of the three conic sections.

• Isolate all the terms on the right-hand side of the equation.
• Complete the perfect square whenever possible.
• After completing the square, make sure the remaining constants are on the left-hand side.
• Compare the new right-hand side equation with the conics’ standard forms and see if they can be categorized.

Method 2: Use the Coefficients of the Equations

• Using the coefficients, particularly $A$, $B$, and $C$, we can immediately identify the conics by finding the value of $B^2 – 4AC$.
• If the result is negative and the conic exists, the conic is an ellipse (or a circle when $a=b$).
• If the result is equal to zero and the conic exists, the conic is a parabola.
• If the result is positive and the conic exists, the conic is a hyperbola.

Now that we know more important conic sections’ properties, we can also identify conic sections based on the equations that represent them. Why don’t we go ahead and take a brief understanding of how we represent these conics on an $xy$-plane.

How to graph conic section?

When graphing conic sections, it’s important to familiarize yourself with each conic’s special properties so that it becomes faster.

Below are some helpful pointers to keep in mind when graphing the three conics, and we’ve each summarized these reminders for each conic.

Graphing a Parabola

Keep in mind that the standard form of parabolas are $y^2 = 4px$ and $x^2 = 4py$, for parabolas that are centered at the origin. The standard form of a parabola when centered at $(h, k)$ is either $(y – k)^2 = 4p(x – h)$ or $(x – h)^2 = 4p(y – k)$.

• To graph a parabola, first identify their centers.
• Plot the focus and directrix $p$ units away from the vertex.
• Take note of the orientation of the parabola (review the refresher section for parabola).
• Use two or more points to connect the curve of the parabola.

Graphing an Ellipse

We’re expecting ellipses to have two foci, a major axis and a minor axis. These elements can be determined by inspecting the equation of the ellipse in standard form.

• As with parabolas, we need to identify the center of the ellipse that’s being graphed first.
• Find the distance of the focus from the vertex of the ellipse by finding the value of $c$.
• Identify if the ellipse’s major axis is parallel with the $x$-axis or the $y$-axis.
• Keep in mind that the vertices are $a$ and $b$ units away from the center.

Make sure to review the refresher section on the ellipse to familiarize yourself with the different variations of an ellipse’s graph.

Graphing a Hyperbola

There can only be two possible options for hyperbolas: the curves are either opening vertically or horizontally.

• It helps to identify which of $x^2$ or $y^2$ is the leading term to determine the orientation of the parabola.
• Locate the center of the hyperbola and calculate the value of $c$ using the denominators.
• Determine the value of $a$ and $c$ to locate the positions of the vertices and foci of the hyperbola.
• Make sure to include the asymptote of the hyperbola as well.

Take a quick look at the variations we created in the earlier section so that you can be guided with how a specific hyperbola will look like.

Summary of the conic section definition and property

How are you holding up so far? It’s okay to take a quick break before trying out the problems shown below.  But for now, why don’t we go ahead and try to summarize what we’ve learned so far about conic sections?

• Conic sections are the result of a cone and plane’s intersection.
• We need to identify the vertex, focus, and center of the conics when graphing them.
• Using the eccentricity (ratio of the fixed point‘s distance from the focus and the distance from the directrix), we can immediately identify the conic sections.

Here’s a chart helping us remember other properties of conic sections that may help us.

Here’s are some more important pointers when we’re given the general form of a conic section equation, $Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0$.

Keep in mind that $A$ and $C$ must not be equal to $0$.

By inspection alone, we can also guess the conic section using the guide questions we’ve prepared below:

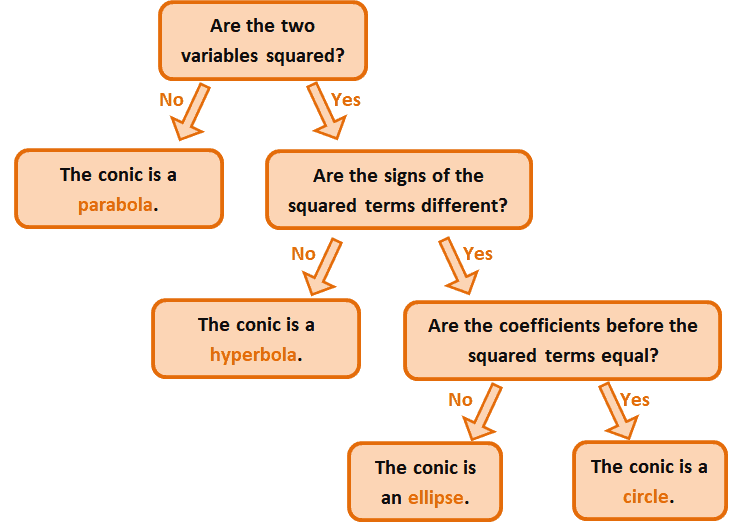
At this point, you’re now ready to try out the problems shown below, and these problems will make you understand the conics’ properties even more.

Example 1

Use your knowledge about ellipses, hyperbolas, and parabolas to fill in the blanks and make the statements true.

a. The foci of the ellipse are $(-6, 8)$ and $(12, 14)$, so the coordinates of ellipse’s center are ______________.
b. The equation $\dfrac{(y – 2)^2}{9} – \dfrac{(x + 1)^2}{4} = 1$ is a _______________ with a center at _______.
c. If the equation of the parabola is $y^2 = 16x$, so the parabola’s focus is ____ units away from the __________.

Solution

The center of the ellipses is found right in the middle of the two given foci, so let’s use the midpoint formula, $\left(\dfrac{x_1 + x_2}{2}, \dfrac{y_1 + y_2}{2}\right)$, to find the center.

a. This means that the ellipse has a center at $\left(\dfrac{-6 + 12}{2}, \dfrac{8 + 14}{2}\right) = (3, 11)$.

One general form that can represent a hyperbola is $\dfrac{(y – k)}{a^2} – \dfrac{(x – h)^2}{b^2} = 1$, where $(h, k)$ is the center.

b. This means that the conic is a hyperbola and a center at $(-1, 2)$.

Given that a parabola centered at the origin has a general form, $y^2 = 4px$, where $p$ represents the distance of the focus from the center. Since $4p = 16$, $p = 4$.

c. This means that the focus is $4$ units away from the center.

Example 2

Identify the conic being represented by the following equations shown below.

a. $9x^2 + 4y^2 – 18x + 16y – 11 = 0$
b. $ x^2 – 4x – 6y = -4$
c. $25y^2 – 9x^2 – 50y – 54x – 119 = 0$

Solution

Let’s use what we know about ellipses, parabolas, and hyperbolas. By inspection, we can see that two squared terms share the same signs starting with the first equation.

This means that the conic is either an ellipse or a circle, but since the coefficients before $x^2$ and $y^2$ are different, the conic can’t be a circle. This means that the first equation represents an ellipse.

We can also confirm this by checking the coefficients of the equation as shown below.

\begin{aligned}9x^2 + 4y^2 – 18x + 16y – 11 &= 0\\\\B&= 0\\A&=9\\C&=4\\0-4(4)(9)&<0 \end{aligned}

Since $B^2 – 4AC$ is less than 0, we can confirm that our investigation’s conclusion is indeed true.

Let’s go ahead and move on to the second equation: only one of the variables is squared, so by inspection, we can see that it is a parabola. Confirming this algebraically, we have the calculations shown below.

\begin{aligned}x^2 – 4x – 6y &= -4\\\\B&= 0\\A&=1\\C&=0\\0-4(1)(0)&=0 \end{aligned}

Since $B^2 – 4AC = 0$, this further confirms what we already know – that the conic section is a parabola.

Moving on to the third equation, we can see that two variables are squared, with each term having opposite signs. This means that the conic being represented by the equation is a hyperbola.

Why don’t we go ahead and inspect the equation’s coefficients?

\begin{aligned}25y^2 – 9x^2 – 50y – 54x – 119 &= 0\\- 9x^2 +25y^2– 50y – 54x – 119&= 0\\B&= 0\\A&=-9\\C&=25\\0-4(-9)(25)&>0 \end{aligned}

Since the result of $B^2 – 4AC$ is greater than $0$, we can further confirm that the equation is indeed a hyperbola.

This example shows that the guide questions we’ve set in the summary are reliable enough for a quick inspection, but you can also double-check this by using a more technical approach.

Example 3

Identify the conic that represents $\dfrac{x^2}{25} + \dfrac{y^2}{9} = 1$. Locate the foci and graph the given conic.

Solution

Recall that the standard form of ellipses centered at the origin, $(0,0)$, is either $\dfrac{x^2}{a^2} + \dfrac{y^2}{b^2}$ or $\dfrac{x^2}{b^2} + \dfrac{y^2}{a^2}$.

This means that the conic we’re trying to graph is an ellipse with a center at the origin.

Since it’s the $x^2$ that has a larger denominator, the ellipse has a major axis parallel with the $x$-axis. Graphically, this means that the ellipse is longer horizontally.

We have $a^2 = 25$ and $b^2 = 9$, so $c^2 = 25 – 16 = 9$ and consequently, $c = 3$. With all these values, we now have an idea on how the ellipse would appear on the $xy$-plane.

• Since $a^2 = 25$, the vertices of the ellipse along the $x$-axis would be $a = 5$ units away from the origin.
• Similarly, with $b^2 = 9$, the vertices along the $y$-axis are expected to be $b = 3$ units away from the origin.
• Using $c = 16$, we know that the foci will be $4$ units away from the origin, $(0, 0)$.

Let’s start by plotting these values on the coordinate system.

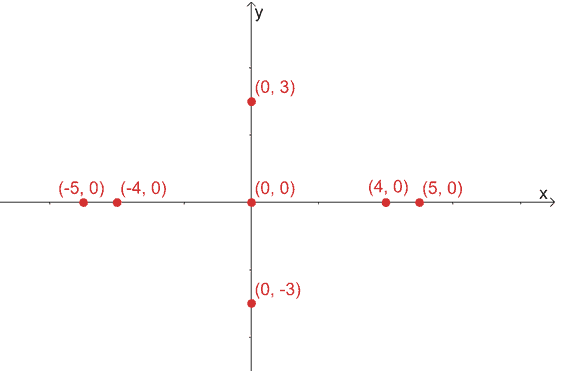
This confirms that the ellipse has a major axis of $2(5) = 10$ units wide and a minor axis of $2(3) = 6$ units long. Let’s connect the vertices to form the graph of the ellipse.

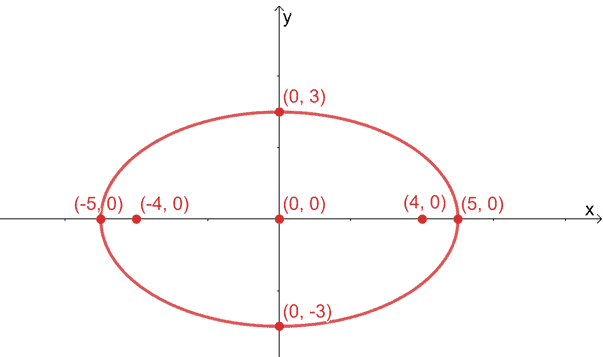
Hence, we have the graph of $\dfrac{x^2}{25} + \dfrac{y^2}{9} = 1$ as shown above. We can also see that its foci are $(-4, 0)$ and $(4, 0)$.

Example 4

Identify the conic that represents $2x^2 – 2y^2 – 4x – 8y – 8 = 0$. Graph the given conic and include the foci as well as the asymptotes (if any).

Solution

Let’s rewrite the given equation in standard form, but first, let’s guess the type of conic this equation represents.

The equation has two squared terms, and these terms have opposite signs, so we’re expecting the conic to be a hyperbola. Now, let’s begin rewriting this equation in standard form.

• Divide both sides of the equation by $2$.
• Group the terms sharing common variables and move the constant on the right-hand side.
• Use the algebraic property, $(a \pm b)^2 = a^2 \pm 2ab + b^2$, to complete the square and express the trinomials as perfect squares.

\begin{aligned}2x^2 – 2y^2 – 4x – 8y – 8 &= 0\\ x^2 – y^2 – 2x – 4y – 4 &=0\\ (x^2 – 2x) -(y^2 + 4y)&= 4\\(x^2 – 2x + 1) – (y^2 + 4y + 4)&= 4 + 1 – 4\\(x – 1)^2 – (y + 2)^2 &= 1\\\dfrac{(x – 1)^2}{1} – \dfrac{(y + 2)^2}{1} & = 1\end{aligned}

Now that the equation is in standard form, $\dfrac{(x – 1)^2}{1} – \dfrac{(y – 2)^2}{1}  = 1$, we can confirm that the conic is in fact a hyperbola centered at $(1, 2)$.

This means that $a = 1$ and $b = 1$. We can also find the distance of the foci from the center using the formula, $c^2 = a^2 + b^2$. Hence, we have $c = \sqrt{2}$.

• Plot the center, $(1, 1)$, of the hyperbola to act as a guide when graphing the hyperbola’s essential components.
• Graph the vertices of the hyperbola that are $a = 1$ unit away from the center.
• Graph the foci of the hyperbola that are $c = \sqrt{2} \approx 1.414$ away from the center.

The asymptotes of the hyperbola with an unshifted center at the origin are $y = pm \dfrac{1}{1}x = \pm x$. When the center is (1,-2), the equations of the asymptote will be

\begin{aligned} y + 2 &= \pm (x – 1)\\\\y + 2 &= x – 1\\y&= x – 3\\y+2 &= – x + 1\\y&= – x – 1\end{aligned}.

Let’s include the asymptotes of the hyperbola on the graph.

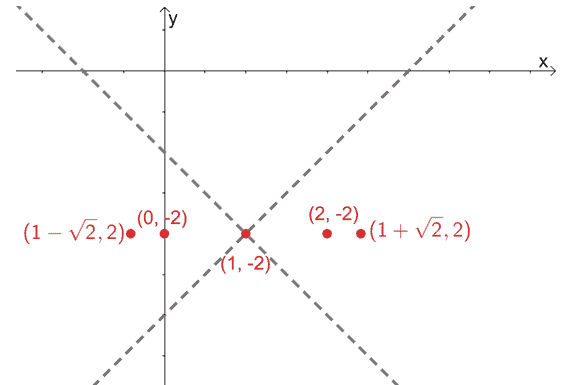
Using the vertices, foci, and the hyperbola’s asymptotes, we can now graph the curve containing all the points that satisfy the equation, $2x^2 – 2y^2 – 4x – 8y – 8 = 0$.

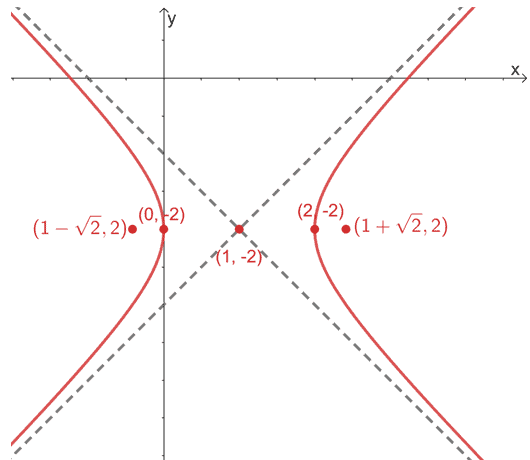
Example 5

What is the standard form of the equation representing the parabola with a focus of $(0, 15)$ and a directrix of $y = -15$?

Solution

We can do a quick sketch of the parabola using these components: a focus of $(0, 15)$ and a directrix of $y = -15$.

• Since the focus is lying along the $y$-axis, we expect the graph to open either upward or downward.
• We also know that the parabola will then have a vertex at the origin, $(0, 0)$
• Since the directrix is negative, the parabola is opening upward.

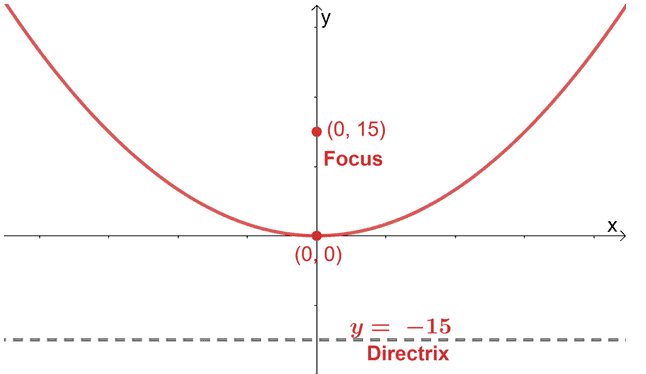
For this type of parabola, its general form will be $y^2 = 4px$, with $p$ as the focus and directrix’s fixed distance from the parabola’s center.

We can see that the focus and the directrix are each $15$ units away, so $p = 15$. This means that the equation representing this parabola is as shown below.

\begin{aligned}y^2 &= 4px \\y^2 &= 4(15)x\\y^2 &= 60x\end{aligned}

Hence, the equation of the parabola that has a focus of $(0, 15)$ and a diretrix of $y = -15$ is $y^2 = 60x$.