Difference of Squares – Explanation & Examples

A quadratic equation is a second degree polynomial usually in the form of f(x) = ax² + bx + c where a, b, c, ∈ R, and a ≠ 0. The term ‘a’ is referred to as the leading coefficient, while ‘c’ is the absolute term of f (x). Every quadratic equation has two values of the unknown variable, usually known as the roots of the equation (α, β).

What is Difference of Squares?

The difference of two squares is a theorem that tells us if a quadratic equation can be written as a product of two binomials, in which one shows the difference of the square roots and the other shows the sum of the square roots.

One thing to note about this theorem is that it does not apply to the SUM of squares.

Difference of Squares Formula

The difference of square formula is an algebraic form of the equation used to express the differences between two square values. A difference of square is expressed in the form:

a² – b², where both the first and last term is perfect squares. Factoring the difference of the two squares gives:

a² – b² = (a + b) (a – b)

This is true because, (a + b) (a – b) = a² – ab + ab – b² = a² – b²

How to Factor Difference of Squares?

In this section, we will learn how to factorize algebraic expressions using the difference of square formula. To factor a difference of squares, the following steps are undertaken:

• Check if the terms have the greatest common factor (GCF) and factor it out. Remember to include the GCF in your final answer.
• Determine the numbers that will produce the same results and apply the formula: a²– b² = (a + b) (a – b) or (a – b) (a + b)
• Check whether you can factor the remaining terms any further.

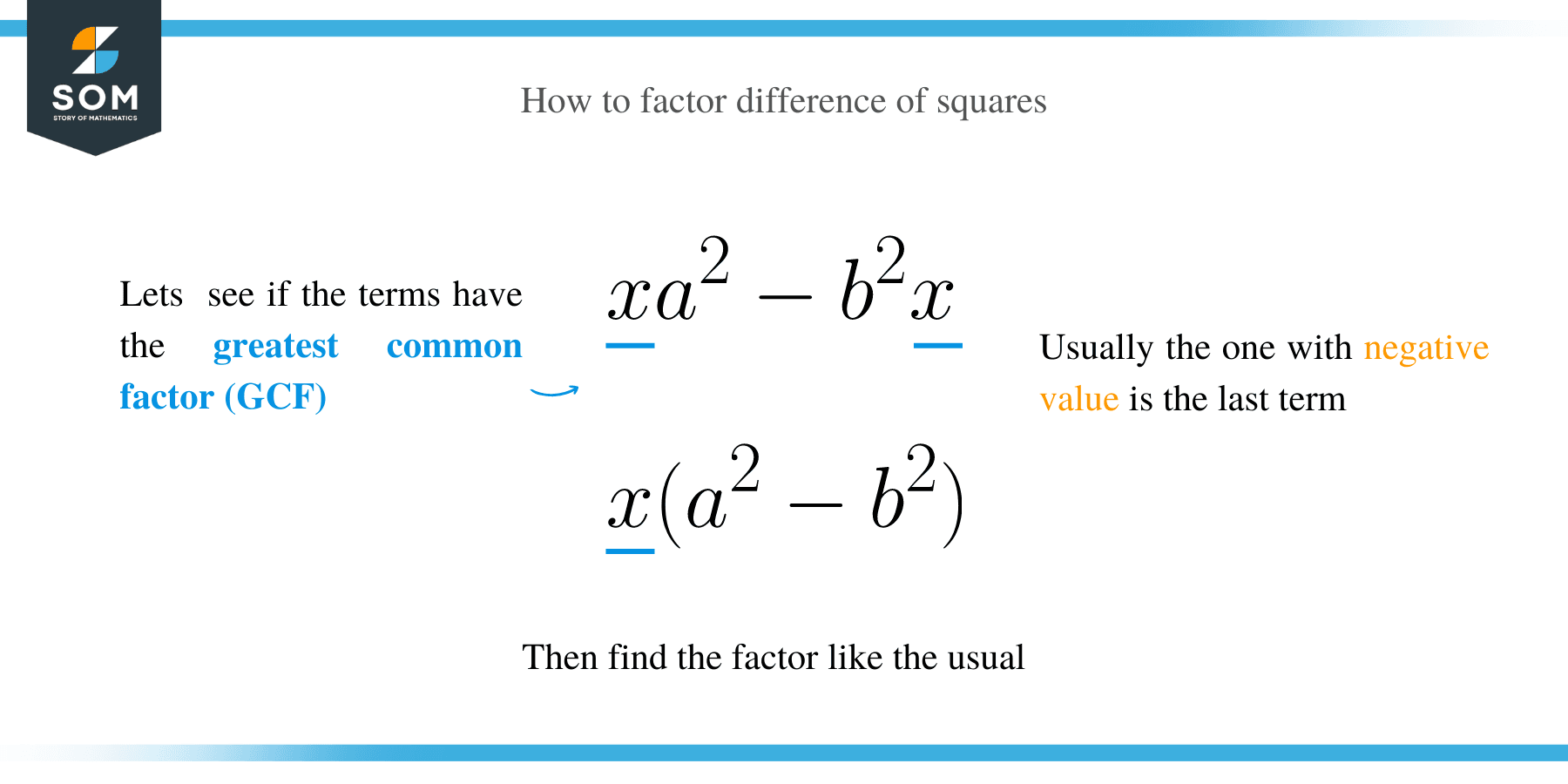Let’s solve a few examples by applying these steps.

Example 1

Factor 64 – x²

Solution

Since we know the square of 8 is 64, then we can rewrite the expression as;
64 – x² = (8)² – x²
Now, apply the formula a² – b² = (a + b) (a – b) to factorize expression;
= (8 + x) (8 – x).

Example 2

Factorize
x ² −16

Solution

Since x²−16 = (x) ²− (4)², therefore apply the difference square formula a² – b² = (a + b) (a – b), where a and b in this case are x and 4 respectively.

Therefore, x² – 4² = (x + 4) (x – 4)

Example 3

Factor 3a² – 27b²

Solution

Since 3 is GCF of the terms, we factor it out.
3a² – 27b² = 3(a² – 9b²)
=3[(a)² – (3b)²]
Now apply a² – b² = (a + b) (a – b) to get;
= 3(a + 3b) (a – 3b)

Example 4

Factor x³ – 25x
Solution

Since the GCF = x, factor it out;
x³ – 25x = x (x² – 25)
= x (x² – 5²)
Apply the formula a² – b² = (a + b) (a – b) to get;
= x (x + 5) (x – 5).

Example 5

Factor the expression (x – 2)² – (x – 3)²

Solution

In this problem a = (x – 2) and b = (x – 3)

We now apply a² – b² = (a + b) (a – b)

= [(x – 2) + (x – 3)] [(x – 2) – (x – 3)]

= [x – 2 + x – 3] [x – 2 – x + 3]

Combine the like terms and simplify the expressions;

[x – 2 + x – 3] [x – 2 – x + 3] = > [2x – 5] [1]

= [2x – 5]

Example 6

Factor the expression 25(x + y)² – 36(x – 2y)².

Solution

Rewrite the expression in the form a² – b².

25(x + y)² – 36(x – 2y)² => {5(x + y)}² – {6(x – 2y)}²
Apply the formula a² – b² = (a + b) (a – b) to get,

= [5(x + y) + 6(x – 2y)] [5(x + y) – 6(x – 2y)]

= [5x + 5y + 6x – 12y] [5x + 5y – 6x + 12y]

Collect like terms and simplify;

= (11x – 7y) (17y – x).

Example 7

Factor 2x²– 32.

Solution

Factor out the GCF;
2x²– 32 => 2(x²– 16)
= 2(x² – 4²)

Applying the difference squares formula, we get;
= 2(x + 4) (x – 4)

Example 8

Factor 9x⁶ – y⁸

Solution

First, rewrite 9x⁶ – y⁸ in the form a² – b².

9x⁶ – y⁸ => (3x³)² – (y⁴)²

Apply a² – b² = (a + b) (a – b) to get;

= (3x³ – y⁴) (3x³ + y⁴)

Example 9

Factor the expression 81a² – (b – c)²

Solution

Rewrite 81a² – (b – c)² as a² – b²
= (9a)² – (b – c)²
By applying the formula of a² – b² = (a + b) (a – b) we get,
= [9a + (b – c)] [9a – (b – c)]
= [9a + b – c] [9a – b + c ]

Example 10

Factor 4x²– 25

Solution

= (2x)²– (5)²
= (2x + 5) (2x – 5