- Home
- >
- Factoring Trinomial – Method & Examples
JUMP TO TOPIC
Factoring Trinomial – Method & Examples
 Proficiency in algebra is a key tool in understanding and mastering mathematics. For those aspiring to advance their level in studying Algebra, factoring is a fundamental skill required for solving complex problems involving polynomials.
Proficiency in algebra is a key tool in understanding and mastering mathematics. For those aspiring to advance their level in studying Algebra, factoring is a fundamental skill required for solving complex problems involving polynomials.
Factoring is employed at every algebra level for solving polynomials, graphing functions, and simplifying complex expressions.
Generally, factoring is the inverse operation of expanding an expression.
For example, 3(x − 2) is a factored form of 3x − 6, and (x − 1) (x + 6) is a factored form of x2 + 5x − 6. While expanding is comparatively a straightforward process, factoring is a bit challenging, and therefore a student ought to practice various types of factorization to gain proficiency in applying them.
If there is any lesson in Algebra that many students find perplexing is the topic of factoring trinomials.
This article will guide you step by step in understanding how to solve problems involving the factoring of trinomials. Therefore, the illusion of this topic being the hardest will be your story of the past.
You will learn how to factor all kinds of trinomials, including those with a leading coefficient of 1 and those with a leading coefficient not equal to 1.
Before we get started, it is useful to recall the following terms:
Factors
A factor is a number that divides another given number without leaving a remainder. Every number has a factor that is less than or equal to the number itself.
For example, the factors of the number 12 are 1, 2, 3, 4, 6, and 12 themselves. We can conclude that all numbers have a factor of 1, and every number is a factor of itself.
Factoring
Before the invention of electronic and graphing calculators, factoring was the most reliable method of finding the roots of polynomial equations.
Though quadratic equations gave solutions that were more direct as compared to complex equations, it was only limited for
second-degree polynomials.
Factoring allows us to rewrite a polynomial into simpler factors, and by equating these factors to zero, we can determine the solutions of any polynomial equation.
There are several methods of factoring polynomials. This article will focus on how to factor different types of trinomials, such as trinomials with a leading coefficient of 1 and those with a leading coefficient not equal to 1.
Before we get started, we must familiarize ourselves with the following terms.
Common factors
The common factor is defined as a number that can be divided into two or more different numbers without leaving a remainder.
For example, the common factors of the numbers 60, 90, and 150 are; 1, 2, 3,5, 6,10, 15, and 30.
The Greatest Common Factor (GCF)
The Greatest Common Factor of numbers is the largest value of factors of the given numbers. For example, given the common factors of 60, 90, and 150 are; 1, 2, 3,5, 6,10, 15, and 30, and therefore the greatest common factor is 30.
The GCF. for a trinomial is the largest monomial that divides each term of the trinomial. For example, to find the GCF of an expression 6x4 – 12x3 + 4x2, we apply the following steps:
- Break down each term of the trinomial into prime factors.
(2* 3 * x * x* x * x) – (2 * 2* 3 * x * x * x) + (2 * 2 * x * x)
- Look for factors that appear in every single term above.
You can encircle or color the factors as:
(2* 3 * x * x* x * x) – (2 * 2* 3 * x * x * x) + (2 * 2 * x * x)
Therefore, the GCF of 6x4 – 12x3 + 4x2 is 2x2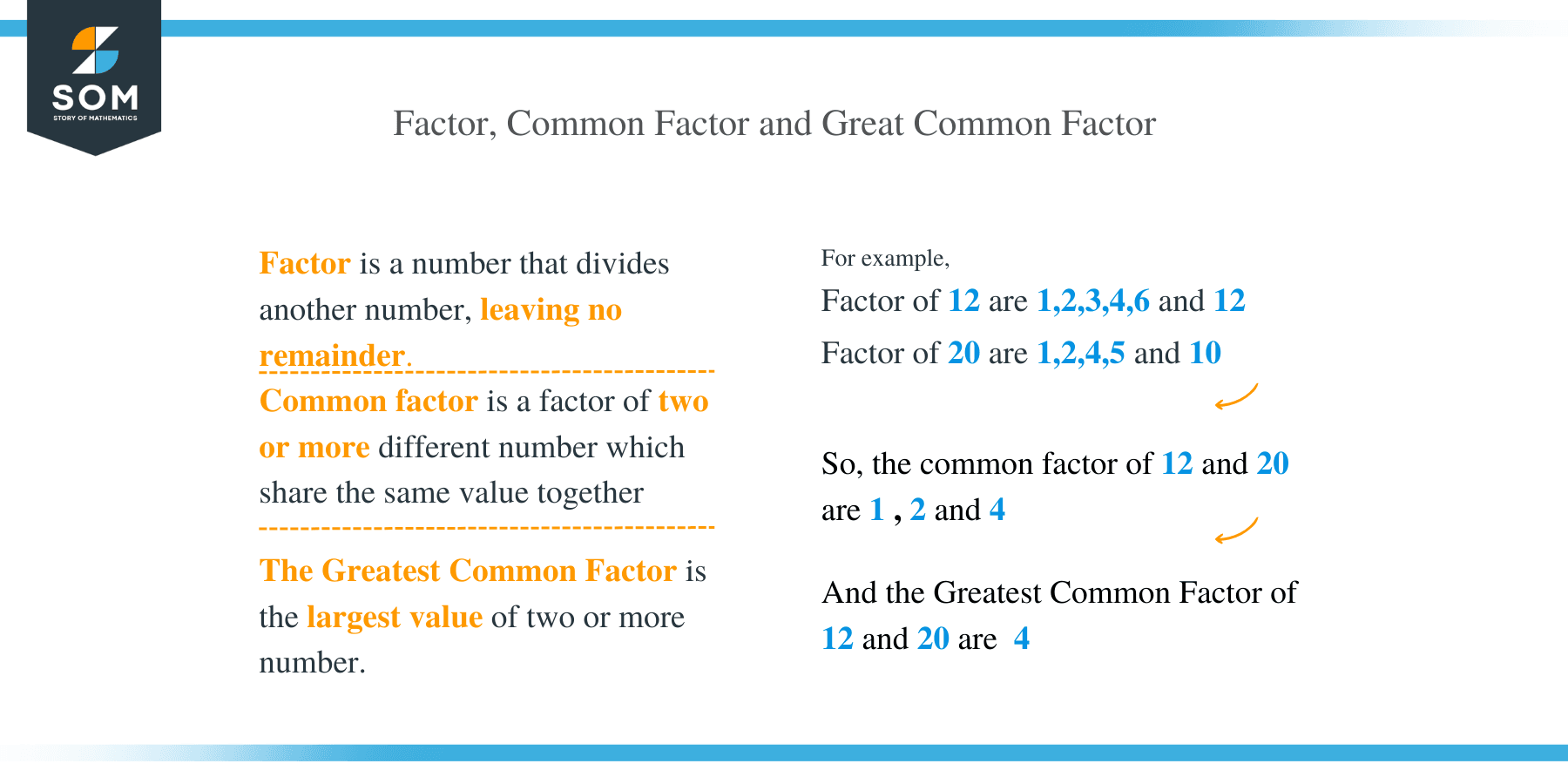
Polynomial
A polynomial is an algebraic expression containing more than two terms, such as variables and numbers, usually combined by addition or subtraction operations.
Examples of polynomials are 2x + 3, 3xy – 4y, x² − 4x + 7 and 3x + 4xy – 5y.
Trinomial
A trinomial is an algebraic equation composed of three terms and is normally of the form ax2 + bx + c = 0, where a, b and c are numerical coefficients. The number “a” is called the leading coefficient and is not equal to zero (a≠0).
For instance, x² − 4x + 7 and 3x + 4xy – 5y are examples of trinomials. On the other hand, a binomial is an algebraic expression consisting of two terms. Examples of binomial expression include; x + 4, 5 – 2x, y + 2 etc.
To factor a trinomial is to decompose an equation into the product of two or more binomials. This means that we will rewrite the trinomial in the form (x + m) (x + n).
Your task is to determine the value of m and n. In other words, we can say that factoring a trinomial is the reverse process of the foil method.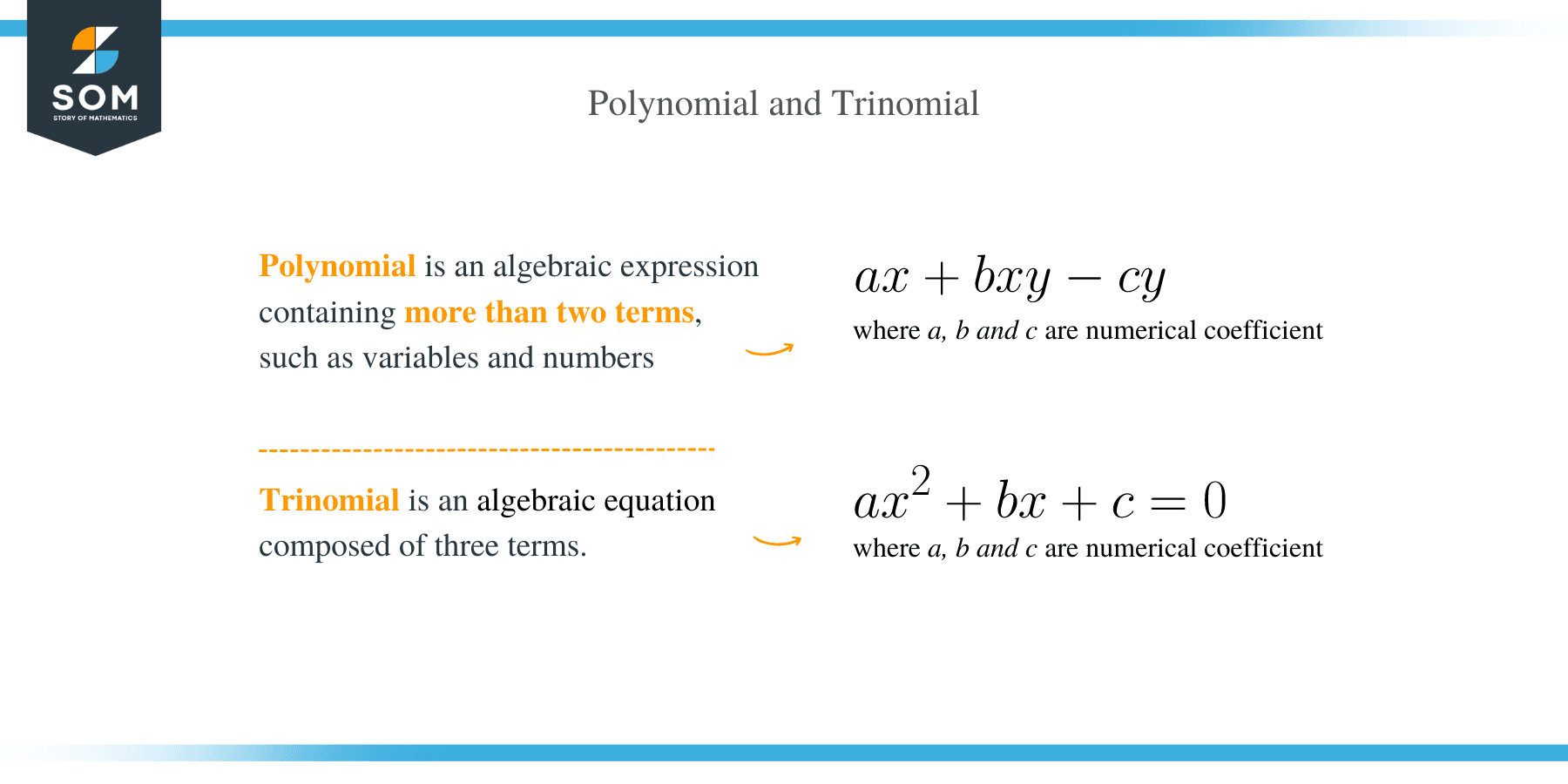
How to factor trinomials with a leading coefficient of 1
Let’s walk through the following steps to factor x2 + 7x + 12:
- Comparing x2 + 7x + 12 with the standard form of ax2 + bx + c, we get, a = 1, b = 7, and c = 12
- Find the paired factors of c such that their sum is equal to b. The pair factor of 12 are (1, 12), (2, 6), and (3, 4). Therefore, the suitable pair is 3 and 4.
- In separate brackets, add each number of the pair to x to get (x + 3) and (x + 4).
- Write the two binomials side by side to get the factored result as;
(x + 3) (x + 4).
How to factor trinomials with GCF?
To factor a trinomial with the leading coefficient not equal to 1, we apply the concept of the greatest common factor (GCF) as shown in the steps below:
- If the trinomial is not in the correct order, rewrite it in descending order, from highest to lowest power.
- Factor out the GCF, and remember to include it in your final answer.
- Find the product of the leading coefficient “a” and the constant “c.”
- List all the factors of the product of a and c from step 3 above. Identify the combination that will add up to get the number next to x.
- Rewrite the original equation by replacing the term “bx” with the chosen factors from step 4.
- Factor the equation by grouping.
To summarize this lesson, we can factor a trinomial of the form ax2 +bx + c by applying any of these five formulas:
- a2 + 2ab + b2 = (a + b)2 = (a + b) (a + b)
- a2 – 2ab + b2 = (a − b)2 = (a − b) (a − b)
- a2 – b2 = (a + b) (a − b)
- a3 + b3 = (a + b) (a2 – ab + b2)
- a3 – b3 = (a – b) (a2 + ab + b2)
Let’s now factor a couple of examples of trinomial equations.
Example 1
Factor 6x2 + x – 2
Solution
The GCF =1, therefore it is of no help.
Multiply the leading coefficient a and the constant c.
⟹ 6 * -2 = -12
List all factors of 12 and identify a pair that has a product of -12 and a sum of 1.
⟹ – 3 * 4
⟹ -3 + 4 = 1
Now, rewrite the original equation by replacing the term “bx” with the chosen factors
⟹ 6x2 – 3x + 4x – 2
Factor the expression by grouping.
⟹ 3x (2x – 1) + 2(2x – 1)
⟹ (3x + 2) (2x – 1)
Example 2
Factor 2x2 – 5x – 12.
Solution
2x2 – 5x – 12
= 2x2 + 3x – 8x – 12
= x (2x + 3) – 4(2x + 3)
= (2x + 3) (x – 4)
Example 3
Factor 6x2 -4x -16
Solution
The GCF of 6, 4 and 16 is 2.
Factor out the GCF.
6x2 – 4x – 16 ⟹ 2(3x2 – 2x – 8)
Multiply the leading coefficient “a” and the constant “c.”
⟹ 6 * -8 = – 24
Identify the paired factors of 24 with the sum of -2. In this case, 4 and -6 are the factors.
⟹ 4 + -6 = -2
Rewrite the equation by replacing the term “bx” with the chosen factors.
2(3x2 – 2x – 8) ⟹ 2(3x2 + 4x – 6x – 8)
Factor by grouping and don’t forget to include the GCF in your final answer.
⟹ 2[x (3x + 4) – 2(3x + 4)]
⟹ 2[(x – 2) (3x + 4)]
Example 4
Factor 3x3 – 3x2 – 90x.
Solution
Since the GCF= 3x, factor it out;
3x3 – 3x2 – 90x ⟹3x (x2 – x – 30)
Find a pair of factors whose product is −30 and sum is −1.
⟹- 6 * 5 =-30
⟹ −6 + 5 = -1
Rewrite the equation by replacing the term “bx” with the chosen factors.
⟹ 3x [(x2 – 6x) + (5x – 30)]
Factor the equation;
⟹ 3x [(x (x – 6) + 5(x – 6)]
= 3x (x – 6) (x + 5)
Example 5
Factor 6z2 + 11z + 4.
Solution
6z2 + 11z + 4 ⟹ 6z2 + 3z + 8z + 4
⟹ (6z2 + 3z) + (8z + 4)
⟹ 3z (2z + 1) + 4(2z + 1)
= (2z + 1) (3z + 4)
