HENRI POINCARÉ AND THE CHAOS THEORY
Biography
 |
Henri Poincaré (1854-1912) |
Paris was a great centre for world mathematics towards the end of the 19th Century, and Henri Poincaré was one of its leading lights in almost all fields – geometry, algebra, analysis – for which he is sometimes called the “Last Universalist”.
Even as a youth at the Lycée in Nancy, he showed himself to be a polymath, and he proved to be one of the top students in every topic he studied. He continued to excel after he entered the École Polytechnique to study mathematics in 1873, and, for his doctoral thesis, he devised a new way of studying the properties of differential equations. Beginning in 1881, he taught at the Sorbonne in Paris, where he would spend the rest of his illustrious career. He was elected to the French Academy of Sciences at the young age of 32, became its president in 1906, and was elected to the Académie française in 1909.
Poincaré deliberately cultivated a work habit that has been compared to a bee flying from flower to flower. He observed a strict work regime of 2 hours of work in the morning and two hours in the early evening, with the intervening time left for his subconscious to carry on working on the problem in the hope of a flash of inspiration. He was a great believer in intuition, and claimed that “it is by logic that we prove, but by intuition that we discover“.
It was one such flash of inspiration that earned Poincaré a generous prize from the King of Sweden in 1887 for his partial solution to the “three-body problem”, a problem that had defeated mathematicians of the stature of Euler, Lagrange and Laplace. Newton had long ago proved that the paths of two planets orbiting around each other would remain stable, but even the addition of just one more orbiting body to this already simplified solar system resulted in the involvement of as many as 18 different variables (such as position, velocity in each direction, etc), making it mathematically too complex to predict or disprove a stable orbit.
Poincaré’s Analysis of the Three Body Problem
Poincaré’s solution to the “three-body problem”, using a series of approximations of the orbits, although admittedly only a partial solution, was sophisticated enough to win him the prize.
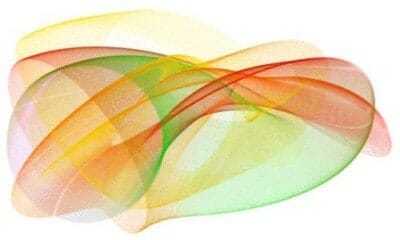 |
Computer representation of the paths generated by Poincaré’s analysis of the three body problem |
But he soon realized that he had actually made a mistake, and that his simplifications did not indicate a stable orbit after all. In fact, he realized that even a very small change in his initial conditions would lead to vastly different orbits. This serendipitous discovery, born from a mistake, led indirectly to what we now know as chaos theory, a burgeoning field of mathematics most familiar to the general public from the common example of the flap of a butterfly’s wings leading to a tornado on the other side of the world. It was the first indication that three is the minimum threshold for chaotic behavior.
Paradoxically, owning up to his mistake only served to enhance Poincaré’s reputation, if anything, and he continued to produce a wide range of work throughout his life, as well as several popular books extolling the importance of mathematics.
Poincaré also developed the science of topology, which Leonhard Euler had heralded with his solution to the famous Seven Bridges of Königsberg problem. Topology is a kind of geometry which involves one-to-one correspondence of space. It is sometimes referred to as “bendy geometry” or “rubber sheet geometry” because, in topology, two shapes are the same if one can be bent or morphed into the other without cutting it. For example, a banana and a football are topologically equivalent, as are a donut (with its hole in the middle) and a teacup (with its handle); but a football and a donut, are topologically different because there is no way to morph one into the other. In the same way, a traditional pretzel, with its two holes is topological different from all of these examples.
Poincaré conjecture: 2-dimensional representation of the 3-dimensional problem
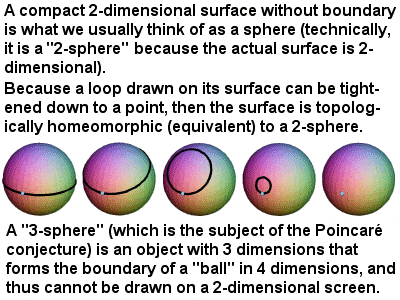 |
A 2-dimensional representation of the 3-dimensional problem in the Poincaré conjecture |
In the late 19th Century, Poincaré described all the possible 2-dimensional topological surfaces but, faced with the challenge of describing the shape of our 3-dimensional universe, he came up with the famous Poincaré conjecture, which became one of the most important open questions in mathematics for almost a century.
The conjecture looks at a space that, locally, looks like ordinary 3-dimensional space but is connected, finite in size and lacks any boundary (technically known as a closed 3-manifold or 3-sphere). It asserts that, if a loop in that space can be continuously tightened to a point, in the same way as a loop drawn on a 2-dimensional sphere can, then the space is just a three-dimensional sphere. The problem remained unsolved until 2002, when an extremely complex solution was provided by the eccentric and reclusive Russian mathematician Grigori Perelman, involving the ways in which 3-dimensional shapes can be “wrapped up” in higher dimensions.
Poincaré’s work in theoretical physics was also of great significance, and his symmetrical presentation of the Lorentz transformations in 1905 was an important and necessary step in the formulation of Einstein’s theory of special relativity (some even hold that Poincaré and Lorentz were the true discoverers of relativity). He also made important contribution in a whole host of other areas of physics including fluid mechanics, optics, electricity, telegraphy, capillarity, elasticity, thermodynamics, potential theory, quantum theory and cosmology.
<< Back to Cantor | Forward to 20th century Mathematics >> |
