JUMP TO TOPIC
Factors of 125: Prime Factorization, Methods, Tree, and Examples
The numbers that may completely divide 125 are referred to as Factors of 125, as are the numbers whose product, when two numbers are multiplied together, is 125. A number is regarded as a factor if it divides 125 with 0 as the remainder.

Figure 1 – All possible Factors of 125
Please make a list of all the integers that are less than or equal to the number to determine its components. For example, the range of 1 to 25 will include the number 125. Splitting each of them will make it obvious the solution to this issue.
A notable point regarding factors is that two is the factor of all integers. Thus, the factors of a number can be determined using division and multiplication. However, several methods can be used to identify an integer’s factors.
There are easier ways to determine a number’s factors. The quotient and the divisor are considered factors of the given number once the remainder equals zero, which can be achieved by simply reducing the number until the remainder equals zero.
Let’s examine one of these situations as a sample. 125/5 comes out to 25, which is the outcome. The outcome is that both the divisor and the solution are considered factors.
This article will show you how to determine the factors of 125, as well as factor pairs, a factor tree, straightforward solutions, and more. In other words, after reading this article and learning how to find the factors of 125, figuring out the factors of any different number will be a breeze for you!
What Are the Factors of 125?
The factors of the perfect cube number 125 are 1, 5, 25, and 125. 125 is a composite number, which includes more than two factors. There are a total of four factors of 125.
The factors of 125 are the values that divide the number 125 precisely without leaving a remainder. The pair factors of 125 are the numbers multiplied by each other in pairs to get the number 125, such as (1, 125) and (5, 25).
How To Calculate the Factors of 125?
To calculate the factors of 125, Multiplication and division are the two methods that can be used.
In order to discover the factors by dividing, let’s first go over how to do that. See if you can find any numbers less than or equal to 125. After that, multiply each number by 125. The division factors of 125 produce a remainder of 0.
Take a look at the sample below to understand this idea better:
We obtain 25 by dividing 125 by 5, which is the smallest multiple of 125 other than one. As a result, the factors of the number 125 are 5 and 25.
\[ \frac{125}{5} = 25 \]
As the quotient is a whole integer and has no remainder, this demonstrates that the divisor and quotient are both factors of 125. (5, 25)
The following is a list of every factor of 125:
\[ \frac{125}{1} = 125 \]
\[ \frac{125}{5} = 25 \]
\[ \frac{125}{25} = 5 \]
\[ \frac{125}{125} = 1 \]
Thus, these are the factors of 125, i.e., 1, 5, 25, and 125.
So let’s start by multiplying to discover the factors of 125. In all likely circumstances, consider 125 to be the product of two whole numbers. In each of these products, all the numbers are the factors of 125. Examine the following examples:
1 x 125 = 125
5 x 25 = 125
25 x 5 = 125
125 x 1 = 125
Hence, listed above are the factors of 125.
Factors of 125 by Prime Factorization
The prime factorization approach involves identifying the prime factors that can multiply with one another to produce the given number as a product. This method can express a specific number as the product of its prime factors.
In other words, it is a method for calculating or displaying a given integer as the sum of prime integers. One and the number are the only two factors that make up a prime number.
The number 125 should have prime factors because it is a composite number. Now let’s learn how to recognize the main factors. First, divide 125 by the smallest prime factor—use let’s two as an example—to get a result.
\[ \frac{125}{1} = 125 \]
An answer is a fractional number, not a whole number, so it is not a factor. Moving on, we check other numbers
\[ \frac{125}{3} = 41.66 \]
\[ \frac{125}{4} = 31.25 \]
The decimal numbers show that 3 and 4 are also not the factors of 125.
The following are the prime numbers of 125:
\[ \frac{125}{5} = 25 \]
\[ \frac{25}{5} = 5 \]
\[ \frac{5}{5} = 1 \]
We were given the number 1 when the division procedure was complete. Consequently, it keeps us from continuing. Thus, the notations 5x5x5 are used to indicate the prime factors of 125. In this case, 5 is the prime number.
Below is the diagram of the prime factorization of 125:
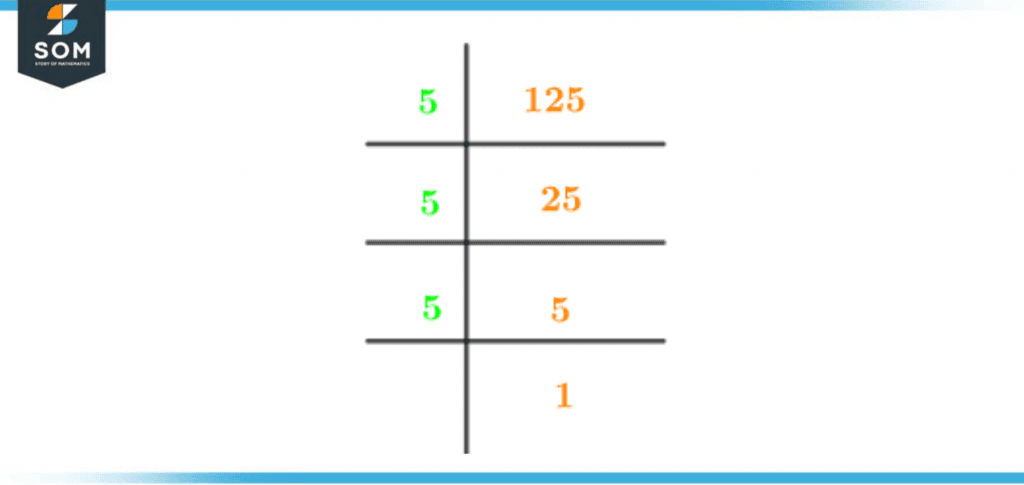
Figure 2 – Prime Factorization of 125
Factor Tree of 125
A “Factor tree” is one of the numerous ways to graphically depict a number’s prime factors, even though a number’s factors can be stated in various ways. The root of the factor tree, from which branches grow to the prime number, is the actual number. Henceforth, it is a factor.
Therefore, 125 has a distinct prime factor of 5 according to prime factorization. Thus, five should be the last number to appear on the factor tree.
The diagram of the factor tree of 125 is as follows:
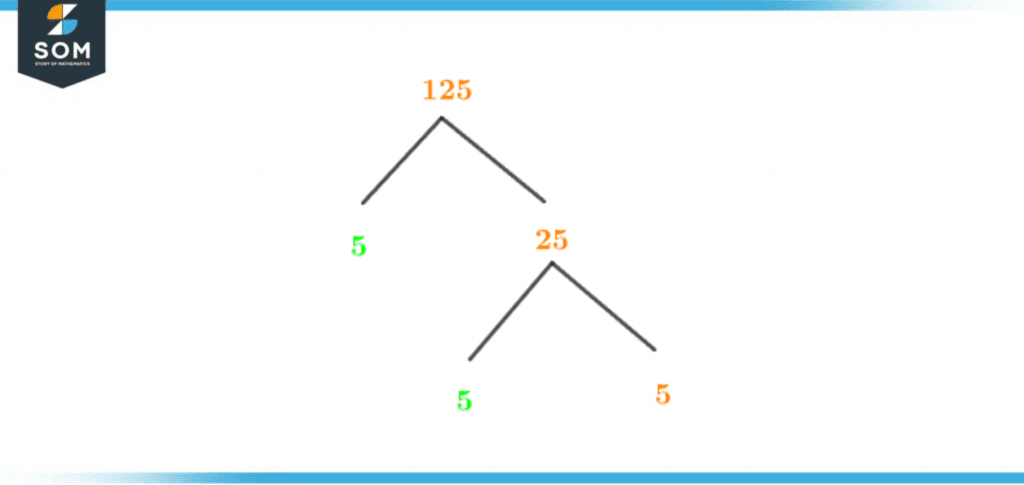
Figure 3 – Factor Tree of 125
The highly entertaining and fascinating facts regarding the number 125 are as follows:
- 125 is a Friedman number in base 10, just like many other powers of 5. Moreover, a close triplet of perfect powers has 125 in its center.
- The US Air Force’s 125th Fighter Wing of the Air National Guard is located at Jacksonville International Airport in Florida.
- Also, the cube of 5 results in 125.
- The Honda XRM 125 motorcycle is one example of a 125cc engine restricted by several motorcycle licenses and racing competitions.
- London Buses has a contract with Transport for London to operate route 125.
- Numerous roads have the number 125, which includes the Mexican Federal Highway 125.
- Minnesota’s 125th Street Bridge spans the Mississippi River.
- One of Shakespeare’s 154 sonnets, Sonnet 125, was penned by the English playwright and poet.
Factors of 125 in Pairs
Factor pairs of 125 are any two numbers multiplied together to get the number 125. The following is a list of positive factors pairs:
If 1 x 125 = 125, then the pair factor of 125 is (1, 125). Likewise, let’s examine other pairs:
1 × 125 = 125, (1, 125) is a pair factor of 125.
125 × 1 = 125, (125, 1) is a pair factor of 125.
5 × 25 = 125, (5, 25) is a pair factor of 125.
25 × 5 = 125, (25, 5) is a pair factor of 125.
The positive factor pairs of 125 are these. All you need to do is reverse the signs to determine the negative factor pair. The following is the list of pairings of negative factors:
-1 × -125 = 125, (-1, -125) is a pair factor of 125.
-125 × -1 = 125, (-125, -1) is a pair factor of 125.
-5 × -25 = 125, (-5, -25) is a pair factor of 125.
-25 × -5 = 125, (-25,- 5) is a pair factor of 125.
Factors of 125 Solved Examples
Example 1
Emily is holding a housewarming party. Some of her closest friends and coworkers have been invited. There will be 25 attendees in total.
As it will be their first time visiting her new home. She decided to bake muffins since she wanted to give them all some treats. She has prepared 125 muffins.
How many muffins should she put in each treat box?
Solution
In total, there are 125 muffins, so let’s divide the number of cupcakes by the number of guests.
\[ \frac{125}{25} = 5 \]
This shows that Emily will have to put five muffins in each treat box.
Example 2
During the maths lesson, Helen’s teacher asked a question from her. The task was to identify the factors 100 and 125 had in common. Assist Helen in identifying the common factors between 100 and 125.
Solution
1, 5, 25, and 125 are the four factors of 125.
1, 2, 4, 5, 10, 20, 25, 50, and 100 are the nine factors of 100.
Therefore, 1, 5, and 25 are the factors that both 100 and 125 have in common. So, Helen will tell her teacher that the answer is 1, 5, and 25.
Example 3
Add, Subtract and multiply all the factors by 125.
Solution
The factors of 125 are as follows:
1, 5, 25, and 125
Addition:
1 + 5 + 25 + 125 = 156
Subtraction:
125 – 25 – 5 – 1 = 94
Multiplication:
1 x 5 x 25 x 125 = 15625
All the images/diagrams are made using GeoGebra.
