JUMP TO TOPIC
Factors of 850: Prime Factorization, Methods, and Examples
850 is a composite number that is even. To put it another way, some integers do indeed have products that equal 850. 850 can be divided evenly by a total of 12 integers, indicating that 450 has 12 components.
The pair of two factors that add up to 850 is referred to as the factor pair of 850.
Factors of 850
Here are the factors of number 850.
Factors of 850: 1, 2, 5, 10, 17, 25, 34, 50, 85, 170, 425, and 850
Negative Factors of 850
The negative factors of 850 are similar to its positive aspects, just with a negative sign.
Negative Factors of 850: -1, -2, -5, -10, -17, -25, -34, -50, -85, -170, -425, and -850
Prime Factorization of 850
The prime factorization of 850 is the way of expressing its prime factors in the product form.
Prime Factorization: 2 x 5 x 5 x 17
In this article, we will learn about the factors of 850 and how to find them using various techniques such as upside-down division, prime factorization, and factor tree.
What Are the Factors of 850?
The factors of 850 are 1, 2, 5, 10, 17, 25, 34, 50, 85, 170, 425, and 850. These numbers are the factors as they do not leave any remainder when divided by 850.
The factors of 850 are classified as prime numbers and composite numbers. The prime factors of the number 850 can be determined using the prime factorization technique.
How To Find the Factors of 850?
You can find the factors of 850 by using the rules of divisibility. The divisibility rule states that any number, when divided by any other natural number, is said to be divisible by the number if the quotient is the whole number and the resulting remainder is zero.
To find the factors of 850, create a list containing the numbers that are exactly divisible by 850 with zero remainders. One important thing to note is that 1 and 850 are the 850’s factors as every natural number has 1 and the number itself as its factor.
1 is also called the universal factor of every number. The factors of 850 are determined as follows:
\[\dfrac{850}{1} = 850\]
\[\dfrac{850}{2} = 425\]
\[\dfrac{850}{5} = 170\]
\[\dfrac{850}{10} = 85\]
\[\dfrac{850}{17} = 50\]
\[\dfrac{850}{25} = 34\]
\[\dfrac{850}{34} = 25\]
\[\dfrac{850}{50} = 17\]
\[\dfrac{850}{85} = 10\]
\[\dfrac{850}{170} = 5\]
\[\dfrac{850}{425} = 2\]
\[\dfrac{850}{850} = 1\]
Therefore, 1, 2, 5, 10, 17, 25, 34, 50, 85, 170, 425, and 850 are the factors of 850.
Total Number of Factors of 850
For 850, there are 12 positive factors and 12 negative ones. So in total, there are 24 factors of 850.
To find the total number of factors of the given number, follow the procedure mentioned below:
- Find the factorization/prime factorization of the given number.
- Demonstrate the prime factorization of the number in the form of exponent form.
- Add 1 to each of the exponents of the prime factor.
- Now, multiply the resulting exponents together. This obtained product is equivalent to the total number of factors of the given number.
By following this procedure, the total number of factors of 850 is given as:
Factorization of 850 is 2 x 5 x 5 x 17.
The exponent of 2 is 1, 5 is 2, and 17 is 1.
Adding 1 to each and multiplying them together results in 24.
Therefore, the total number of factors of 850 is 24. 12 are positive, and 12 factors are negative.
Important Notes
Here are some essential points that must be considered while finding the factors of any given number:
- The factor of any given number must be a whole number.
- The factors of the number cannot be in the form of decimals or fractions.
- Factors can be positive as well as negative.
- Negative factors are the additive inverse of the positive factors of a given number.
- The factor of a number cannot be greater than that number.
- Every even number has 2 as its prime factor, the smallest prime factor.
Factors of 850 by Prime Factorization
The number 850 is a composite number. Prime factorization is a valuable technique for finding the number’s prime factors and expressing the number as the product of its prime factors.
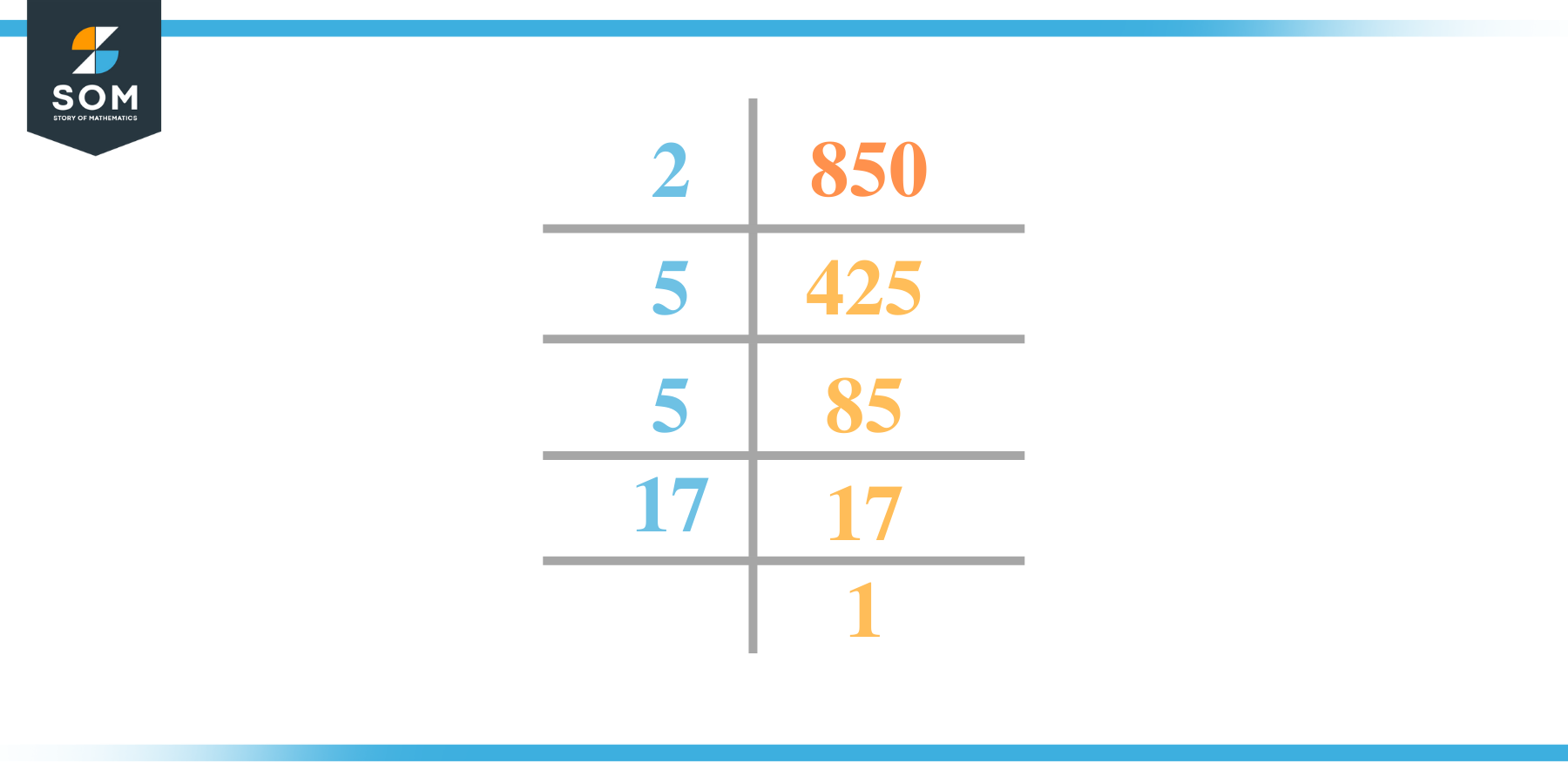
Before finding the factors of 850 using prime factorization, let us find out what prime factors are. Prime factors are the factors of any given number that are only divisible by 1 and themselves.
To start the prime factorization of 850, start dividing by its most minor prime factor. First, determine that the given number is either even or odd. If it is an even number, then 2 will be the smallest prime factor.
Continue splitting the quotient obtained until 1 is received as the quotient. The prime factorization of 850 can be expressed as:
850 = 2 x 5 x 5 x 17
Factors of 850 in Pairs
The factor pairs are the duplet of numbers that, when multiplied together, result in the factorized number. Factor pairs can be more than one depending on the total number of factors given.
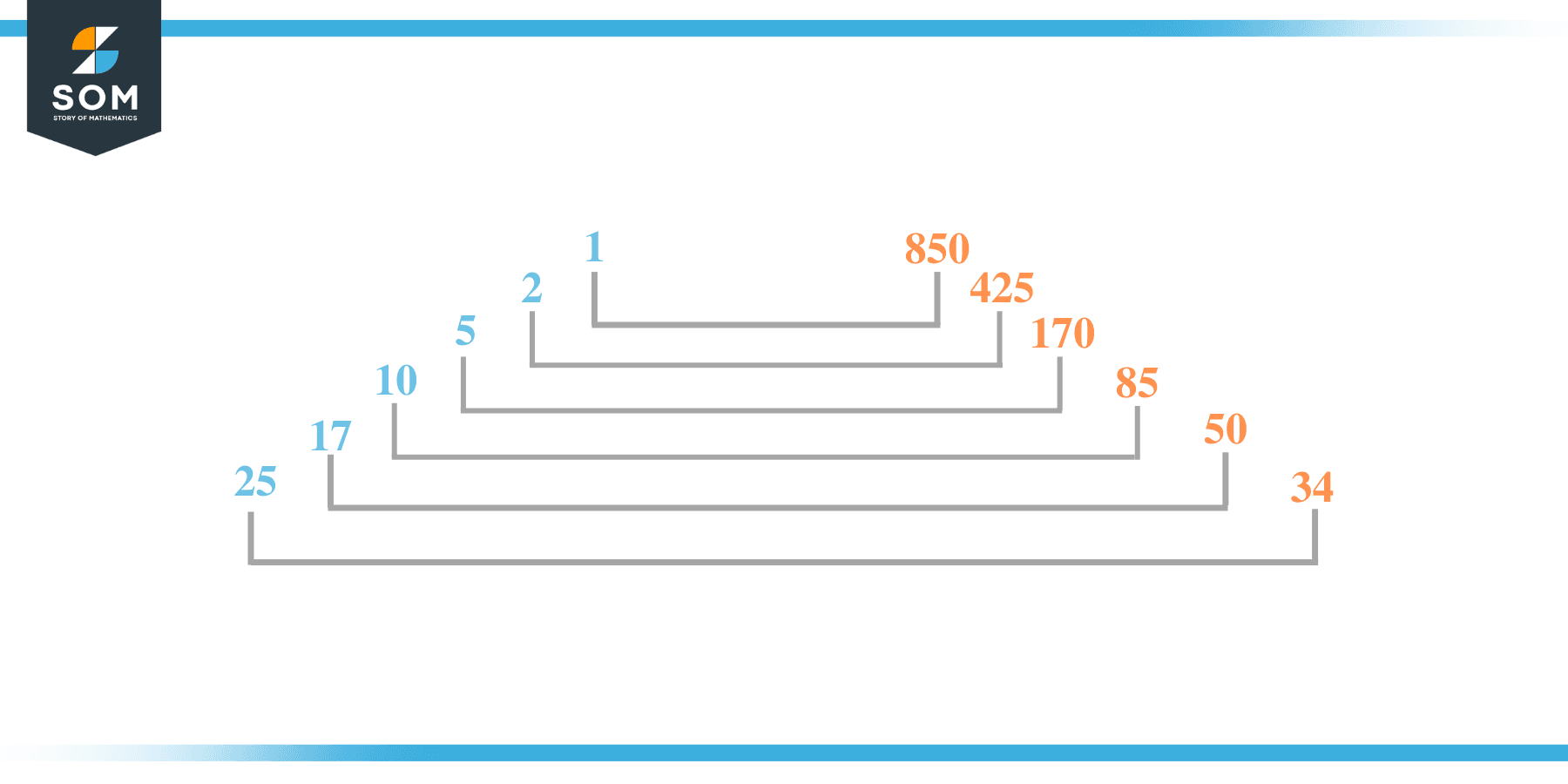
For 850, the factor pairs can be found as:
1 x 850 = 850
2 x 425 = 850
5 x 170 = 850
10 x 85 = 850
17 x 50 = 850
25 x 34 = 850
The possible factor pairs of 850 are given as (1, 850),(2, 425 ),(5, 170),(10, 85),(17, 50),and (25, 34).
All these numbers in pairs, when multiplied, give 850 as the product.
The negative factor pairs of 850 are given as:
-1 x -850 = 850
-2 x -425 = 850
-5 x -170 = 850
-10 x -85 = 850
-17 x -50 = 850
-25 x -34 = 850
It is important to note that in negative factor pairs, the minus sign has been multiplied by the minus sign, due to which the resulting product is the original positive number. Therefore,-1, -2, -5, -10, -17, -25, -34, -50, -85, -170, -425, and -850 are called negative factors of 850.
The list of all the factors of 850, including positive as well as negative numbers, is given below.
Factor list of 850: 1,-1, 2,-2, 5,-5, 10,-10, 17,-17, 25,-25, 34,-34, 50,-50, 85,-85, 170,-170, 425,-425, 850, and -850
Factors of 850 Solved Examples
To better understand the concept of factors, let’s solve some examples.
Example 1
How many factors of850 are there?
Solution
The total number of Factors of 850 is 24.
Factors of 850 are 1, 2, 5, 10, 17, 25, 34, 50, 85, 170, 425, and 850.
Example 2
Find the factors of 850 using prime factorization.
Solution
The prime factorization of 850 is given as:
850 $\div$ 2 = 425
425 $\div$ 5 = 85
85 $\div$ 5 = 17
17 $\div$ 17= 1
So the prime factorization of 850 can be written as:
2 x 5 x 5 x 17 = 850
