What Is 1/46 as a Decimal + Solution With Free Steps
The fraction 1/46 as a decimal is equal to 0.02173913.
A Fraction can be a decimal number both in cases it is an Improper fraction or a Proper fraction. The decimal number consists of two parts the first part is a value that is written before the decimal point and in the second part the value is a fraction of the whole number.
Here, we are more interested in the division types that result in a Decimal value, as this can be expressed as a Fraction. We see fractions as a way of showing two numbers having the operation of Division between them that result in a value that lies between two Integers.
Now, we introduce the method used to solve said fraction to decimal conversion, called Long Division, which we will discuss in detail moving forward. So, let’s go through the Solution of fraction 1/46.
Solution
First, we convert the fraction components, i.e., the numerator and the denominator, and transform them into the division constituents, i.e., the Dividend and the Divisor, respectively.
This can be done as follows:
Dividend = 1
Divisor = 46
We introduce the most important quantity in our division process: the Quotient. The value represents the Solution to our division and can be expressed as having the following relationship with the Division constituents:
Quotient = Dividend $\div$ Divisor = 1 $\div$ 46
This is when we go through the Long Division solution to our problem. The following figure shows the long division:

Figure 1
1/46 Long Division Method
We start solving a problem using the Long Division Method by first taking apart the division’s components and comparing them. As we have 1 and 46, we can see how 1 is Smaller than 46, and to solve this division, we require that 1 be Bigger than 46.
This is done by multiplying the dividend by 10 and checking whether it is bigger than the divisor or not. If so, we calculate the Multiple of the divisor closest to the dividend and subtract it from the Dividend. This produces the Remainder, which we then use as the dividend later.
Now, we begin solving for our dividend 1, which after getting multiplied by 10 twice and adding zero in the Quotient after the decimal point becomes 100.
We take this 100 and divide it by 46; this can be done as follows:
100 $\div$ 46 $\approx$ 2
Where:
46 x 2 = 92
This will lead to the generation of a Remainder equal to 100 – 92 = 8. Now this means we have to repeat the process by Converting the 8 into 80 and solving for that:
80 $\div$ 46 $\approx$ 1
Where:
46 x 1 = 46
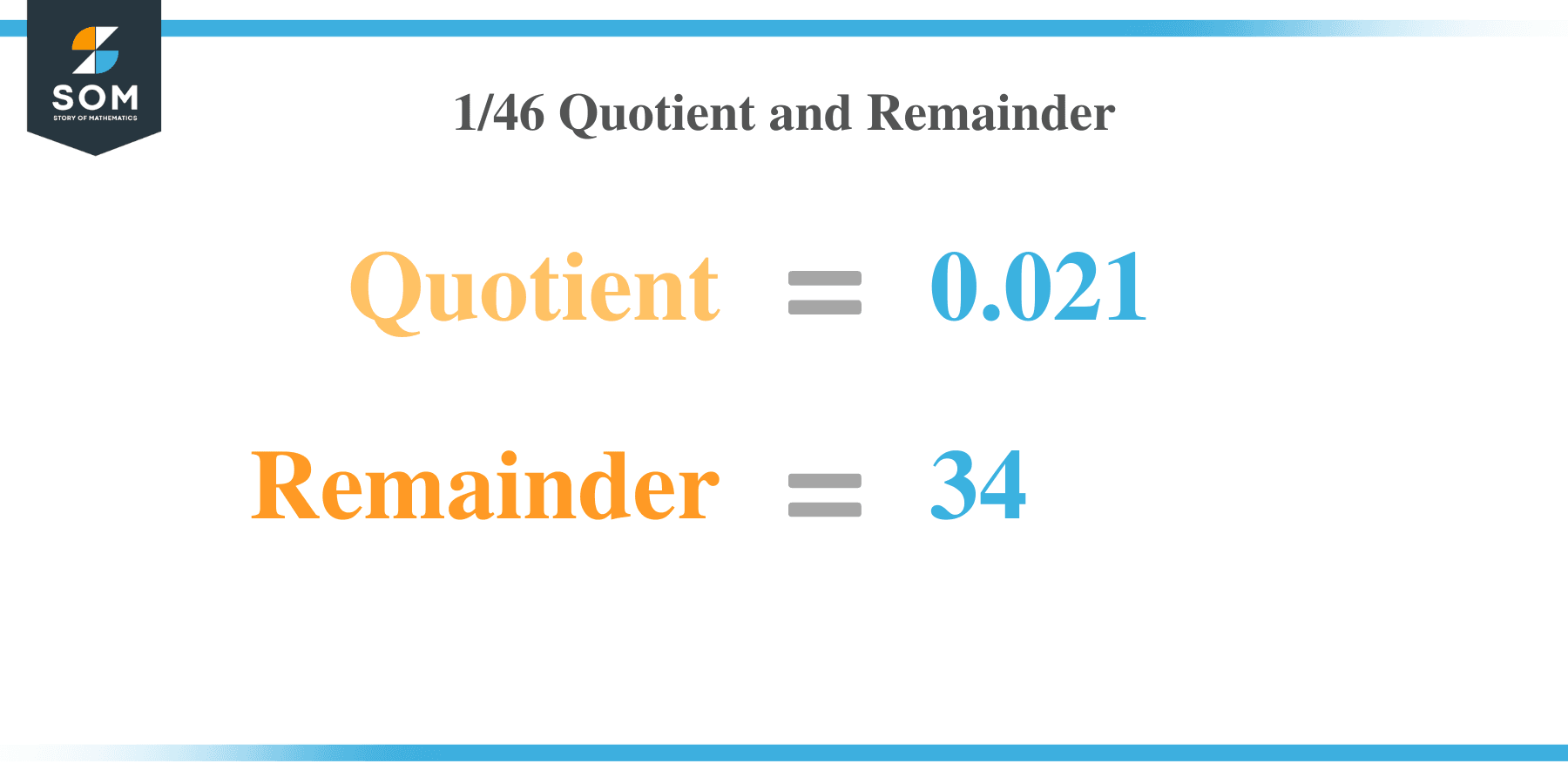
Therefore, Remainder is equal to 80 – 46 = 34. Now we stop solving this problem, we have a Quotient generated after combining the three pieces of it as 0.021=z, with a Remainder equal to 34.
Images/mathematical drawings are created with GeoGebra.
