What Is 3 1/8 as a Decimal + Solution With Free Steps
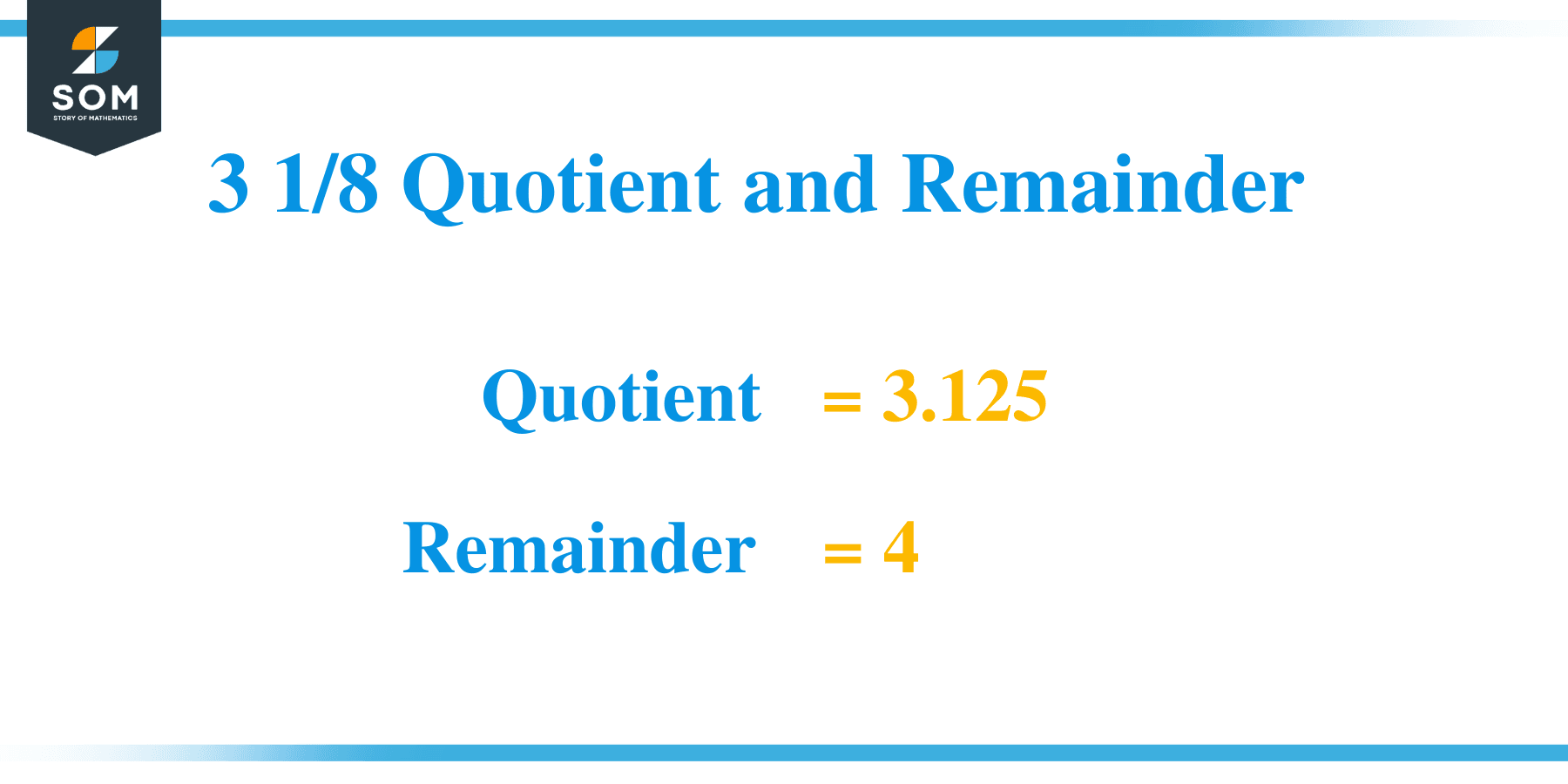
The fraction 3 1/8 as a decimal is equal to 3.125.
Fractions are converted into Decimal values to make them easy to understand. Fractions can be classified into three types: improper fraction, proper fraction, and mixed fraction.
When the fraction has a numerator greater than the denominator, the fraction is known as an Improper fraction. When we have a numerator less than the denominator of the fraction, we call the fraction the Proper fraction. A Mixed fraction has a whole number along with an improper fraction.
To convert fractions into their decimal values, we have to use the mathematical operator called division. The division is one of the toughest mathematical operators among all. We can make this easier by using a method called the Long Division method.
Solution
We need to convert the given mixed fraction into desired p/q form. The p is referred to as the Numerator, while the q in the fraction is known as the Denominator.
To get the numerator from the mixed fraction, we will multiply the denominator by 8 with the whole number of 3 and will add 1 to it while the denominator remains the same. So now we have a fraction of 25/8.
The key concepts used in the long division method are Dividend and Divisor. In the fraction representation of p/q, the p is referred to as the dividend, while the q in the fraction is known as the divisor. Here the dividend and divisor are:
Dividend = 25
Divisor = 8
The solution of the fraction in decimal form is referred to as the Quotient.
Quotient = Dividend $ \div $ Divisor = 25 $ \div $ 8
The long division method for the given fraction is as under: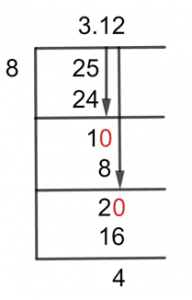
Figure 1
25/8 Long Division Method
The fraction we have is:
25 $ \div $ 8
Here, we can directly divide the two numbers because the dividend is greater than the divisor.
Another key term used in the long division method is “Remainder.” It is the number that remains after the division of numbers that are not completely divisible.
25 $ \div $ 8 $ \approx $ 3
Where:
8 x 3 = 24
For the remainder, we have 25 – 24 = 1. The remainder is less than the divisor, so to proceed further, we need to add zero to the right side of the remainder. For that, we will add a decimal point to the quotient. By doing so, now we have a new remainder of 10.
Now we will divide 10 by the divisor of 8, and we will get:
10 $ \div $ 8 $ \approx $ 1
Where:
8 x 1 = 8
We now have a remainder of 10 – 8 = 2. Again, we will add zero to the right side of the remainder and we will get 20.
20 $ \div $ 8 $ \approx $ 2
Where:
8 x 2 = 16
Finally, we have a resulting Quotient of 3.12 with a Remainder of 4.
Images/mathematical drawings are created with GeoGebra.
