Result|Definition & Meaning

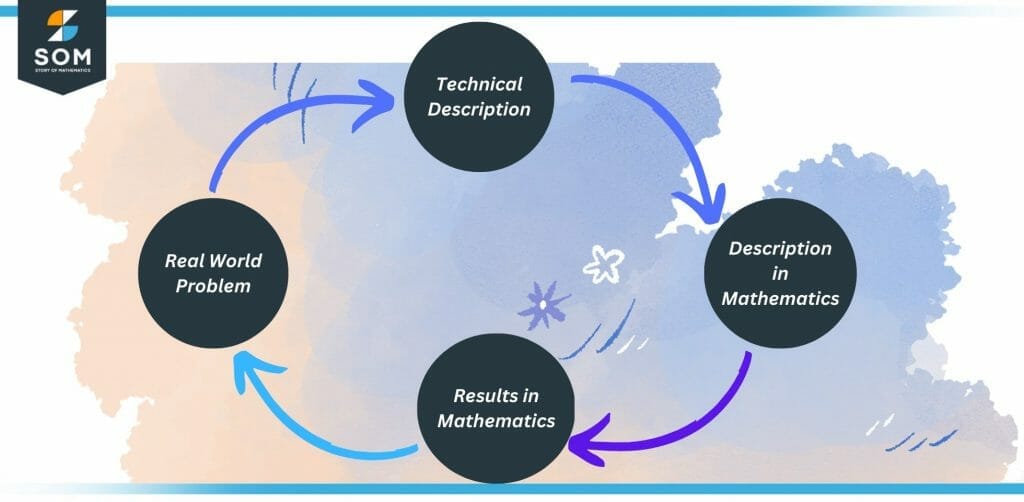
Figure 2 – Cycle of Finding results in math

In order to prove a statement is true, mathematicians use logical reasoning and mathematical techniques to arrive at their conclusions. Building a proof, which is a series of logical steps that show a statement is true, may be a step in this process.

A number of techniques, including proof by contradiction, proof by induction, and proof by construction, can be used to build a proof. Because they lay the groundwork for comprehending and resolving issues in a variety of fields, such as physics, engineering, and computer science, results are an essential component of mathematics.

They are essential in the creation of fresh mathematical theories and concepts.

Types of Results

In mathematics, there are various kinds of outcomes, including:

1. Theorem: A theorem is a proposition that has been shown to be accurate in light of a number of widely acknowledged presumptions or axioms. Because they are thoroughly demonstrated and have numerous applications, theorems are regarded as the most significant form of mathematical result.
2. Lemma: A lemma is a claim that must be established as true in order to support another claim, typically a theorem. Because they serve as a bridge to the primary conclusion, lemmas are frequently utilized to prove theorems.
3. Corollary: A corollary is a conclusion that logically follows from a theorem or lemma that has already been established. Due to their reliance on the veracity of the initial result, corollaries are typically simpler to demonstrate than theorems.
4. Proposition: A proposition is a statement that is put forth for thought but whose truth or falsity has not yet been established. The construction of a proof or the testing of a conjecture frequently begins with a proposition.
5. Conjecture: An assertion that is thought to be true but has not yet been verified is referred to as a conjecture. Observations or patterns found in a particular branch of mathematics are frequently used as the basis for conjectures.
6. Axiom: An axiom is a proposition that is assumed to be true without any supporting evidence. Axioms are taken to be self-evident truths and are used as the foundation for building a proof.

Methods for Obtaining Results

To get outcomes in math, a variety of techniques are employed, such as:

1. Proof by contradiction: In this approach, a claim is first taken as untrue, from which a contradiction is then deduced. This demonstrates that the presumption must be true, proving the validity of the initial claim.
2. Proof by induction: This technique shows that if a claim is true for one base case, it must also be true for the following instance if it is true for the base case. This process is repeated until the assertion is demonstrated to be true in all circumstances.
3. Proof by construction: In this approach, a claim is demonstrated to be true by consciously creating a counterexample or example that shows it to be false.
4. Proof by exhaustion: In this approach, every conceivable scenario is taken into account, and it is demonstrated that the claim is true in each one.
5. Proof by approximation: This technique establishes the truth of a claim by demonstrating that it holds for a series of more precise approximations.

These are only a few of the numerous techniques that are employed in math to arrive at conclusions. The type of problem being tackled, as well as the tools and methods that are at hand, all influence the solution that is chosen.

Figure 3 – Examples of results for operators in math

Application of Results

The applications of mathematical findings are numerous and spread across many industries. Here are a few illustrations:

1. Physics: Mathematical results are utilized to comprehend and describe physical events, such as particle behavior, celestial body motion, and material qualities. For instance, mathematical findings serve as the foundation for the laws of thermodynamics, which describe the connection between heat, work, and energy.
2. Engineering: In engineering, structures, machines, and systems are designed and analyzed using math results. For instance, the design and analysis of control systems use the results from calculus and differential equations. such as those found in autos and aircraft.
3. Computer Science: Algorithms and data structures in computer science are designed and analyzed using math results. For instance, results from combinatorics and graph theory are used to create effective algorithms for resolving issues in computer science.
4. Economics: Mathematical results are used to model and analyze economic systems and processes, such as market behavior and resource distribution. For instance, results from probability and game theory are used to comprehend and forecast the actions of economic actors.
5. Medicine: In medicine, mathematical models and analyses are used to simulate and examine biological systems and processes.

Results from statistics and probability, for instance, are used to plan and evaluate clinical studies and comprehend the spread of diseases. These are just a few instances of the numerous applications of math in a variety of professions.

Challenges and Limitations of Results

The process of getting results in math can be difficult and time-consuming, and there are a number of difficulties and restrictions that might occur:

1. Complexity: A lot of math problems are extremely complicated and challenging to solve. This can make it difficult to put together a proof or come up with a fix.
2. Limited resources: Tools for solving math issues, including as theorems, lemmas, and procedures, are sometimes in short supply.Finding the ideal strategy for resolving a problem can become challenging as a result.
3. Intuition and creativity: Getting outcomes in arithmetic frequently calls for a combination of intuition and imagination to generate fresh concepts and methods. This can be challenging because it calls for both a thorough comprehension of the material and the capacity for abstract and original thought.
4. Current state knowledge limitation: There are still a lot of unsolved math issues, and because they typically include concepts and ideas that are still being better understood, it can be challenging to make progress on them.
5. Current methods have limitations: It’s common for math problem-solving strategies and methods to have restrictions. For instance, certain problems might be too difficult to be solved by current techniques, or those techniques might be ineffective or unworkable.

Mathematicians continue to make tremendous progress in comprehending and resolving mathematical issues, and new findings are constantly being made in spite of these difficulties and restrictions.

Examples of Problems and Their Results

Example 1

Find the result of Adding 45 and 26, then take 10 out of the total.

Solution

The result of adding 45 and 26 is:

Sum = 45 + 26 = 71

10 is now subtracted from the total, giving us:

71 – 10  = 61

Example 2

Find the result of  35 + 35 + 35 +35 + 35.

Solution

Provided, 35 + 35 + 35 +35 +35

It is obvious that 35 is increased by five. Thus, we can write 5 times 35 equals 5 x 35 and 175.

The solution is the same if we simply add them.

Example 3

Find the Result for the equation (5 x 7) ÷ 5 + 80 ÷ 10 – 4.

Solution

Provided:

(5 x 7) ÷ 5 + 80 ÷ 10 – 4

⇒ (35 ÷ 5) + (80 ÷ 10) – 9

Applying the BODMAS rule:

⇒ 7 + 8  – 4

⇒ 15 – 4

⇒ 11

All images/mathematical drawings were created with GeoGebra.