JUMP TO TOPIC
Factors of 23: Prime Factorization, Methods, Tree, and Examples
Factors of 23 refer to the numbers that give off no reminder; the answer is in whole numbers when the numbers are divided by 23. The answer should never be in decimals, fractions, or any other form if it is a numerical factor.
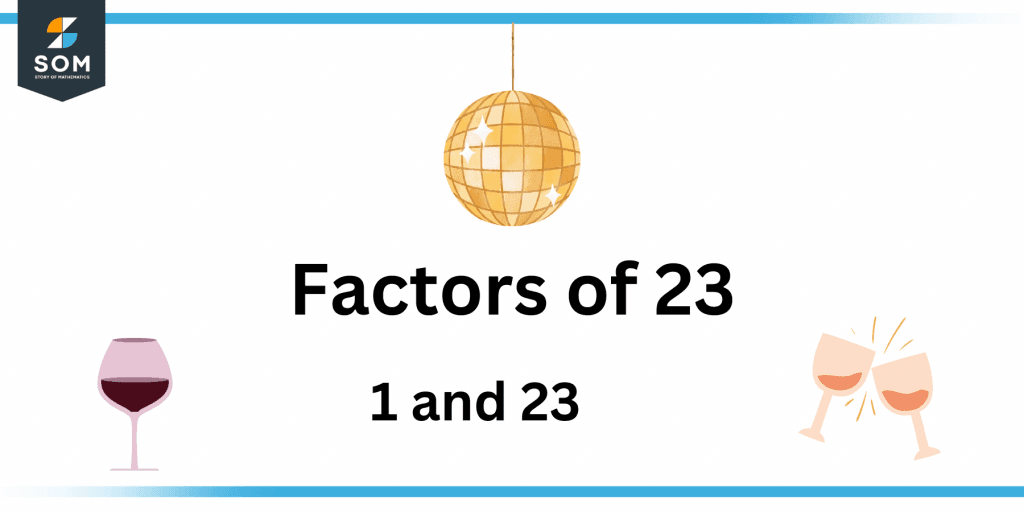
Figure 1 – All possible Factors of 23
Finding the factors of a specific number is not a complicated process as you can find them by either simple multiplication or basic division. To check factors of a number, list down the half and the number more than the half of 23. Each of them has to be divided by the number given, i.e., 23.
The number will either be a composite or a prime number. A composite number will have more than two factors, whereas a prime number always has 2 factors only. The factors of a prime number are always either 1 or the number itself, and 23 is a prime number.
Thus, in such a case, no division of numbers will give us 0 remainder or the answer in a whole number other than the division of 23 or multiplication of 1 with the number 23. If you go for the division method when finding factors of a number, the quotient and divisor are both considered factors of a number.
In the following article, you’ll get a detailed account of the factors of the number 23, how you can calculate the numbers and the easiest ways to represent the factors of a number. Let’s wait no more and get started!
What Are the Factors of 23?
Factors of 23 are 1 and 23. The following number has two factors only as it is a prime number which is why the factors are 1 and the number.
When put into factor pairs, 23 only has 1 positive and one negative factor pair, i.e., when 1 is multiplied by 23, the product, i.e., the answer, is 23.
Similarly, in the case of division, the only division will be when 23 is divided by 23; the answer is 1. It is considered a factor as the answer is in whole numbers, and no remainder is left.
How To Calculate the Factors of 23?
You can calculate the factors of a specific number by using two methods: division and multiplication. Though, it is not at all a difficult thing to do. Let’s quickly review how you can find the factors of 23 using both these methods.
A pro tip is first to know if the number is either a prime number or a composite number. Suppose it’s a composite number; you need to divide the given number by two as divisibility can be checked by numbers half or more than half of the specific number.
However, if the number given to you is a prime number, the procedure is even simpler as prime numbers always have only two factors- 1 and the number itself. To check if the number is a prime number, start by dividing it by a random number. Let’s take a look at a few examples:
\[ \frac{23}{3} = 7.6667 \]
\[ \frac{23}{5} = 4.6 \]
As it can be seen from the divisions done above that neither is the answer in whole numbers nor does it doesn’t have a remainder, they can not be considered as factors of 23. To be considered a factor of the number, the divisor is supposed to give the quotient in whole numbers (not in fractions or decimal form).
In addition, no remainder should be left once the division is done. Now let’s look at how we get the only two factors the number 23 has as it is a prime number.
The answer in the whole number is only gotten when we divide 23 by 23, the answer is 1, and no remainder is left. Thus, this makes the number 1 and 23 the factors of 23. The only positive factor pair of 23 is also (1, 23), and the negative factor pair is (-1,-23).
There is only one possible division of the number 23, which is mentioned below:
\[ \frac{23}{23} = 1 \]
Thus, factors 23 are 1 and 23.
Factors of 23 by Prime Factorization
Among various ways of figuring out how we can represent factors of a specific number, Prime factorization is one of the most used ways. Through Prime Factorization, you can quickly determine the number that multiplies together to give 23 as the answer.
A prime number is a number with only two factors- 1 and the number itself, and the number 23 is a prime number. Thus, to get the prime factors of 23, you don’t need to put in much effort as you can’t break down the quotient by dividing numbers in this case.
For surety, you can check if the number can further be divided by a division of 23 with random numbers e.g.
\[ \frac{23}{2} = 11.5 \]
\[ \frac{23}{3} = 7.6667 \]
\[ \frac{23}{5} = 4.6 \]
Thus, as the answers to these divisions are not in whole numbers thus, they can’t be considered a factor of 23. The only way 23 can be prime factorized is:
\[ \frac{23}{23} = 1 \]
Therefore, the prime factors of 23 are 1 and 23.
The prime factorization of 23 can also be seen in the diagram attached below:
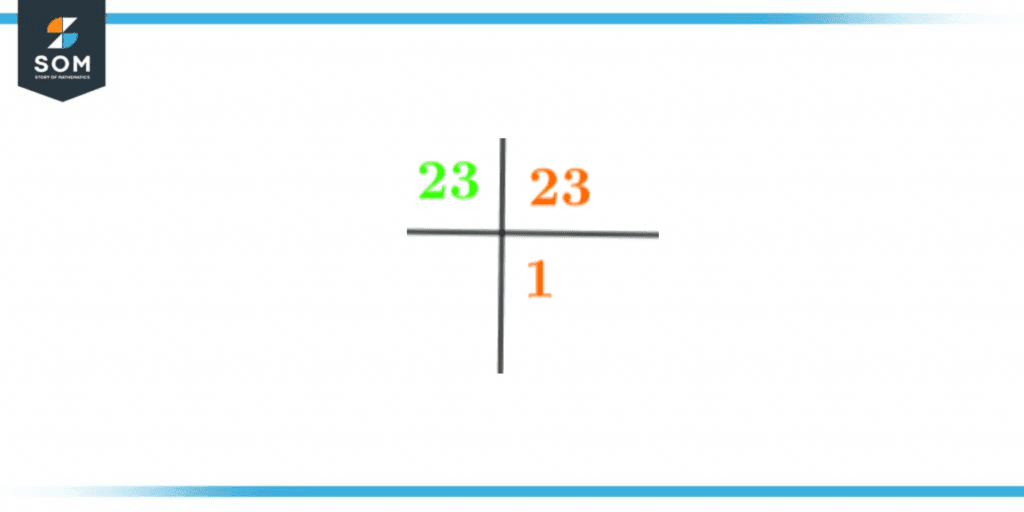
Figure 2 – Prime Factorization of 23
Factor Tree of 23
Factors of a number can be represented in various ways, and expressing the factors of a specific number through a Factor Tree is one very commonly used way. Saying factors on a factor tree is fundamental and makes the concept easy to understand.
The Factor Tree begins with the number itself on the top and through branches; the tree keeps extending till we get the prime number at the end of the tree. As per Prime Factorization, we know that only 1 and 23 are the factors of 23; thus, the last numbers on the factor tree of 23 should be both of these factors.
For a more precise idea, you can take a look at the Factor Tree of the following number below:
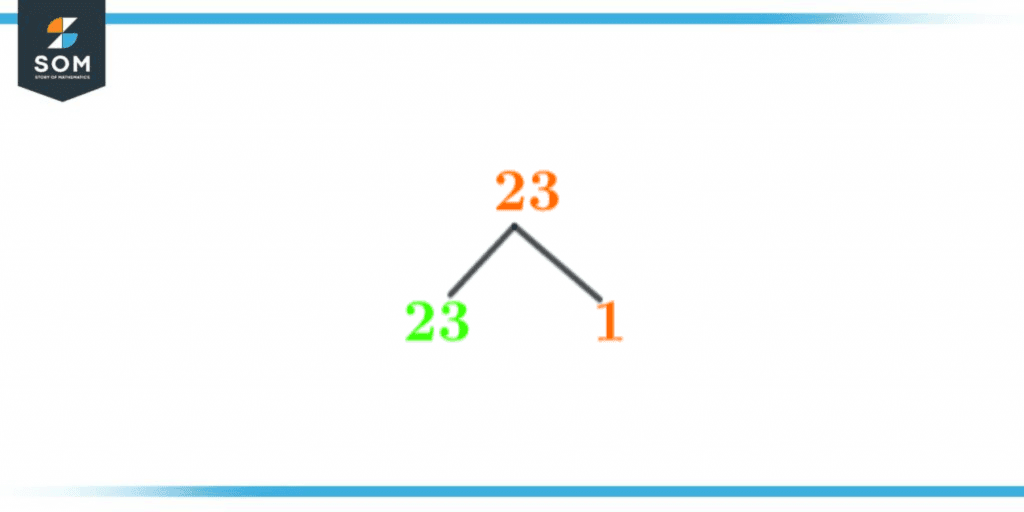
Figure 3 – Factor Tree of 23
Factors of 23 in Pairs
The Factor pair refers to when divided by a specific number, the remainder is always zero, and the answer should never be in decimals or fractions. When such division happens, a factor pair is made by considering the quotient and divisor as factors of the specific number.
As the number 23 is a prime number, it only has two factors; thus, just a one-factor pair. In the Factor pairs, the number, i.e., 23, in this case, is called the product, and to find a factor pair, there are just a few easy steps.
The very first step is to check if the number is a composite or prime number, as it makes the next step easy. You now need to find all the factors of the number. If it’s a prime number, there will be two factors only- one and the number itself.
Once you’re done figuring out the factors, start multiplying them with each other, and the ones that give the specific number as the answer will be considered as a factor pair of the number 23. There is only one-factor pair for the number 23, which is mentioned below:
1 x 23 = 23
Factors of 23 Solved Examples
Now that we know how to calculate and represent the Factors of this number. Let’s look at a few examples of how to solve queries related to the following number, 23:
Example 1
How many even numbers are there in the factors of the number 23?
Solution
The first step is to note down all the factors of 23 that are:
Factors of 23:
1 and 23
As the number has only two factors, 1 and the number itself. It means that 23 is a prime number that is only divisible by one and the number itself.
Thus the answer is none, as both the number factors are odd numbers.
Example 2
What are the common factors of 23 and 27?
Solution
Finding common factors is very basic. To find the common elements of two numbers, you first have to find the factors through either multiplication or division.
Then note down all the factors and circle out the common ones. For this question, the steps will be as follow:
The factors of number 23 are:
1 and 23
Whereas the factors of 27 are:
1, 3, 7 and 27
Thus, the common factor of the numbers 23 and 27 is just 1, as 23 is a prime number with only two factors.
All images/mathematical drawings are created using GeoGebra.
