JUMP TO TOPIC
Greater Than|Definition & Meaning
Definition
To illustrate the inequality between two values, amounts, or numbers, mathematicians make use of the “greater than” and “less than” symbols.
They demonstrate which one is greater or smaller than the other, and are utilized greatly in various fields of mathematics,
especially for modeling constraints on equations.
The Symbol of “Greater Than”
The sign of greater than is “>” and it is utilized wherever there is a need to convey the notion of inequality between two variables. This mathematical symbol is made up of two slashes of equal length, and they are connected at the acute angle on the right side.
When comparing two different numbers, we typically utilize the “greater than” sign. In this instance, the first value is higher than the second value that was provided. As a result, you can observe that the sign for “greater than” is an imitation of the angle bracket that closes.
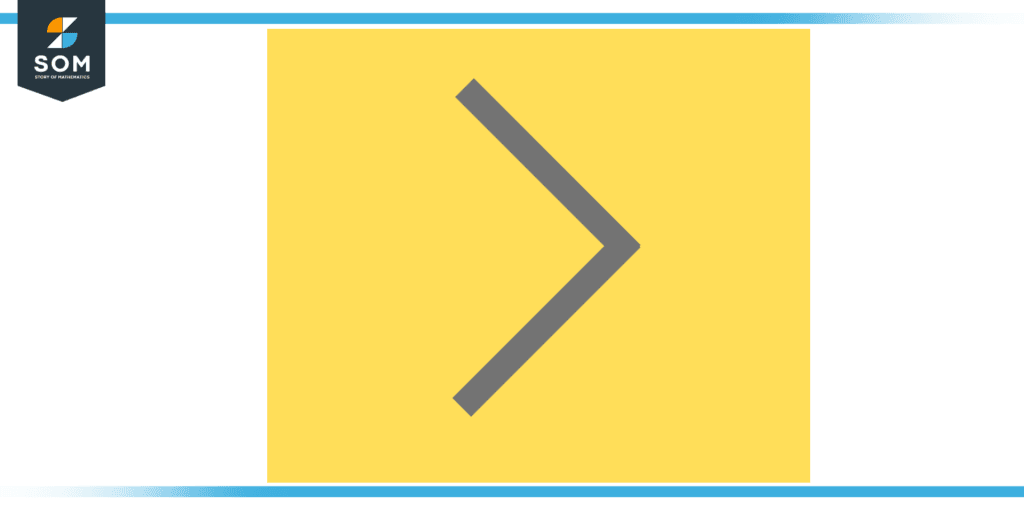
Figure 2: Symbol of Greater Than
What Does It Mean for Something To Be Greater Than the Other?
When you are doing mathematical comparisons of different sets of data, you will find out that not all are equivalent. It is possible that you will come across or be searching for a set of values that satisfies a particular criterion. This variety of possible outcomes can manifest in the following three primary ways:
- The values might be higher than a particular number.
- They can be lower than a predetermined threshold number.
- Neither greater nor lower, rather Between the two numbers.
When something like this occurs, we utilize symbols to describe the way we are attempting to compare the values we are operating with, whether they are specific numbers or variables.
The larger than sign is represented by the symbol “>.” The “greater than” sign indicates that one or maybe more numbers are higher than the value that has been specified.
When comparing two variables, the phrase “greater than” is employed to indicate that one of the quantities is larger than the other.
However, it is also possible to convey that two variables are equivalent by using the phrase “is equal to.” When these two terms are joined with each other, a new phrase “Greater Than or Equal To” is generated. This term is used to demonstrate that the limit on the quantity or amount value could be equal to or greater than the limit that has been specified.
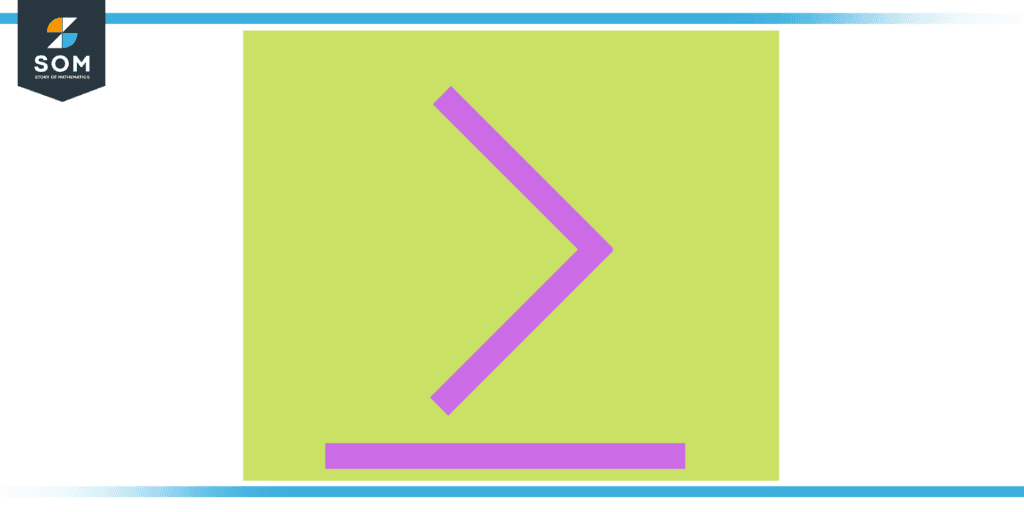
Figure 1: Symbol of Greater Than or Equal To
To give just one illustration, let us say the minimum age requirement for a candidate to become the head of the department in a university is 45 years old. This indicates that the age of the candidate must be “greater than or equal to” 45 years.
Where Did the Symbols for “More Than” and “Less Than” Come From?
During the year 1631, renowned British scientist Thomas Harriot published his work “Artis Analyticae Praxis ad Aequationes Algebraicas Resolvendas,” in which he debuted these symbols for the very first time. These symbols were devised to emphasise ‘inequalities‘ among mathematical values and were utilised in the sophisticated algebraic calculations that Harriot employed in the book. Harriot’s book was based on the work of John H. Harriot.
Methods To Remember Greater Than Symbols
The Alligator’s Mouth Method
An interesting way to understand the concept of inequalities or the signs of greater than and smaller than is through the Alligator mouth method. Consider the signs of “greater than” and “less than” to be the mouth of an alligator, and the values to the left and right of it to be small fish.
The alligator’s primary objective will always be to consume a greater quantity of fish. Thus, the alligator’s jaws will be opened towards the greater of the two numbers because that’s how it works.
In case you were provided with the numbers 6 and 9 and were told to demonstrate whether the number is larger or whether the number is smaller than the other, the alligator’s mouth would be turned towards the number 9. This demonstrates that 6 is a lower number than 9.
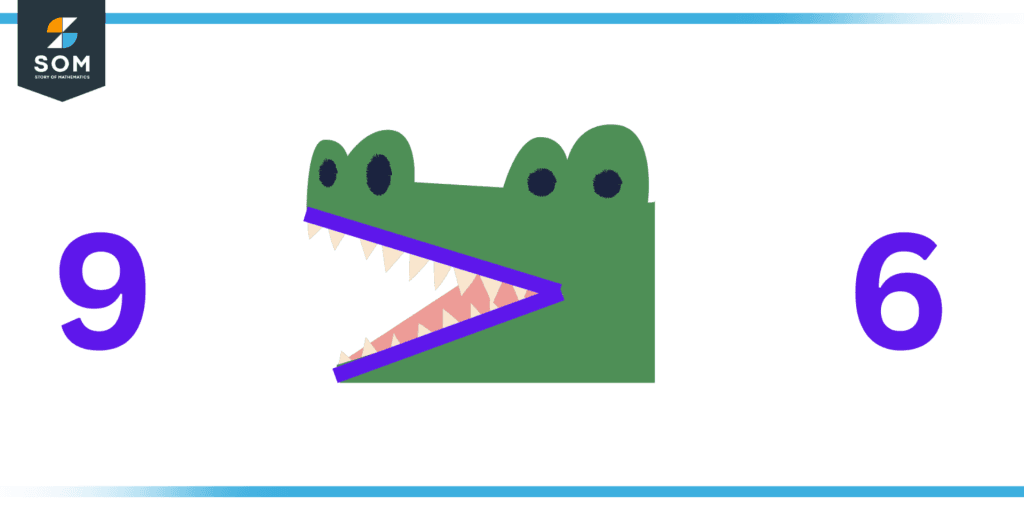
Figure 3: Alligator Mouth Method
The Rule of the “L” Method
Using the ‘L Rule’ is yet another simple way that may be used to easily recall the symbols of greater than and less than. Following is the one thing you need to keep in mind above all else if you want to be able to differentiate between the larger than symbol and a “less than” symbol: The phrase “less than” begins with an “L,” and the sign for “less than” is represented by the “<“.
If you observe the sign a bit keenly, you will notice that it resembles an ‘L‘ that is skewed. Therefore, a symbol for less than is any sign that resembles the letter ‘L’ in any way.
The Rule of 7 Method
Applying the “rule of 7” is yet another useful strategy that can be utilised in order to better retain the “greater than” and “less than” symbols in our memory. In the same way as using the rule of L, Utilizing the rule of 7 will allow you to distinguish between both of the symbols.
To be able to tell the difference between the “greater than” symbol and the less than symbol you need to keep in mind that the “greater than” symbol is the one with a side that is shaped like a 7. In this particular instance, the symbol for more than looks like an inverted number seven, and as a result, it is the symbol for greater than.
Examples of “Greater Than” Inequalities
Example 1
John is a student who has gathered 600 dollars in his savings account by the start of summer vacation. He has planned some fun activities to spend his money on, but at the same time, he wants to have more than 300 dollars remaining in his savings account for the period after the summer vacation. He plans to draw out 25 dollars from his savings account weekly for 10 weeks.
Express the scenario in a mathematical form using an inequality equation. Also, find out at the rate of withdrawal mentioned above, will john have more than 200 dollars remaining at the end of vacation?
Solution
To develop a general equation for the given scenario, let us say john wants to spend 25 dollars for ‘y’ number of weeks. According to the given data john should have more than 300 dollars out of 600 dollars at the end of the vacation. Now we can express the situation in the following manner.
600 – 25 (Y) > 300
Now let us check whether John will have more than 300 dollars at the end of 10 week long summer vacation after withdrawing 30 dollars every week.
600 – 25 (10) > 300
600 – 250 > 300
350 > 300
Since the above equation is true. Therefore, John will have more than 300 dollars at the end of 10 week long summer vacation.
Example 2
Robert got 40 marks on the science exam whereas Adam got 55 marks. Using the inequality symbols, show who scored better than the other.
Solution
Robert’s score on the science exam = 40
Adam’s score on the science exam = 55
Since 55 is greater than 40, therefore, we can express the given situation using inequality symbols as follows.
55 > 40
Or in other words,
Adam’s score in the science exam > Robert’s score in the science exam
All images/mathematical drawings were created with GeoGebra.
