JUMP TO TOPIC
Pi|Definition & Meaning
Definition
The circumference-to-diameter ratio of any circle is known as pi. It is an irrational number, and like most mathematical constants, it is denoted by a Greek letterπ.
Its value in decimal form is roughly 3.14159.
No matter how big the circle is, this ratio will always be equal to pi.
The ratio of a circle’s circumference to its diameter is known as pi. Pi is a real number that cannot be represented by a straightforward fraction since it is an irrational number. Pi is what mathematicians refer to as an “infinite decimal” since the digits continue indefinitely after the decimal point.
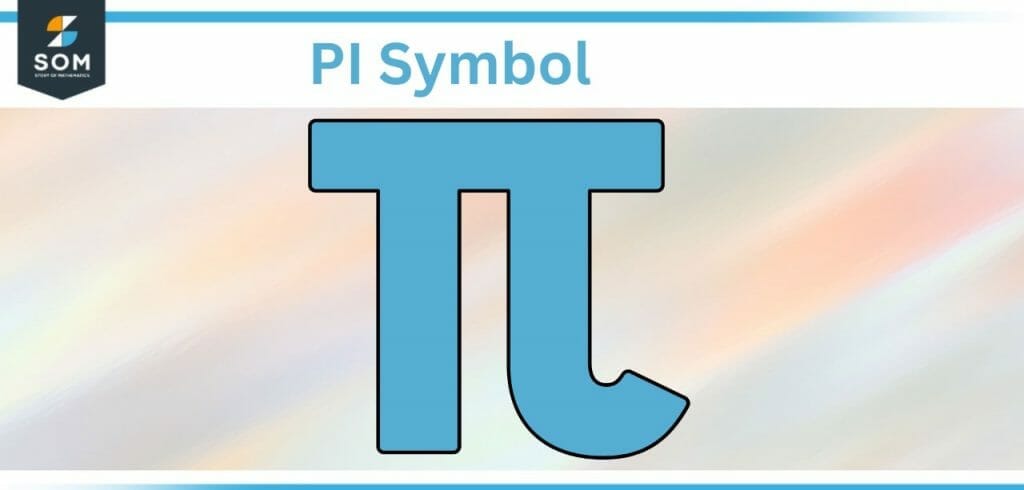
Figure 1 – Symbol of Pi
Pi is typically introduced to students as having a rough value of 3.14 or 3.14159. Although pi is an irrational number, some individuals estimate it using rational formulae like 22/7 or 333/106. (These rational expressions only have a few decimal places of accuracy.)
Calculating pi to as many digits as feasible is of interest to mathematicians and math aficionados. According to the Pi World Ranking List, Suresh Kumar Sharma of India holds the record for reciting pi to the most decimal places, reaching 70,030 in 2015.
The value of pi has been estimated by certain computer algorithms to an amazing 62.8 trillion digits. Pi Day, a fictitious festival celebrated annually on March 14 (3/14), is a day when calculations like this are frequently revealed.
What Is the Pi Value?
Since “pi” has a constant value, it cannot be altered. It is an irrational number that is typically rounded to 3.14. It is incorporated into numerous formulas to evaluate the surface area and volume of several solid shapes.
The ratio of a circle’s circumference to its diameter is known mathematically as “Pi.” The longest line segment that traverses the center of a circle is understood to be the diameter of the circle.
Imagine that a portion of the circle’s circumference is covered by the diameter line after it has been bent. Now, pi is defined as the total number of times the circle’s circumference is wrapped around its diameter or roughly 3.14 times.
The answer is roughly 3.14 when we divide the diameter by the circumference. Remember that the ratio of a circle’s circumference to its diameter will always be the same, regardless of the size of the circle.
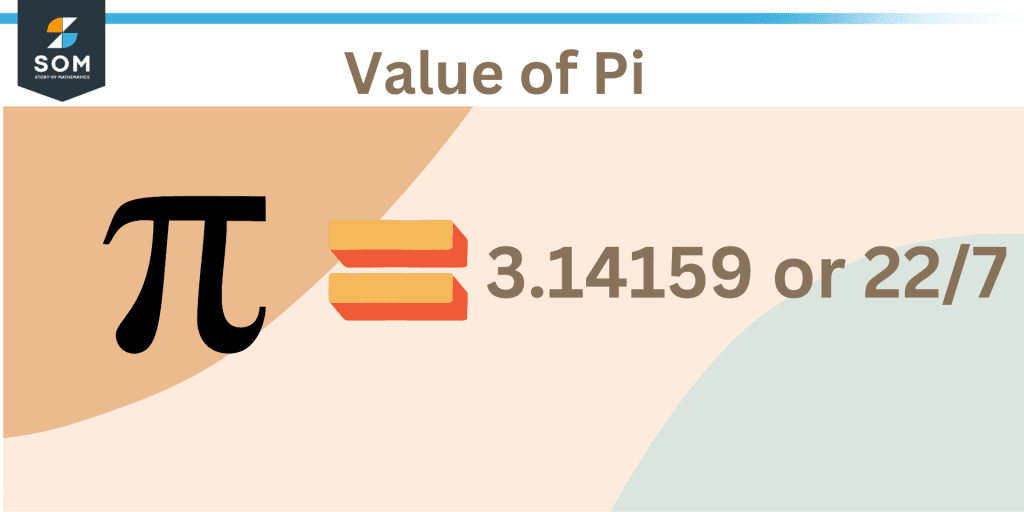
Figure 2 – Value of irrational number pi
Pi’s Symbol
In mathematics, the symbol for pi is represented by the Greek letter. Since its value is constant, it is referred to as a mathematical constant. Pi is a mathematical constant that can be written as 3.14 in decimal form or 22/7 in fractions.
Pi (π) in Decimal Values
Up to the first 50 decimal places, pi is equal to: 3.14159 26535 89793 23846 26433 83279 50288 41971 69399 37510
Pi’s Value Expressed in Fractions
22/7 is the pi fractional value. It is well known that pi is an irrational number, meaning that the decimal place is followed by an infinite amount of digits, making pi a non-terminating value. As a result, 22/7 is used in common calculations. i is an irrational number since it cannot be expressed as the ratio of any two numbers.
Origin of Pi
The ratio of a circle’s circumference to its diameter is known as pi. Pi is defined as the circumference divided by the diameter (c/d), in other words. On the other hand, a circle’s circumference is equal to pi times its diameter (c = d). Pi always equals the same number, regardless of how big or tiny a circle is.
Pi (π) is the name of the well-known mathematical constant and is represented by the 16th letter of the Greek alphabet, which is.
Pi Invention
Pi was discovered by the ancient Babylonians and has been around for around 4,000 years. According to the Exploratorium in San Francisco, pi was discovered to be 3.125 on a tablet that was created between 1900 and 1680 B.C. The Rhind papyrus from 1650 B.C. shows that similar discoveries were being made in ancient Egypt.
In this writing, the Egyptians used a method that gave pi a rough value of 3.1605 to calculate the area of a circle. According to a communication that appeared in the magazine Nature in 1999, there is also a biblical scripture where it seems pi was approximately calculated:
He then created a molten sea that measured ten cubits from brim to brim, was five cubits tall, and had a line of thirty cubits around it. — I Kings 7:23 (King James Version)
According to the Exploratorium, Greek mathematician Archimedes of Syracuse (287 B.C. to 212 B.C.) performed one of the earliest calculations of pi. The Pythagorean theorem was utilized by Archimedes to calculate the areas of two polygons.
Based on the areas of a regular polygon that was encircling the circle and a regular polygon that was inside of it, Archimedes calculated the approximate area of a circle. The upper and lower bounds for the size of a circle were determined by the polygons as Archimedes mapped them, and he estimated pi to be between 3 1/7 and 3 10/71.
Earlier, Chinese mathematician and astronomer Zu Chongzhi (429–501 B.C.) used a similar technique to compute pi and discovered its value was 355/113. Zu’s writings have sadly been lost. Therefore, little is known about his methodology or accomplishments.
In 1706, William Jones, a British mathematician, was the first to use the sign to denote pi.
Pi Formula
By dividing a circle’s circumference by its diameter, one may determine pi. The formula for determining pi is: pi = Circumference/Diameter because pi is the ratio of a circle’s circumference to its diameter.
“How I wish I could calculate pi” is a clever way to help us remember the value of pi. We can quickly write the value of pi by calculating how many letters are contained in each word. Since “How” has three letters, “I” has one, “want” has four, “I” has one, “could” has five, “calculate” has nine, and “pi” has two. Consequently, 3.141592 is the estimated value of pi to six decimal digits.
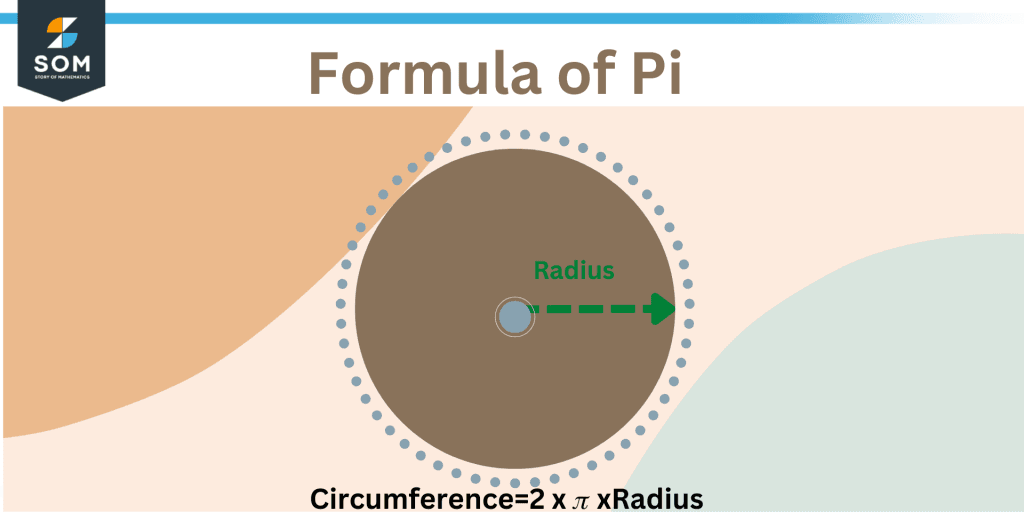
Figure 3 – Relation of pi with a circle’s circumference and radius
How Do You Find Pi’s Value?
With the use of a straightforward activity, the value of pi may be computed. To find out why pi is the ratio of a circle’s circumference to its diameter, follow the instructions below:
- Draw a circle with a diameter of 1 unit.
- Following this, grab a thread and stitch it to the circle’s edge (the circumference).
- Lay the thread on the ruler and record the length. Repeat the procedure with diameters of 2, 3, 4, and 5, then note your observations.
- We can see that the circumference to diameter ratio, which is always 3.1, is constant.
Key Information
- The following is some important information regarding the pi symbol and value:
- The reciprocal of the ratio of a circle’s circumference to its diameter is known as “Pi,” a mathematical constant.
- It is an irrational number that is frequently calculated as 3.14159.
- Based on the issue, we utilise pi as 22/7, or 3.14, to simplify calculations.
Example of Pi’s Usage in Math
A circle has a circumference of 66 units. If the value of pi is assumed to be 22/7, what is the diameter of the circle?
Solution
The circle’s circumference is 60 units, and pi is equal to 22/7.
We shall therefore change the values in the formula for a circle’s Diameter,
The formula is pi = Circumference/Diameter.
The formula for diameter will become Diameter = Circumference/Pi
Diameter = $\frac{60}{\frac{22}{7}} = \frac{{60}\times {7}} {22} $ = 21 units
The circle’s diameter is, therefore, 21 units.
All images/mathematical drawings were created with GeoGebra.
