JUMP TO TOPIC
Precision|Definition & Meaning
Definition
Precision measures the closeness of a group of values (usually measured as part of an experiment) but ignores the truthfulness of the values in the process (called accuracy). Measuring precision helps us identify the consistency of an experiment’s results. Alongside accuracy, the two quantify the overall quality of the experiment’s results.
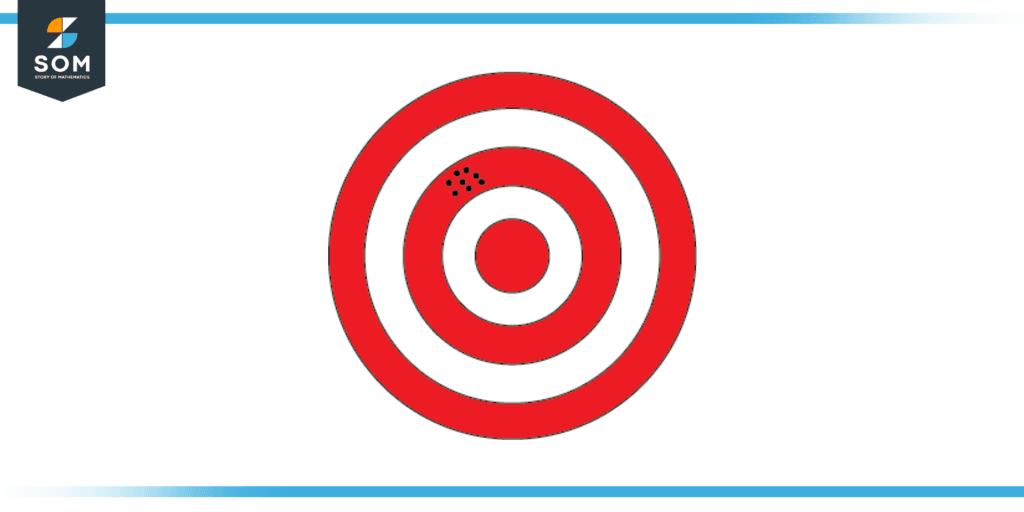
Figure 1: The darts are precisely targeted as they are close to each other.
Precision measures the percentage of instances or samples that are rightly classified among those that are categorized as positives. The formula for precision is defined as true positives divided by false positives, or:
Precision = TP / (TP + FP)
Difference Between Precision and Accuracy
We frequently use phrases like “spot on” and bullseye. These sentences typically appear when one deduces the correct answer to a query. How closely a measurement resembles the “actual” value is indicated by the data’s accuracy
For instance, the time period of a simple pendulum is 0.8 seconds. When student A calculates it using the apparatus in the laboratory, it comes out to be 0.74 seconds, while student B calculates it to be 0.78 seconds. We can say the latter has a more accurate reading.
Now, let us consider the bullseye as an illustration. The very center of the target, which is the “actual” value, must be struck in order for one to be judged to have thrown a dart with great accuracy.
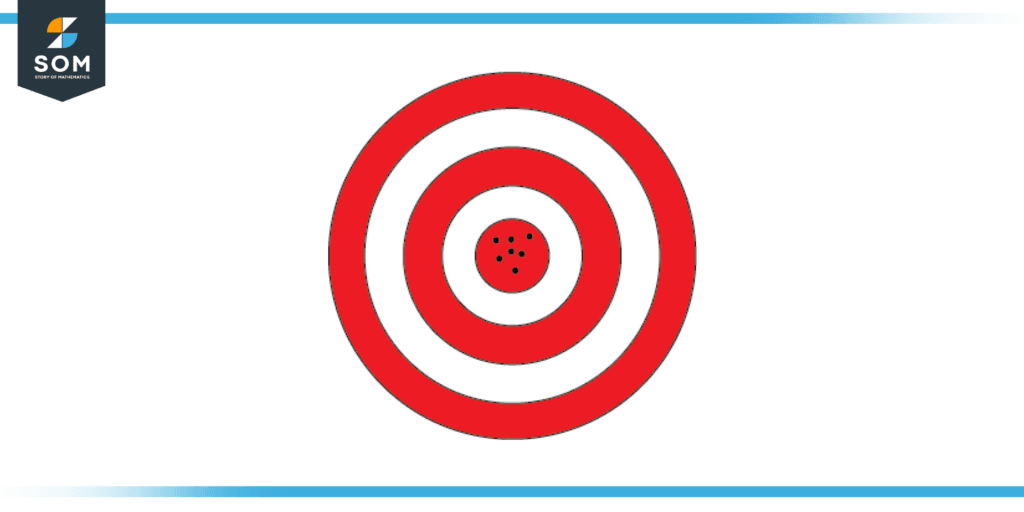
Figure 2: The darts are accuately targeted as they are hitting the center of the target.
However, if a shot repeatedly misses the center of the target, it would be seen as inaccurate because it is far from the “actual” value. Therefore, accuracy will decrease the further one goes from the center.
The hit is far from the center when the darts are thrown off. But one always strikes the same place. In this instance, the person was precise rather than accurate. The agreement of multiple testing is what is meant by precision.
If the darts neither hit the bullseye nor fall close to each other, then there is no precision as well as accuracy.
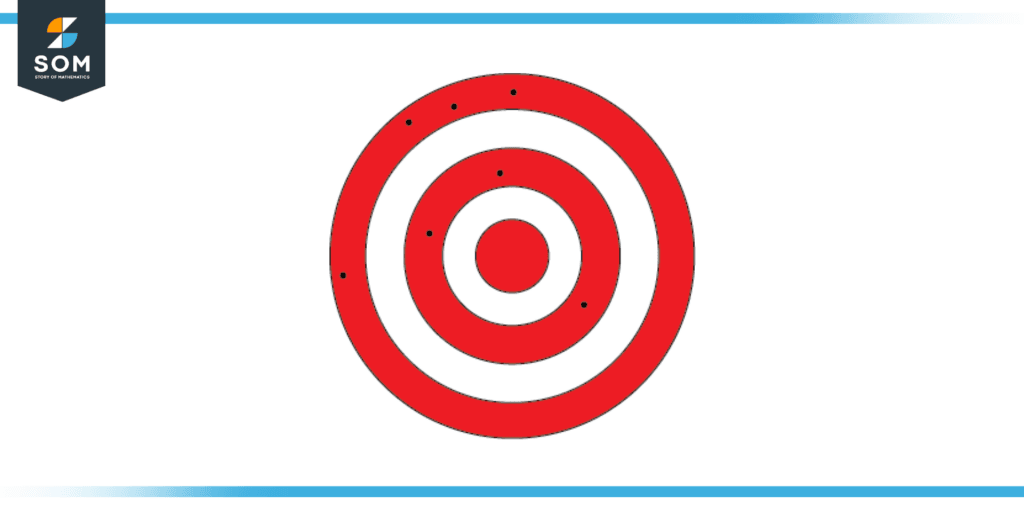
Figure 3: The darts are neither precise nor accurate.
As a result, precision demonstrates how frequently the same measurement would be obtained under identical conditions, while accuracy indicates how near one was to the actual value. Precision is frequently referred to as reproducibility or repeatability by experts.
The more accurate you are, the closer you are to the target value. You must have well-defined goals or performance targets to establish accuracy.
To check for precision, provide a monitoring system that illustrates how numerous findings or statistics compare to one another over time.
In short, precision alludes to the consistency of the answer, whereas accuracy indicates the correctness of the solution.
Importance of Precision in Measurement
Imprecise measurements can frequently lead to rework or even more drastic measures, such as restarting a project entirely. As a result, precise measurement data is one of the main information sets that can help assure a solid project plan throughout the early stages of a project.
To obtain the most consistent results from a scientific investigation, it is critical to reduce bias and errors while also being precise and accurate in data gathering. Precision and accuracy are both concerned with how near a measurement is to its real or actual.
Modern engineering is heavily reliant on precise, convenient measurements in design, building, and communication, and modern measurements rely on quantum standards of frequency, wavelength, voltage, and other parameters.
The highly precise measurements made possible by these techniques have numerous applications, including the study of matter structure, geophysics, and astronomy.
Precision-inscribed components are required in numerous industries to guarantee that electronics and engines function smoothly, that automobiles and aircraft stay where they are supposed to be, that technology aids rather than hinders our lives, and much more.
Measurements Should Be Both Precise and Accurate
The implications of precision and accuracy go hand in hand. A measurement can be precise but inaccurate, and vice versa. We decide based on precision when observing changes in a process. When striving to reach a goal, accuracy is critical.
Suppose a group of students has developed a shared database in order to have access to reporting in a centralized manner and elevate the level of participation.
If they are extremely accurate but not quite as precise, they will create the database but may only notice an increase in collaboration between one or perhaps two projects.
If they are simply concerned with precision, they may add many comparable entries to the database. However, they may not be the ones on which your group needs to work.
If they were to create a database that is neither precise nor accurate, only one person would have access to it, which discourages collaboration.
It it is precise as well as accurate, then they have created a shared database with a slew of projects that are simple to work on as a group. Because the system is reproducible, they may continue to cooperate on many different projects as they arise.
Examples of Precision in Sets of Measurements
Example 1
Person A and person B are both assigned the task of measuring the length of a room five times. When person A measures it, the values are: 10.3 m, 10.4 m, 10.5 m, 10.6 m, and 10.7 m. When person B measures the length, the values are 10.0 m, 10.7 m, 10.9 m, 11,1 m, and 11.3 m.
Which person has the more measurements?
Solution
Let us calculate the average of lengths calculated by both persons.
Person A:
Average length = (10.3 + 10.4 + 10.5 + 10.6 + 10.7) / 5 = 10.5
Person B:
Average length = (10.0 m + 10.7 m + 10.9 m + 11.1 m + 11.3 m) / 5 = 10.8 m
As 10.5 m is closer to the measured values, person A has the more precise measurement values.
Example 2
Sonny and Ally performed an experiment to measure the density of copper (8.96g/mL). They come out with the following results:
Sonny: 8.52, 8.95, 8.68 and 8.21
Ally: 8.81, 8.84, 8.91, and 8.87
Solution
As we can observe from the values, when Ally performed the experiment, the values were close to each other. Hence, we can conclude Ally has measured the precise values.
Example 3
A binary model produces predictions and estimates 250 specimens from a segment, 170 of whom are correct, and 80 are erroneous. Determine the precision of this model.
Solution
Using the following formula, we can calculate the precision of the model:
TP/ (TP + FP)
TP (True Positive) = 170
FP (False Positive) = 80
Precision = 170/170 + 80 = 0.68 or 68%.
All images/mathematical drawings were created with GeoGebra.
