JUMP TO TOPIC
Problem|Definition & Meaning
Definition
A mathematical problem is an unsolved question. These problems usually provide some values and ask to find some unknown value. For example, if you cycled a total distance of 16 km in one hour, then what was your average speed? In other problems, you might have an equation already and need to solve it for the unknown variable e.g., what is the value of x in x + 6 = 21?
Some mathematical Problems include the usage of words, such as, “Jim maintained a speed of 30 kilometers per hour during the entire hour. How much ground did he cover?” Others use equations such as “If x + 19 = 78, what is x?” In this article, we shall talk about them in great detail.
Figure 1 – Problems in Mathematics
Word Problems
Word problems are notoriously difficult for pupils to master. Because there are so many moving parts in the process of solving word problems, it can be difficult to isolate the specific factor that is making things difficult for students.
Types of Word Problems
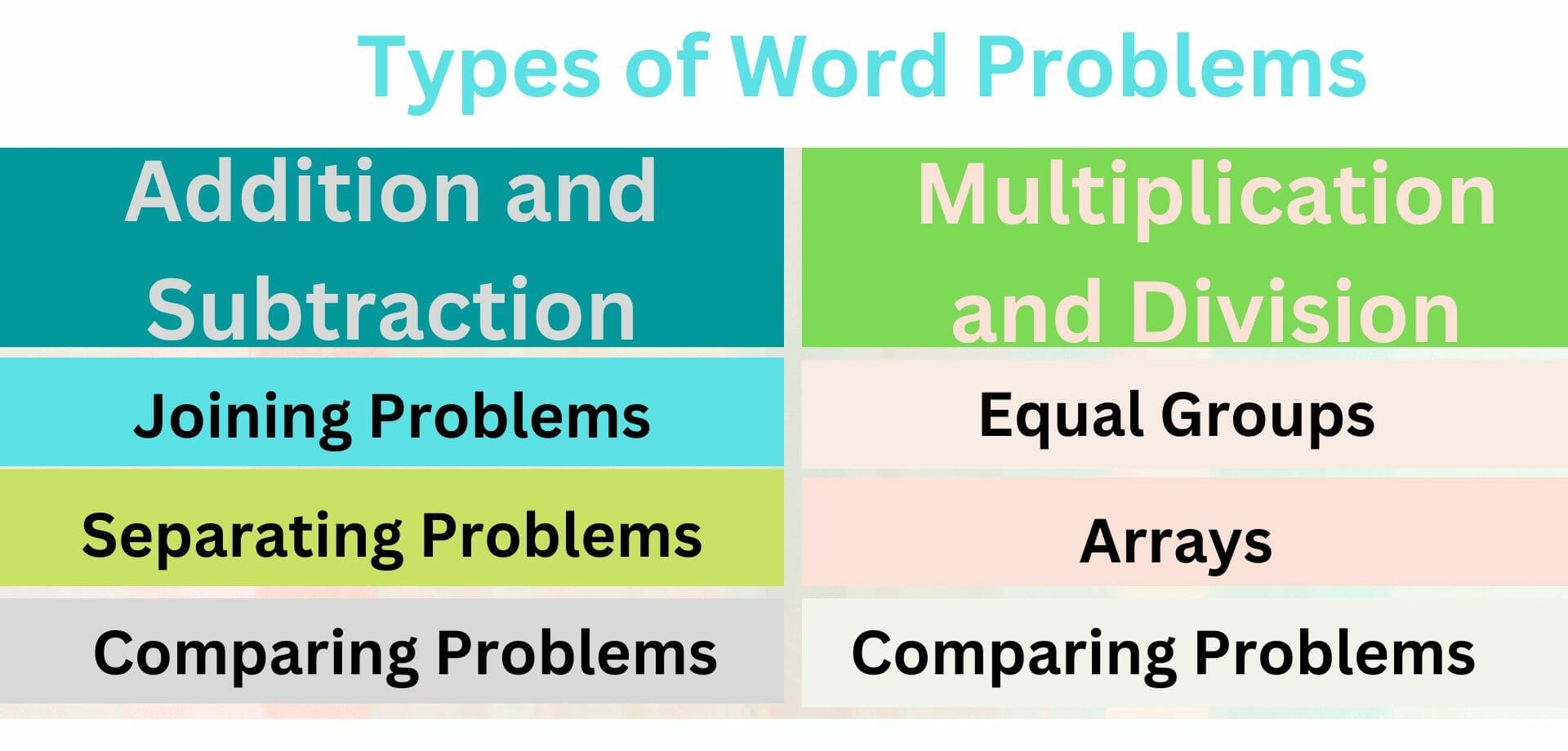
Figure 2 – Types of Word Problems
Addition and Subtraction
There are three primary categories of problems involving addition and subtraction:
- joining problems
- separating problems
- comparing problems
Any problem in which you begin with one quantity, acquire some more, and then finish with a greater quantity is said to be a joining problem.
Any situation in which you begin with one quantity, remove some of that quantity, and then finish up with a smaller quantity is known as a separating problem.
Take, for instance:
- Joining: make sure you have 4 orange slices. Another 5 orange slices were handed to me by my brother. How many orange slices do I still have in my possession right now?
- Separating: Count nine blueberries before you separate them. I eat four of them in total. How many blueberries are there still available?
In each of these scenarios, the end outcome is unknown because we know how much we begin with, we know how much is joined or separated (the change), and now we need to determine how much is left over after the process. Both of these issues may be solved using the following straightforward pattern:
Students need to solve issues in which either the result, the change, or the starting point is unknown.
- Make a tally of the first set.
- Add some of them to the original set, or take some of them away from it.
- Determine the updated total when the event has been completed.
You’ll find that there are innumerable opportunities throughout the day to utilize the terminology of joining and separating! The most obvious of them is eating because your child is always considering joining other children in order to grab more of their food and then consuming that food after it has been obtained (separating).
When you play a game with your child that involves blocks, ask them to count how many are in their tower. Then you should ask them, “Now I’ve added three more blocks to your tower. How many blocks do you currently have on your tower?”
Have your child count the number of cars in a row while they see vehicles parked in a parking lot. Then pose the following question: “If two vehicles depart the parking lot, how many vehicles will be left?”
If your child is having trouble, you shouldn’t give them the solution. Instead, you should assist them in carrying out the action.
“You have 9 blueberries. Now you are going to devour four of them. When you consume those blueberries, what happens to the blueberries? Your kid might remark something along the lines of “They went in my stomach!” And you are able to reply, “Good.” So, those blueberries are still sitting there on your plate, are they?”
They will respond with a negative, at which point you can say, “Ok. So please display those blueberries that are disappearing from your dish. When performing an additive comparison, the problem might have the following forms, where x could be any whole number.
- How much further is it?
- How much less do you want?
- x more
- x less
Multiplication and Division
There are three primary kinds of word problems involving multiplication and division
- Equal groups
- Arrays
- Comparing problems
Equal Groups
When there is the same number of items in two different groups, we refer to such groupings as “equal groups.” Therefore, an equal number of items or things are grouped together in each equal group.
For example: If there are three boxes and you put five candies in each box, then there will be an equal number of candies in each box. At this point, we will assume that there are three equal groupings, each containing five candies.
Array
The operations of multiplication and division can be represented by using rows and columns in an array. The rows denote the number of different categories. The number of items in each category, as well as their respective sizes, are denoted by the columns, although this is not necessarily a strict rule, and the two can be swapped.
It is essential for one to keep in mind that rows, which represent groups, are drawn horizontally, and columns, which represent the number of items in each group, are drawn vertically.
Comparing Problems
When doing a multiplicative comparison, the problem can involve expressions such as where x can be any whole number.
- x multiples as many as that
- x multiplied in comparison to
It is possible that the product, the size of the group, or the number of groups will all be unknown within each type of problem. Once again, we make use of counters to guide the students through the process of selecting the appropriate equation to apply while trying to solve the various kinds of issues.
The difference between issues involving addition and subtraction and problems involving multiplication and division should be brought to the attention of the students.
Students’ thinking and ability to solve problems are considerably improved when they are required to compose word problems in a fashion that is consistent with a certain word problem style. Because this is a considerably more difficult ability, the first time we practice composing word problems, we usually do so under the supervision of an instructor.
Equation-related Mathematical Problems
Equations are used to solve issues, and in order to solve a problem using equations, we must do two things:
- Translate the expression of the question from the common language into the algebraic language to get an equation.
- Reduce this equation so that the unknown quantity will stand by itself, and its value will be stated in terms of known quantities on the opposite side.
One of the most notable characteristics of an algebraic solution is that the quantity that is being sought is incorporated into the very operation that is being performed. Because of this, we are able to construct a statement of the conditions in the same form as if the problem had already been solved.
Figure 3 – Equation Related Mathematical Problems
Nothing else has to be done at this point other than to simplify the equation and determine the total sum of the quantities that are already known. Because they are equivalent to the unknown quantity on the opposite side of the equation, the value of that is likewise determined, which means that the problem has been solved as a result.
Examples of Problems
These examples are quite literally examples of problems and illustrate the process of translating words into equations and then simplifying them to find the value of the unknown variable.
Example 1
When a given integer is divided by 10, the sum of the quotient, dividend, and divisor equals 54. Determine the number that satisfies it.
Solution
Let x equal the desired number. Then:
(x / 10) + x + 10 = 54
x + 10x + 100 = 540
11x = 540 – 100
x = 440/11
x = 40
Example 2
When a deal is made, a particular amount of profit or loss is realized by a merchant. In the second deal, he makes a profit of 250 dollars, but in the third, he loses 50 dollars. In the end, he determines that the three transactions resulted in a profit of one hundred dollars for him. In comparison to the first, how much ground did he make or lose?
Solution
In this particular illustration, the profit and the loss are of opposing characters, so it is necessary to differentiate between them using signs that are the opposite of one another. When the profit is a plus sign (+), the loss should be a minus sign (-).
Let’s say x equals the total amount needed.
The conclusion that follows from this is that x plus 250 minus 50 equals 100.
So, x = -100.
The fact that the answer has a negative sign attached to it demonstrates that there was a loss incurred in the initial transaction; hence, the correct sign for x is also a negative sign. However, because this is dependent on the response, leaving it out of the calculation won’t result in an error at all.
All images were created with GeoGebra.
