JUMP TO TOPIC
Factors of 13: Prime Factorization, Methods, and Examples
A factor of 13 is any integer, smaller or equal to 13, that divides itself into 13 without leaving any remainder. In other words, any two numbers that multiply together to give the product ‘13’ are the factors number 13 as both are divisible by 13.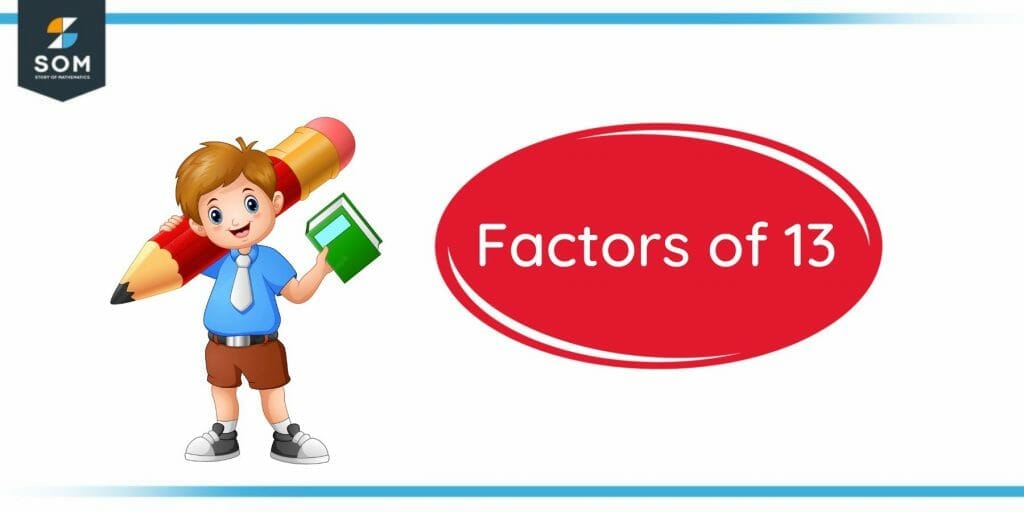
Figure 1 – All possible Factors of 13
What Are the Factors of 13?
The factors of 13 are 1 and 13 only, demonstrating that it is a prime number as only prime numbers have two factors — 1 and the number itself. As a factor is any number, positive or negative, that divides itself with 13, not leaving any remainder. Factors cannot be in decimals or fractions.How To Calculate the factors of 13?
To calculate the factors of 13, two methods can be used: multiplication and division. For finding a factor through division, we will take numbers smaller than or equal to half of 13. Half of 13 is 6.5. Since factors cannot be in decimals or fractions, we will take numbers to 6 and start dividing 13 by them. Any number dividing 13 fully will be the factor of 13 along with the quotient. Since 13 is a prime number, no other number except for one will divide 13 fully by itself. Let’s look deep into this concept for better understanding:Upon dividing 13 by 1, we will get 13 as the quotient, and nothing will be left as the remainder. So we will consider 13 and 1 as our factors. There is no other whole number between 1 and 6.5 that will divide 13 without leaving any remainder. Now about finding factors through multiplication, we will have to find numbers that multiply with each other to give us the product of a given number, which in this case is 13. For this method, you can start with smaller numbers first. Here is an example of this method:1 x 13 = 13
However, for prime numbers like 13, we will only have to multiply 1 with the number itself, as shown in the example above.Both of these methods are easy and reliable as working through them gave us the same factors, 1 and 13.Factors of 13 by Prime Factorization
Prime factorization is a method for finding prime factors of a number. You break a number down into a set of prime numbers, which are multiplied to give the product of your assigned number.This method requires you to start by breaking that number into any divisible numbers until you are left with prime numbers as your final answer. Those prime numbers are your answer.A fact to remember here is that any prime number has only two factors: prime numbers. So in the case of 13, we will divide it by the smallest divisible number, and our answer will be 1 and 13, proving that they are the only prime factors of 13.For our satisfaction, we can divide 13 further, in this method, by other whole numbers between 1 and 6 to see if they divide 13 without leaving any remainder. \[ \frac{13}{2} = 6.5 \]\[ \frac{13}{3} = 4.333.. \]\[ \frac{13}{6} = 2.167 \]Since none of these numbers provided an answer in whole digits, we won’t be able to divide 13 any further, considering 13 as our final answer.You can take an overview of the Prime Factorization of 13 from the diagram attached below: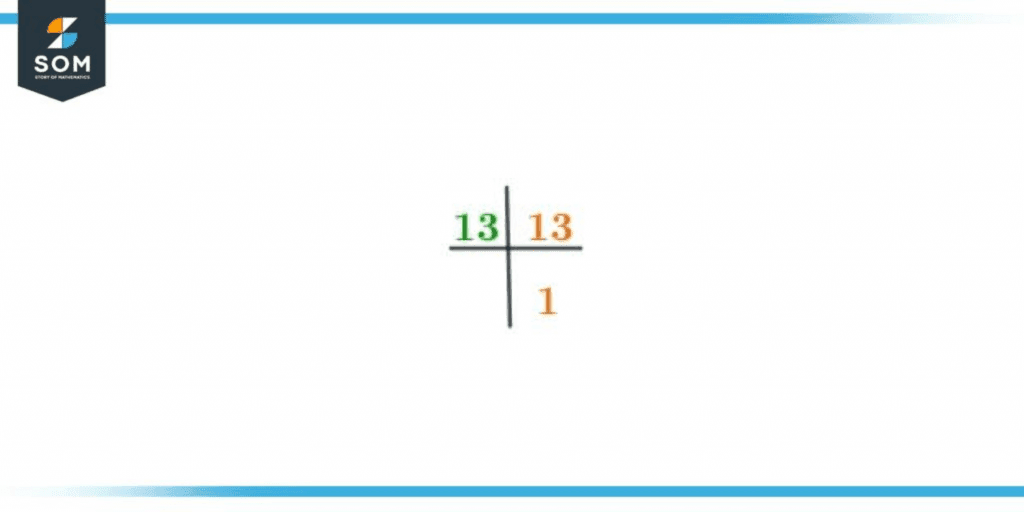
Figure 2 – Prime Factorization of 13
Factor Tree of 13
A factor tree is a method to find out the prime factors of any number, and it is also an excellent way to represent the working of your answer. You can simply keep dividing the given number by numbers thoroughly until you get prime numbers as your final answer.This method is even more straightforward for prime numbers as there are only two numbers that are your answer. For 13, you will have to find a number that breaks it down to its prime factors. Since it’s a prime number, it will be divided by itself to break it into its prime factors, 13 and 1.For making a factor tree of any given number, we will write it on top and start dividing it. We will document our answer by extending branches below the number, then divide those numbers until we don’t get prime numbers as our answer.For the factor tree of 13, we will write 13 on top. Now we have to divide it by any divisible number, giving us results in whole numbers. As it is a prime number, we will divide it by itself, and our answers will be 1 and 13. We will write both of them separately by extending branches below 13. Since both results are prime numbers, our working process would stop here. A factor tree looks like a family tree. You can now visualize an image in your mind combined with the information above for an initial understanding of this topic. Thus, by drawing a factor tree, you can prove the working of your results just as the prime factors of 13 were proved here.You can take a look at the image of the factor tree attached below for a visual representation: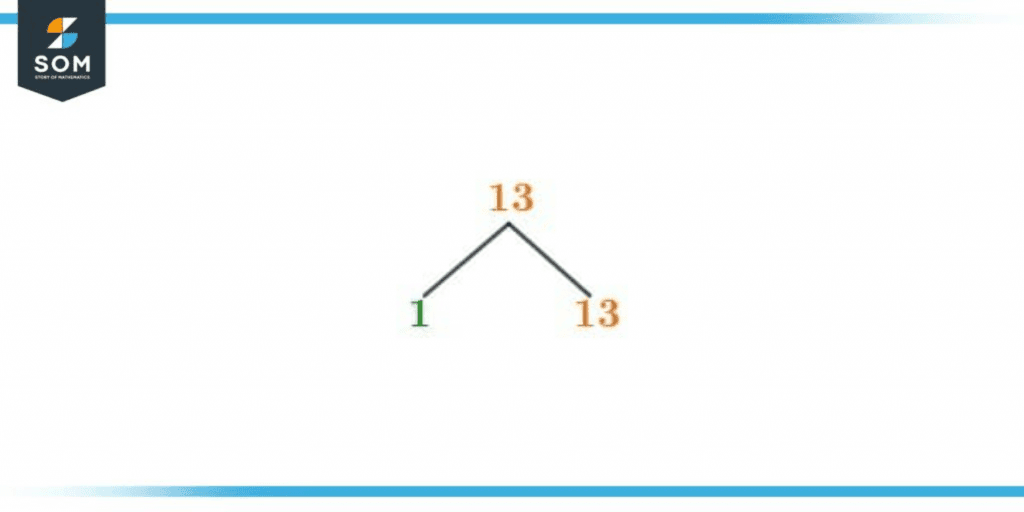
Figure 3 – Factor Tree of 13
Factors of 13 in Pairs
A factor pair is a pair of numbers that multiply together to give you a product of a given number. Both numbers will be the factors of that given numbers separately as well. They can be negative or positive but cannot be in decimals or fractions. As discussed earlier in the article, when factors of a given number are found through division, both the quotient and divisor are considered factors at the end. In this concept of factor pairs, both of those factors are paired together to form a factor pair for a specific number.The first step is determining whether the number is prime or composite. If it’s a composite number, we will have to find all of its factors, and then we will try multiplying different pairs together to find out if their answer gives us the product of our given number or not. All the pairs sharing the desired outcome will be considered factor pair for that specific number. Finding a factor pair of a prime number is easy and takes less time. We already know it only has two factors, and if we multiply both, we will get the given prime number as our product. Thus, both factors are paired together for a prime number to form the only factor pair for the given prime number.An example of this can be seen below:13 x 1 = 13
Factors of 13 Solved Examples
Now that we have gained enough information about the factors of 13, we should go through a few examples to strengthen our concepts.Example 1
How many even numbers are there in the factors of 13?Solution
To answer this, we will write all of the factors of 13 then search for any even numbers. So the factors of 13 are 1 and 13. Both of them are odd numbers, so the answer to this question is 0.Example 2
List the factors 11 and 13. Also, write down any common elements shared between them.Solution
We will again make a list of factors for both numbers. This will answer the first half of our question, and for the second half, we will look for any standard number in both the list of their factors.Factors of 11: 1 and 11
Factors of 13: 1 and 13
1 is the common factor between both of them. A fact to remember is that 1 is the factor of all numbers. It will always be a common factor between the factors of any two numbers.All images/mathematical drawings are used using GeoGebra.