Equidistant|Definition & Meaning

Overview 

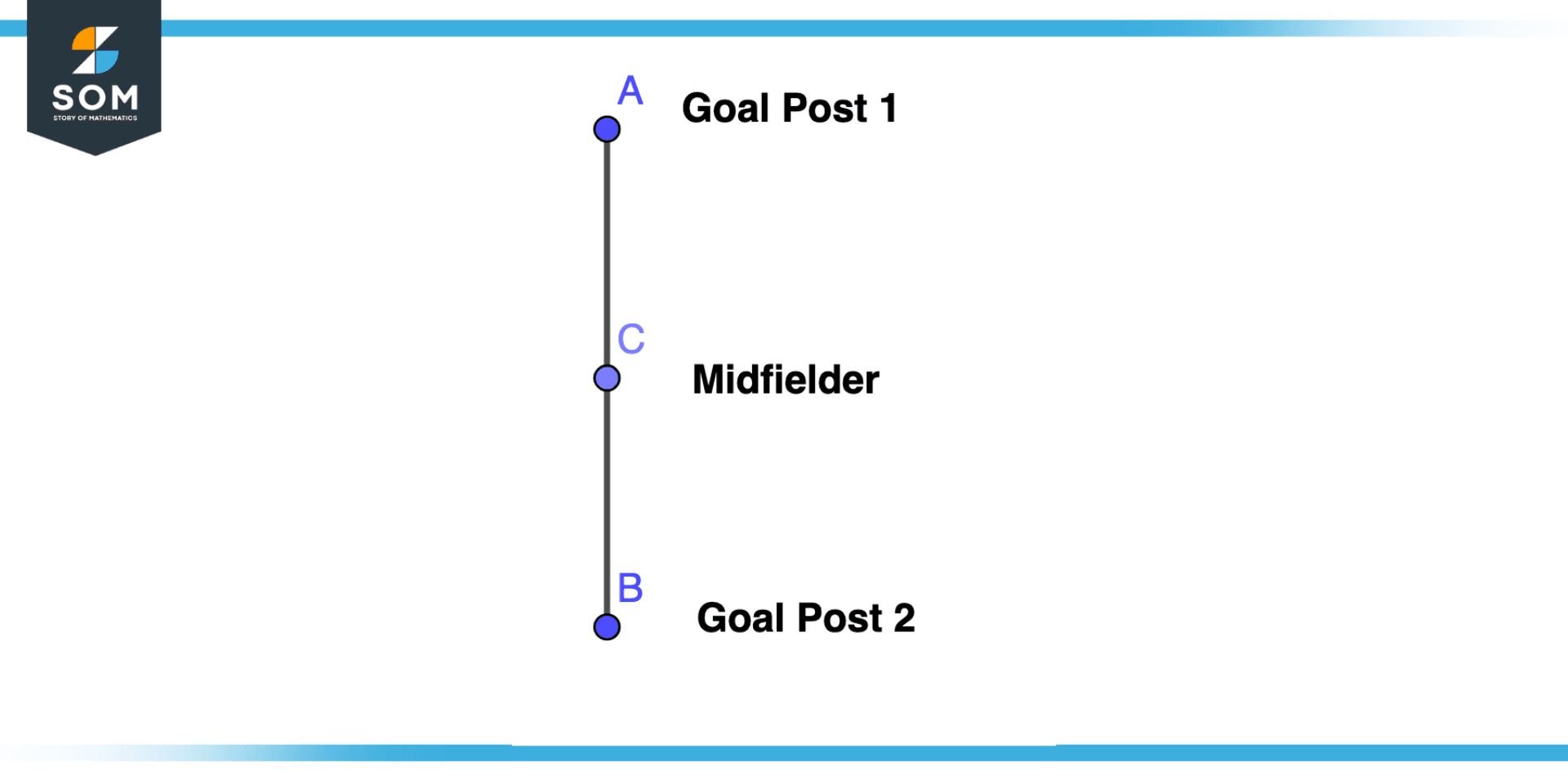
Figure 1 – Midfielder Illustration for the equidistant concept

Suppose a scenario of a football ground where many players have been assigned some positions as part of the lineup. Take the example of a midfielder; the midfielder position is assigned in the middle of the ground from the goal post. So we can say that the midfielder is an equidistant point that has to be in center from both the goal post. This concept is elaborated on in the above figure.

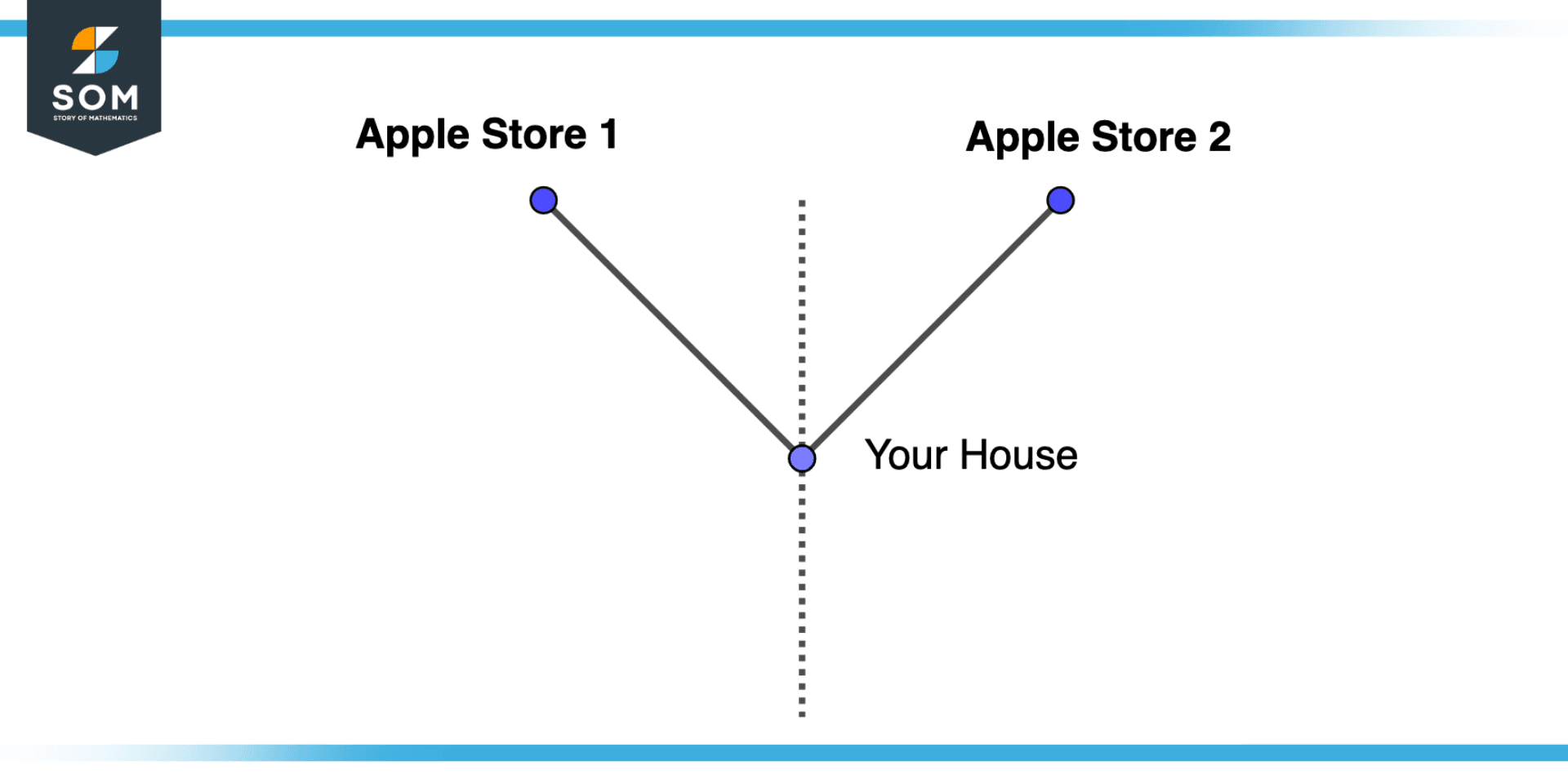
Figure 2 – Distance Between House and Apple Store for equidistant Concept

Suppose another scenario where your house is located near two Apple stores. The distance from one of the apple stores to your house is 5Km and the distance from the second Apple store to your house is also 5 km, but it is not mandatory that your house will be in the middle of both stores. Your house might be at perpendicular bisector of both the stores. This scenario is elaborated on in the above figure.

Equidistant in Triangle and Polygon

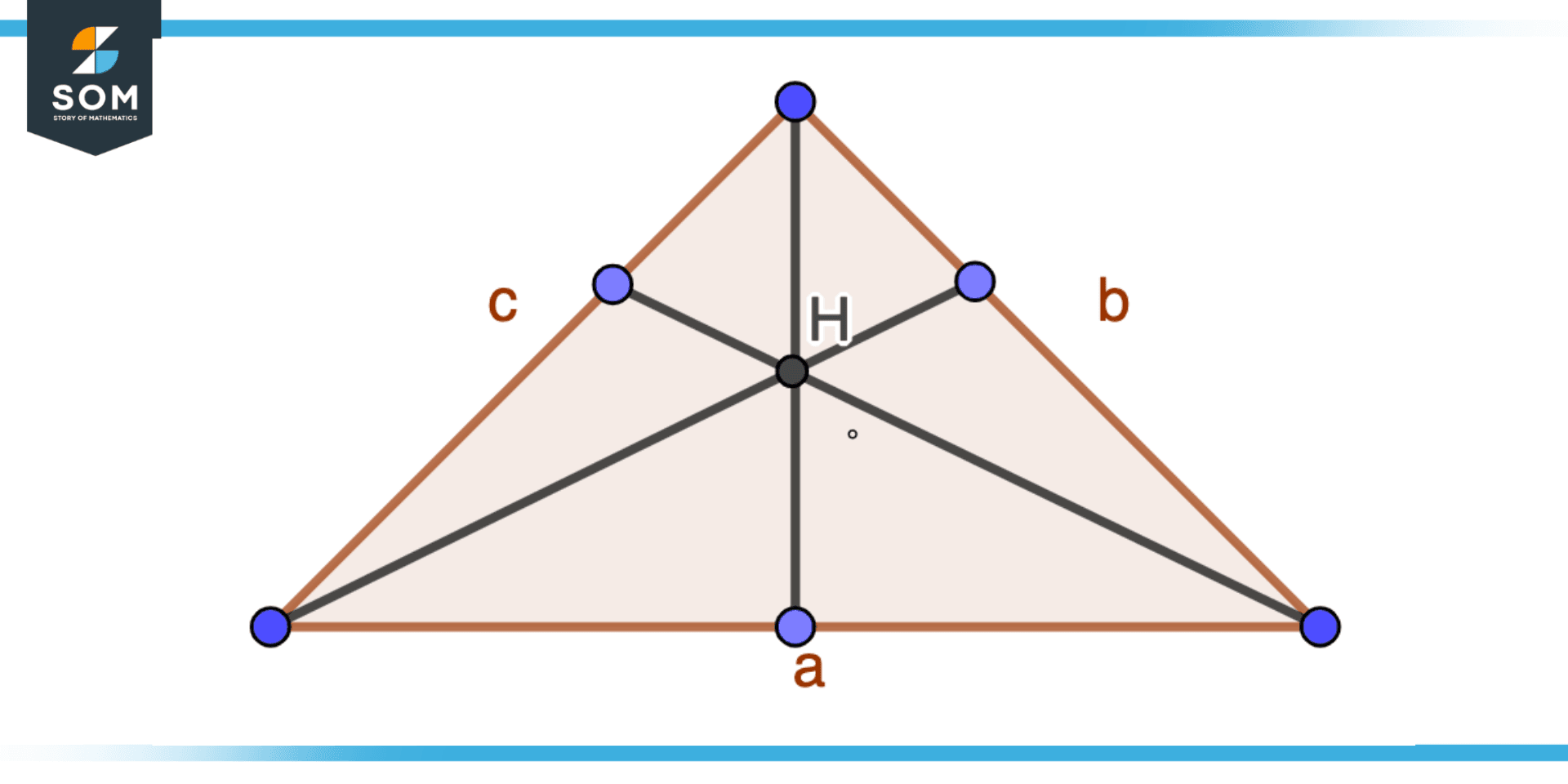
Figure 3 – Circumcenter of Triangle Equidistant from its vertices

Typically, the circumcenter of a triangle is a point that is equally distant from each vertex. There is always a point at the center of a non-degenerate triangle.

Likewise, cyclic polygons have equidistant circumcentres: each vertex is equally distant from its circumcentre. Triangles and other tangential polygons have an incentre equidistant from each of their points of tangency with the circle.

Whenever a perpendicular bisector connects two vertices at the ends of a triangle or other polygon, every point is equidistant from them. Angles have bisectors where the points that emanate from them are equidistant from each other.

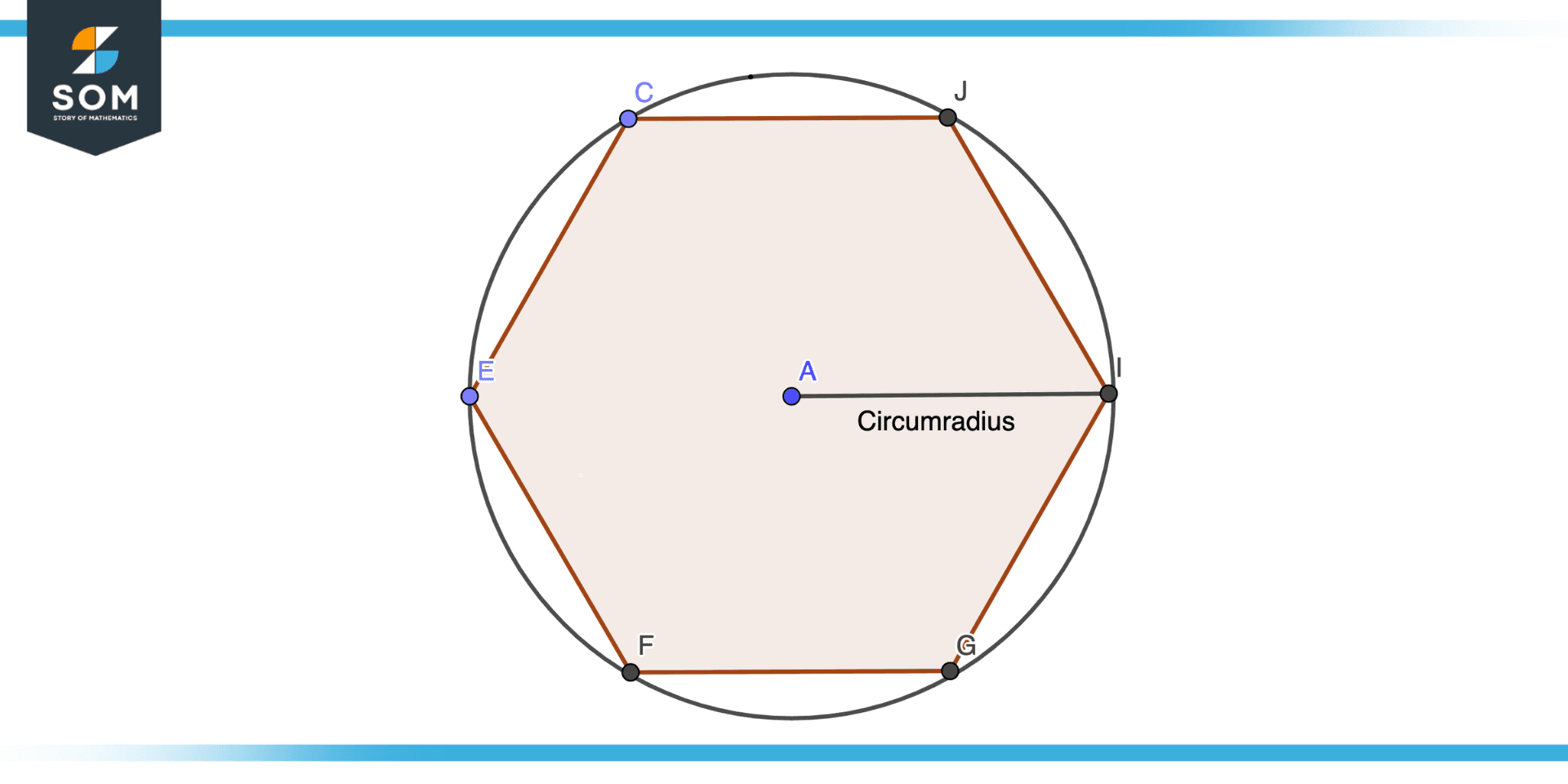
Figure 4 – Center A of Regular Polygon Equidistant from its vertices

Equidistant in Rectangle

All four vertices of a rectangle are equidistant from the center, as are two opposite sides, and two other opposite sides are equidistant from the center as well. 

Equidistant in Circle and Sphere

There is an equidistant line connecting the center of a circle to the center of every point on the circle, which implies that the center of the circle is equidistant. The same is the case for the sphere in which its center is equidistant from each point on the surface.

Equidistant Parabola

Parabolas are lines perpendicular to the directrix where distances between them are determined along a line perpendicular to the directrix, which is equidistant from the focal point and directrix, respectively.

Equidistant Parallel Lines

Parallel lines are defined as lines that are infinite lines and never intersect each other. Consider the two parallel lines then, according to euclidean geometry, any point lying on the first parallel line is equidistant from the point lying on the second parallel line because the distance from a point, say p1, lying on line L1 to the point p2 lying on line L2 is same.

Equidistant Points Formula

Under the topic of equidistant, there are two main formulas. Distance formulas are used to determine the distance between any two points, and line segment midpoint formulas are used to determine line segments’ midpoints.

Distance Formula

The length of the line connecting two points is the distance between them. Distance between two points can be obtained by subtracting the coordinates that don’t match if the horizontal or vertical line is the same. Due to the fact that Pythagorean distances are calculated from Cartesian coordinates, they are sometimes called Pythagorean distances.Consider the points p1, p2, p3 and p4. We can write the distance formula for these points as follows:

d = $\sqrt{(p3-p1)^{2}+(p4-p2)^{2}}$

Midpoint Formula

Midpoints are the points in the middle of line segments in geometry. There is an equal distance between the two endpoints, making it the centroid of both segments and of the ends. Basically, it cuts the segment in half. Consider coordinate pairs (a1,b1) and (a2,b2), which represent the end of the line segments. We can write the formula for the midpoint as follows:

M = $\dfrac{(a1+a2)}{2},\dfrac{(b1+b2)}{2}$

Properties of Equidistant

1. Two points are considered equidistant if they have the same distance from a specific location which is usually said to be the midpoint.
2. Conversely, the midpoint(center of the two points) is equidistant from that point which shares an equal distance from the midpoint.
3. Generally, there are only two techniques for determining if the given set of points is equidistant: the Midpoint and Euclidean distance formula.

Steps for Checking Equidistant Criteria

Step 1

In order to find whether the points are equidistant or not, first of all, mark three things. Coordinates of the midpoint, coordinates of two points that we want to find whether they are equidistant.

Step 2

After finding the coordinates of two points and the coordinates of the midpoint next step is to give the names of each coordinate. For instance, for point 1, we can give names x1 and y1. For point 2, we can give names x2 and y2 and for the midpoint, we can give names Cx3 and Cy3.

Step 3

After naming each coordinate, the next step is to decide which formula to apply. Either use the distance formula or the midpoint formula as described above. If we want to prove that the center of a line segment is equidistant from the endpoints, then we will apply the midpoint formula, and if we want to prove that endpoints are equidistant from the midpoint or any other point, we will apply the distance formula.

Examples of Finding Distances Between Points

Example 1

Consider a line segment L1 having two points (1,2) and (4,5). Find the distance between the points.

Solution

(1, 2) = (p1, p2)

(4, 5) = (p3, p4)

d = $\sqrt{(p3-p1)^{2}+(p4-p2)^{2}}$

d = $\sqrt{(4-1)^{2}+(5-2)^{2}}$

d = $\sqrt{(3)^{2}+(3)^{2}}$

d = $\sqrt{(9+9)}$

d = $\sqrt{18}$

d = 4.242

Example 2

Consider the endpoints of the line segment as (-5,4) and (1,-2). Find the midpoint that is equidistant from the two points.

Solution

(a1, b1) = (-5, 4)

(a2, b2) = (1, -2)

M = $\dfrac{(a1+a2)}{2},\dfrac{(b1+b2)}{2}$

M = $\dfrac{(-5+4)}{2},\dfrac{(4-2)}{2}$

M = $\dfrac{-1}{2},\dfrac{2}{2}$

M = (-0.5, 1)

All mathematical drawings and images were created with GeoGebra.

Equiangular Triangle Definition < Glossary Index > Equilateral Definition