JUMP TO TOPIC
Septagon|Definition & Meaning
Definition
A septagon is a polygon with exactly seven sides. It may be regular (all sides and angles equal) or irregular (if any side or angle is not equal to the others). Septagons are also called septagons, where “sept” comes from septem and septua- in Latin, and “hept” from hepta in Greek. Septem means “seven,” and the other two are numerical prefixes for “seven.”
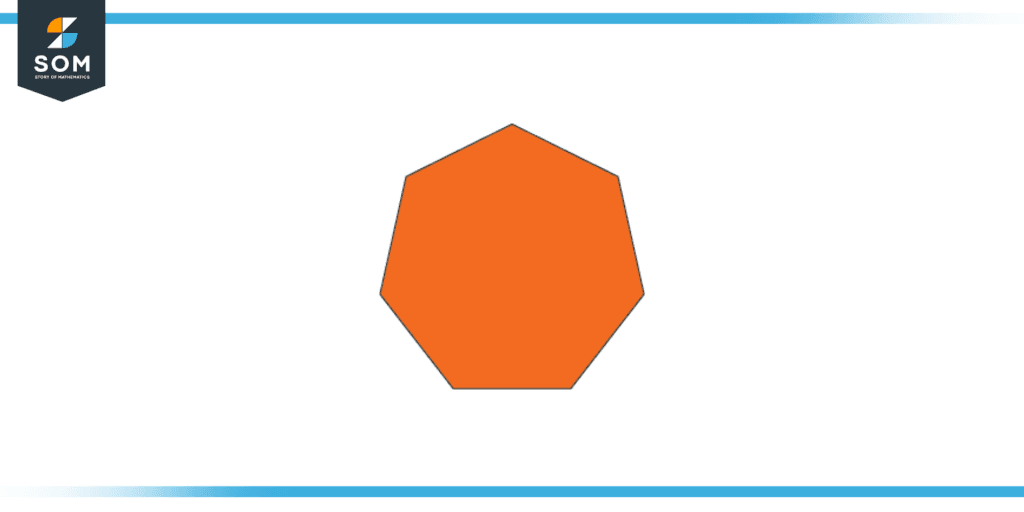
Figure 1: A regular septagon
A polygon is a shape in geometry that has at least three inner angles or straight edges surrounding it. They have no curves and are closed shapes constructed of straight lines.
The most prevalent polygons are:
- Triangle (3-sided polygon)
- Quadrilateral (4-sided polygon)
- Pentagon (5-sided polygon)
- Hexagon (6-sided polygon)
- septagon (7-sided polygon)
- Octagon (8-sided polygon) and so forth
A polygon is a closed, two-dimensional form with any number of straight sides. To put it simply, a septagon is a polygon with seven sides.
Septagon Sides
The lengths of the seven straight edges that make up a septagon’s seven sides might vary or remain constant. These sides come together but do not overlap or intersect. A seven-sided enclosed polygon is created when the septagon’s sides converge at its vertices.
Septagon Angles
Seven interior angles make up a septagon, and the total of all seven interior angles is 900°. There are some obtuse and sharp angles in the figure. Septagons can be regular or asymmetrical, and both types of septagons have a sum of outside angles that is equal to 360°.
Now let us examine more about the septagon’s interior and exterior angles.
Interior Angles of a Regular Septagon
The interior angle formula, which is (n – 2) 180º where n is the number of sides in the polygon, can be used to calculate the sum of interior angles of a regular polygon. Thus, n = 7 for a septagon. A regular septagon’s interior angles are calculated as (7 – 2) 180° = 900°. A regular septagon’s interior angles are therefore equal to 900/7, or 128.57º
Exterior Angles of a Regular Septagon
The formula for the sum of exterior angles states that a regular polygon’s total exterior angle is 360 degrees. As a result, the total of all the outside angles of a standard septagon is 360 degrees. A regular septagon’s exterior angles are, therefore, equal to 360/7, or 51.43 degrees.
Septagon Diagonals
Fourteen diagonals make up a septagon. As opposed to a concave septagon, which has at minimum one diagonal outside the figure, a convex septagon has diagonals that are inside the figure.
Types of Shape of Septagon
The sides and angles of a septagon can be used to classify the shape.
Septagons can be categorized into the following groups according to their side lengths:
Regular Septagon
A septagon with equal sides and angles is referred to as a regular septagon. The total internal angle of a polygon, where n is the number of sides, is equal to (n – 2) 180°. The sum of a septagon’s interior angles is (7 – 2) 180° = 5 x 180° = 900° because it has seven sides. A regular septagon has an internal angle value of 900°/7 = 128.57°.
Irregular Septagon
A septagon is said to be irregular if its sides and angles are of varied sizes. An irregular septagon will have different values for each of its internal angles. But an irregular septagon’s internal angles added together equal 900°.
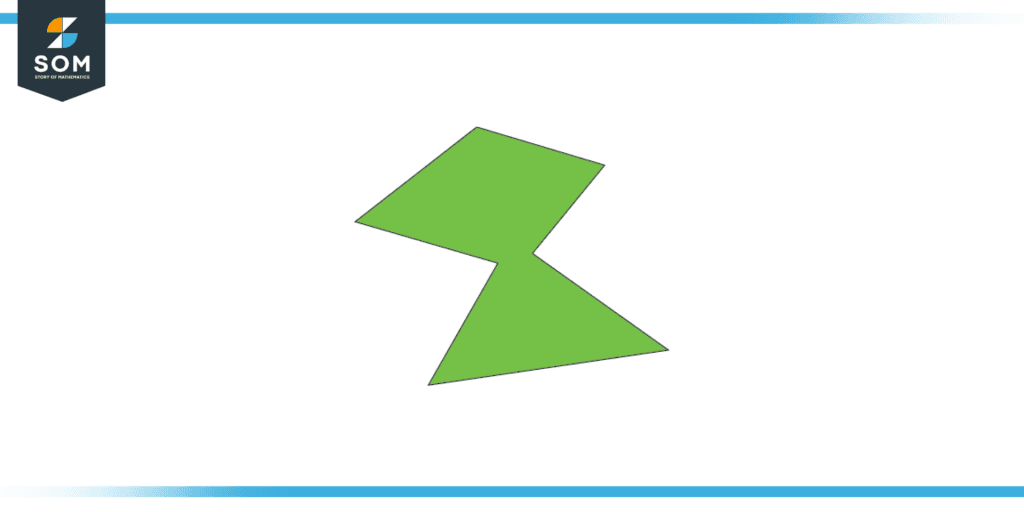
Figure 2: An irregular septagon
Septagons can be categorized in the following ways based on angle measurements:
Convex Septagon
All of the inner angles in a convex septagon are fewer than 180 degrees. They might be septagons that are regular or irregular. The convex septagon’s vertices are all inclined toward the outside.
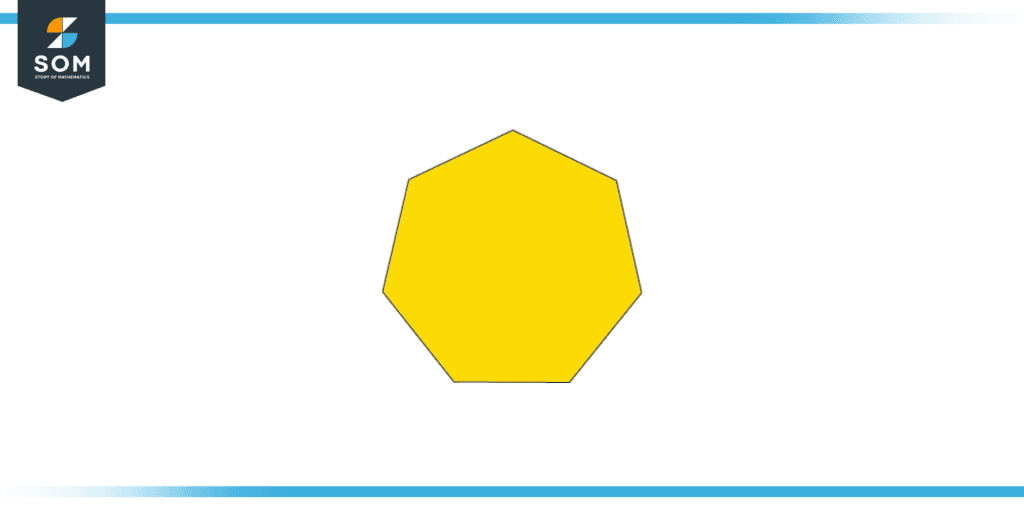
Figure 3: A convex septagon
Concave Septagon
A concave septagon has at least one internal angle that is more than 180 degrees. They might be septagons that are regular or irregular. In a concave septagon, at least one vertex faces inward.
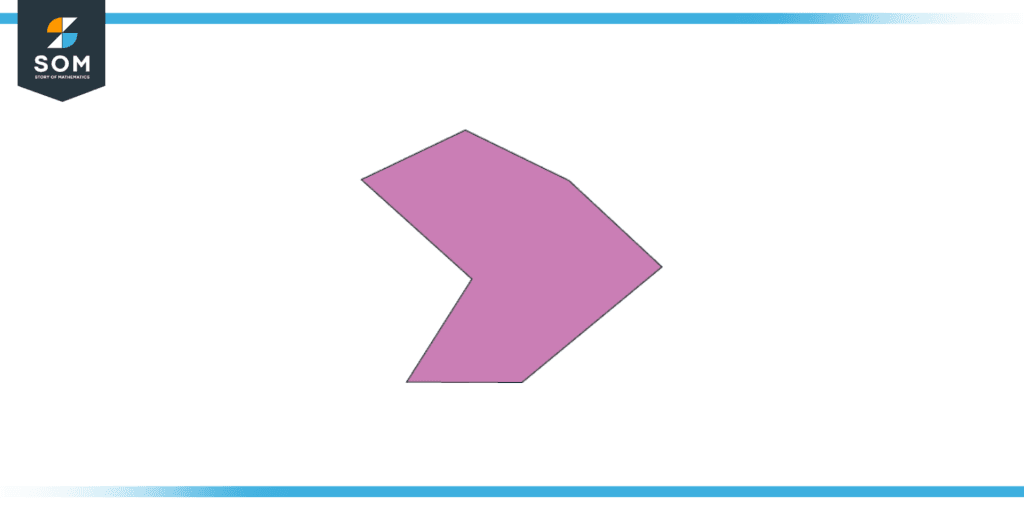
Figure 4: A concave septagon
Properties of a Septagon
We now have a fundamental understanding of what a septagon is, so let’s examine some of its key characteristics.
- Seven sides, seven edges, and seven vertices make up a septagon.
- A septagon’s internal angles add up to 900 degrees.
- A regular septagon has interior angles with values of 128.57° on each side.
- A septagon’s exterior angles add up to 360 degrees.
- A septagon can have a maximum of 14 diagonal lines.
- A typical septagon has a central angle that is 51.43 degrees or so in diameter.
- Since all of the interior angles of a regular septagon are fewer than 180 degrees, it is often referred to as a convex septagon.
Regular Septagon Formulas
A regular septagon can be represented using a variety of formulas. Let’s explore how to calculate a normal septagon’s area and perimeter using the septagon formulas.
Perimeter of a Septagon
We are aware that a typical septagon has seven equal-length sides. As a result, the normal septagon’s perimeter is stated as 7 side length. By using this concept, the perimeter of a regular septagon having side length “a” is given as
Perimeter = 7a
Area of a Septagon
The overall area encompassed by a septagon is referred to as the polygon’s area. The following equation can be used to compute the area of a regular septagon with side length “a”:
Area = (7a^2/4) cot (π/7)
It is possible to write this equation more concisely as:
Area = 3.634a^2
where “a” stands for the side length. This can be used to determine the size of a standard septagon.
Summary of a Septagon
The following points summarize the characteristics of a septagon.
- There are 7 vertices, 7 interior angles, and 7 edges in a septagon.
- The septagon’s exterior angles add up to 360° while its interior angles add up to 900°.
- The number of sides distinguishes between two types of septagons: regular and irregular. While on the basis of angles, it is classified into convex and concave septagons.
Examples Involving the Formulas of Septagon
Example 1
A regular septagon has a 52 cm perimeter. How far does each side extend?
Solution
Considering that a regular septagon’s perimeter is 52 cm. We are aware that a regular septagon’s perimeter is provided as 7 Side length. Let ‘a’ units be the side length.
Thus:
7a = 52
a = 52/7
= 7.42 cm
Consequently, the septagon’s length is 7.42 cm on each side.
Example 2
Calculate the area of a regular septagon with 13 cm of each side length.
Solution
Given that a regular septagon has sides that are 13 cm long, we are aware that the formula can be used to get the area of a regular septagon:
Area = 3.634a^2
= 3.634(13)^2
= 568.516 square cm.
Resultantly, 568.516 square cm is equal to the area of a regular septagon with a side length of 13 cm.
All images/mathematical drawings were created with GeoGebra.
