Standard Form|Definition & Meaning

Figure 1 – Basic division of Standard Form

The simplest form of decimal numbers is a standard form, which is easily readable and writable. As an example, the decimal number 0.007 has the standard form 7 × 10^-3. Very large or very small numbers can sometimes be difficult to read or write. It is possible to write any number in standard form, not only decimals. 

Numbers in Their Standard Form

In Maths, the standard form of a number refers to the illustration of small numbers or large numbers in terms of mathematical expression. The standard form of these numbers is represented with the help of exponents. A better way to understand standard form would be to explain it in terms of decimal numbers along with certain rules that must be followed.

A decimal number, as we all know, is the simplest form of a fraction that can be expressed as a number. A fraction can give a decimal number that follows a decimal by a thousandth, a hundredth, or a tenth. In some cases, however, fractions give a large decimal number.

There are several ways to represent large numbers that can be expressed in simple forms, also called scientific notation, which allows us to represent them easily. It’s the standard way to represent rational numbers. A rational number can be represented as p/q, where p and q are integers. For example, 3/2, 7/11, 9/5 etc.

Standard Form of Writing a Number

To write a number in the standard form, you need to follow the following steps

• The first step is to write down the first number from the number that has been given.
• After the first number, make sure to add the decimal point.
• Count the number of digits after the first number on the given number and write them as the power of 10.
• For example, 273000000000 is a number. A number is thus written in standard form as follows
• Here, in this example 2 is the first number
• Add a decimal point after the number 2, it becomes 2.
• There are 10 digits after 2.
• Therefore, to express 273000000000 in the standard form, it would then be 73×10$^10$.

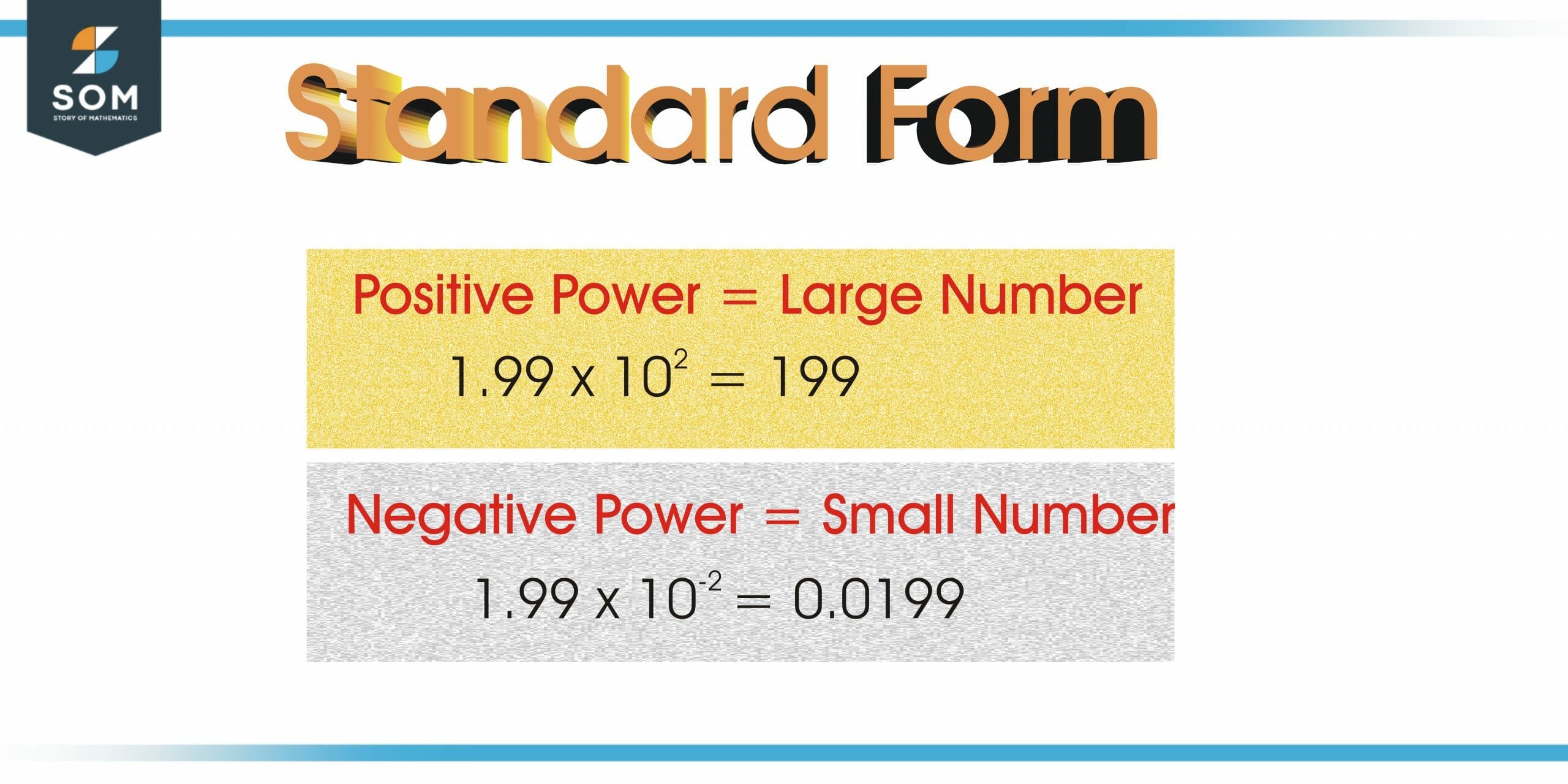
Figure 2 – Positive and Negative Powers in scientific notation

Standard Form for Writing Decimal Numbers

A decimal number is one in which the base number is 10, and for its representation, a dot, as well as 10 different numerals, are required. It is important to note that in this system, the digits that are used to represent the number have different place values depending on the position.

For example, the number 342.341 can be written as 3 hundredths 4 tenths, 2 ones 3 tenths 3 hundredths 1 thousandth.

Here is a brief overview of how decimal numbers are written in standard form.

• From the given number, write the first non-zero
• Then add the decimal point after that digit.
• In the final step, we must find out what decimal point is shifting in the given number and then write that decimal point to the power of ten.

For example, the standard form of 0.00000023 is as follows

• The first non-zero digit in this example is 2.
• Add the decimal point after 2. Thus, it becomes “2.”
• This is followed by shifting the decimal point to the right by seven places.
• Therefore, the standard form of 00000023 is 2.3×10^-6.

How To Write the Numbers in Expanded Form

Let us consider the number 3457684, which has 7 digits. This number can be expressed in expanded form as follows:

3457684 = 3 × 1000000 + 4 × 100000 + 5 × 10000 + 7 × 1000 + 6 × 100 + 8 × 10 + 1

Expanded Form of Decimal Numbers

It is possible to write decimal numbers in expanded form, just like whole numbers. Multiplying digits with increasing exponents of 1/10 or 10^-1 is the way to convert decimals to expanded form.

Here is an example that will help us understand:

0.845 = 8 × 10 – 2 + 4 × 10 – 4 + 5 × 10 – 5

= 8× 1/10 + 4 × 1/100 + 5 × 1/1000

= 0.8 + 0.04 + 0.005

A Rational Number in Its Standard Form

The standard form of a rational number ‘p/q’ is based on two conditions. Firstly, there has to be a positive denominator, q, and both constants, a and b, must not be divisible by any other integer than 1.

A rational number can be converted into its standard form by following the steps below.

• We need to check whether the denominator is positive or negative. If it is negative, then both the numerator and the denominator must be multiplied by -1 to make the denominator positive.
• To cancel the numerator and denominator, find their greatest common divisor (GCD).
• The numerator and denominator will be divided by GCD to get the result.
• The result that is obtained is the rational number in its standard form.

For example, write -4/14 in standard form. The GCD, in this case, is 2, so the answer becomes -2/7.

An Equation in Standard Form

An equation has the following “Standard Form:”

a certain expression = 0

Hence, “= 0” appears on the right, whereas everything else appears on the left.

For example, what is the standard form of x²=6?

= x² – 6 = 0

Polynomials in Standard Form

A polynomial is written in Standard Form by starting with the terms with the highest degree first (like “3” in y³ if there is only one variable).

For example, what is the standard form of 2x² – 5 + 3x³ + x^4?

= x⁴ + 3x³ + 2x² – 5

4 is the highest degree, so it comes first, followed by 3, 2, and then the constant at last.

A Few Solved Examples

Figure 3 – Tips for writing in the standard form

Example 1

Express 4300000000000 in scientific notation.

Solution

There are exactly 11 zeros at the end of this number, so we simply put a decimal after the first non-zero digit (4 in this case), put the following non-zero digits after it (3 in this case), and then multiply by 10 raised to a power of 11. That is:

4300000000000 = 4.3 × 10¹¹

Example 2

a) Express 0.00000045 in standard form.

b) What is the standard form of 90000 + 6000 + 200 + 10 + 1?

Solution

Part (a): We have the number 0.00000045. In order to move the decimal right after the first non-zero digit (4 in this case), we must multiply by 10 exactly 7 times. To get back the actual number, we need to divide by 10 exactly 7 times, so we write it as:

0.00000045 = 4.5 × 10^-7

Part (b): 9 ten thousands, 6 thousands, 2 hundreds, 1 tens, and 1 unit adds up to give the number:

90000 + 6000 + 200 + 10 + 1 = 96211

Example 3

a) Write 4x⁴ + 3x² – 2 + x³ in standard form.

b) Write -8/18 in standard form

Solution

Part (a): 4 is the highest degree, so it comes first, followed by 3, 2, and then the constant 2 at the end.

= 4x⁴ + x³ + 3x² + 2

Part (b): The denominator is positive in this case. 2 is GCF in this case, so we will multiply the numerator and denominator with 2.

= -4/9

All mathematical images are generated using GeoGebra.

Standard Deviation Definition < Glossary Index > Standard Notation Definition