JUMP TO TOPIC
Whole|Defintion & Meaning
Definition
To be “whole” means to be complete. In that sense, an un-sliced cake is a whole cake. We write a whole as a non-fraction which provides us the set of whole numbers 0, 1, 2, 3, …, where 0 means “none” of something, and the numbers from 1 and onwards represent an exact number of whole items.
Figure 1 – Examples of Whole cake and Pizza
In mathematics, an undivided and unbroken quantity is referred to as “whole,” and the term “whole” is used in this context. The concept that a quantity represents the entire amount of something and does not contain any fractional or decimal parts is what this phrase is referring to.
A whole is believed to be an entire unit, in contrast to a fraction, which is a representation of a portion of a whole. In mathematics, an illustration of the idea of a whole can be seen in the concept of whole numbers, which are positive integers that are greater than zero.
The relevance of whole numbers can be found in the roles they play in the various arithmetic operations as well as the contributions they make to the study of number theory. let us look into what whole numbers are.
Whole Number
The set of numbers known as whole numbers consists of all the natural numbers as well as 0. Real numbers are those numbers that do not include fractions, decimals, or negative values. They are a portion of real numbers. The numbers used for counting are also regarded to be whole numbers.
Natural numbers, in addition to the digit zero (0), make up what are known as whole numbers. It is common knowledge that the term “natural numbers” refers to a group of counting numbers that begin with 1 and continue on through 1, 2, 3, 4, and so on.
To put it another way, a collection of numbers is said to be “whole” if it does not contain any fractions, decimals, or even negative integers. It consists of positive integers and the value zero in its entirety. Or, we may say that the set of all integers that are not negative is the set of whole numbers.
The addition of zero to the set of whole numbers is the key characteristic that distinguishes natural numbers from whole numbers.
A complete sum is an integer that has a value that is both 0 and larger than 0. Zero, one, two, three, and four were the first five numbers in the total range. They are all infinite in their scope.
The sets of natural numbers and the whole numbers are essentially identical to one another, with the exception of 0. Because integers can take on any value, from a negative infinity to a positive infinity, complete whole numbers are considered to be a subset of integers.
The entire number set is described by utilizing the basic mathematical definition of a set W = {0, 1, 2, 3,…..} as a collection of objects that share a well-defined attribute. The creation of the element x of the set begins with the number 0 and continues with the addition of the number that came before x to the number that came before that, which is x minus 1.
The use of an ellipsis (…) suggests that the total number of entries in the set is infinite rather than finite (i.e., infinite).
Every number x, with the exception of 0, has exactly one immediate predecessor, which is the amount that comes before x. 0 is an exception to this rule. Every number y has precisely one immediate counterpart, and that counterpart is the number that comes immediately after y.
It is noteworthy to note that the full set of numbers does not contain a number that is the greatest of all the whole numbers. Let’s say b is the greatest whole number, in which case b plus one is also a whole number. Nevertheless, b plus one is larger than b by itself. This approach indicates that you can always find a bigger whole number.
Symbol for Whole Numbers
Whole numbers are denoted by the capital letter W, which stands for zero, one, two, three, four, five, six, seven, eight, nine, ten, and so on.
Smallest Whole Number
The number 0 is the smallest whole number. There are no other whole numbers smaller than 0. According to the definition of a whole number, a whole number is a number that starts at 0 and goes all the way up to infinity. Because of this, 0 is now the smallest whole number that can ever be found. Zero is neither positive nor negative; rather, it serves as a stand-in for other values.
Largest Whole Number
There is no such thing as the largest whole number because every number has a number that comes after it. As a result, there is no such thing as the greatest whole number.
You won’t have any trouble locating the smallest Whole number, but you won’t be able to locate the largest Whole number because every number has a number that comes after it. Therefore, you are only able to locate the smallest whole number, but not the highest whole number possible.
Whole Numbers on the Number Line
Because a number line, in the general case, depicts integers from negative values to positive numbers, and whole numbers are positive integers that include zero.
It is simple to spot entire numbers on a number line. When looking at a number line, it is possible to see that the integers that are on the right side of 0 include 0 and are, therefore, whole numbers, whereas the integers that lie on the right side of 0 and do not include 0 are natural numbers and do not include 0.
You can use the following graphical depiction of the number line to understand the conclusion that all natural numbers are whole numbers, but not vice versa (0 is not part of the set of natural numbers).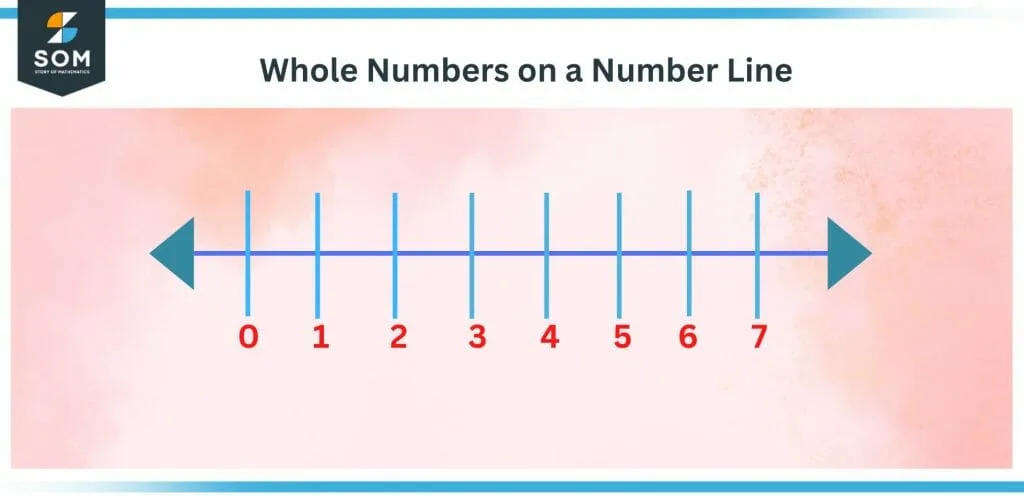 
Figure 2 – Whole Numbers on a Number Line
Whole Numbers and Natural Numbers
We are now knowledgeable about natural numbers as well as whole numbers. Because the set of natural numbers is a subset of the whole numbers’ set, and the set of whole numbers is the natural’s numbers’ proper superset, it is easy to see from the number line that all natural numbers are also whole numbers.
The picture that follows demonstrates how natural numbers and whole numbers are connected to one another.
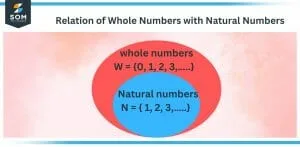
Figure 3 – Relation of Whole Numbers with Natural Numbers
The Properties of Whole Numbers
The features of Whole numbers are derived from a variety of mathematical operations, including adding, subtracting, multiplying, and dividing. Two whole numbers can make up a complete number on their own if they are added together, removed from one another, or combined.
As a consequence of this, we can also obtain a fraction by using the method of division. The following is a list of characteristics shared by whole numbers.
Closure Property
When you add the two whole numbers together, you get another whole number. Therefore, if x and y are both whole numbers, then the product of the two, x times y, or x plus y, is also a whole number.
Commutative Property of Addition and Multiplication
The sum and product of any two whole numbers are always the same, regardless of the order in which they are added or multiplied. For example, if X and Y are two whole numbers, then X + Y = Y + X and X . Y = Y . X. Therefore, it does not matter if you add or multiply X to Y or Y to X; the answer remains the same.
Additive Identity
It is possible to rewrite the equation to read as follows: X + 0= 0 + X when x is a whole number. This is because the value of 0 does not change when it is added to a whole number.
Associative Property
Whole numbers can be added together or multiplied in any order, and the result will always be the same. Suppose x, y, and z are all whole numbers. Then, we can write the equation as follows: x + (y + z) = (x + y) + z
And, x · (y · z) = (x · y) · z
Distributive Property
The multiplication by the distributive property is expressed as follows when x, y, and z are all three whole digits: x (y + z) = (x y) + (x z).
In a similar way, the formula for the distribution property known as multiplication over subtraction is as follows: X x (Y – Z) = (X x Y) – (X x Z)
Can Whole Number Be Negative
It is impossible for the whole number to be negative! The justification for this stance can be seen down below:
- According to the concept of whole numbers, the number line is constituted in the whole numbers line as indicated by “0, 1, 2, 3, 4, 5, 6… till positive infinity.”
- There is no room for negative numbers in the whole numbers line.
Important Facts
- There is no such thing as the “largest” whole number.
- Every whole number, with the exception of 0, has an immediate predecessor.
- A number that is either decimal or fractional in nature and that lies between two whole numbers cannot be considered a whole number.
Examples
Example 1
Using distributive property, find the result of the following operation on whole numbers: 5 × (2 + 8). Is the result also a whole number? Will that always be the case?
Solution
The distributive property, in this case, is as follows:
x × (y + z) = (x × y) + (x × z)
5 × (2 + 8) = (5 × 2) + (5 × 8)
= 10 + 40
= 50
Therefore, 5 multiplied by (2 +10) equals 50, which is also a whole number. No, the result of operations on whole numbers is not necessarily a whole number if the operation is that of division.
Example 2
Take the whole numbers 5, 7, and 8 into consideration. Now Show associative property under the addition and multiplication operations.
Solution
5 + (7 + 8) = 5 + 15 = 20
(5 + 7) + 8 = 12 + 8 = 20
Thus:
5 + (7 + 8) = (5 + 7) + 8
5 × (7 × 8) = 5 × 56 = 280
(5× 7) × 8 = 35 × 8 = 280
Here:
5 × (7 × 8) = (5× 7) × 8
As a result, addition and multiplication are operations that can be performed associatively on whole integers.
All images were created with GeoGebra.