JUMP TO TOPIC
Width|Definition & Meaning
Definition
The width of a shape or object is its lateral or side-to-side distance. Since it represents length, it can be measured in any of the units of length, such as m, cm, inches, feet, etc. It can also represent the spacing between two parallel lines and other things.
One way to think about width is as the horizontal measurement, but it may also be thought of as the distance calculated from side to side. How does one determine how wide something is? It is measured perpendicular to the length of the object. The distance between two adjacent sides of an object is its width.
One way to determine the width of a road, for instance, is to measure the distance that extends through one side of a road towards the other.

Figure 1 – Length and width of a rectangle.
In geometry, the term “width” most frequently refers to the dimension that measures the length of the object’s shorter side. For instance, in the provided rectangles, both represent the same rectangle, although one of them has been rotated slightly so that it can be seen that the width refers to the side of a rectangle that is the shortest.
What Are Length, Width, and Height?
The length, width, and height of a geometrical shape are its dimensions, and they represent the length, width, and height of the form, respectively. The length of a figure is the side that is the longest, while the width is the side that is the shortest and the height is the dimension that is vertical. To determine the dimensions of an object, the tools that are needed are the object’s length, width, and height.
We just use length and width to describe two-dimensional shapes (also known as 2D shapes), but when we talk about three-dimensional shapes (also known as 3D shapes), we also talk about the height in addition to the length and width.
Let’s take a moment to define these three concepts.
Length is a measurement that determines the distance that exists between two places.
The length of an object or figure is represented by the length dimension of a figure, which is the longest dimension of a figure. Linear units such as meters, centimeters, and inches, among others, are used to express it.
Width is indeed the shorter distance between two points on an object or figure; it indicates how broad or even wide the provided figure is. Width is measured in centimeters.
There are additional linear units that are used to express width, such as meters, centimeters, inches, etc. The height of an object is its depth, or the third vertical dimension of the object, and it indicates how high or deep an object is. The height of an object can also be referred to as its depth.
Linear measurements such as meters, centimeters, and inches are used to indicate the height and depth of an object. Other linear units include millimeters.
Note that the words length, width, height, and depth are all obtained from the words long, wide, high, & deep, respectively. Length, breadth, height, and depth all refer to dimensions. Because of this, they communicate the proportions of an object. Take a look at the diagram that is provided below to get an idea of the length, breadth, & height of such a cuboid.
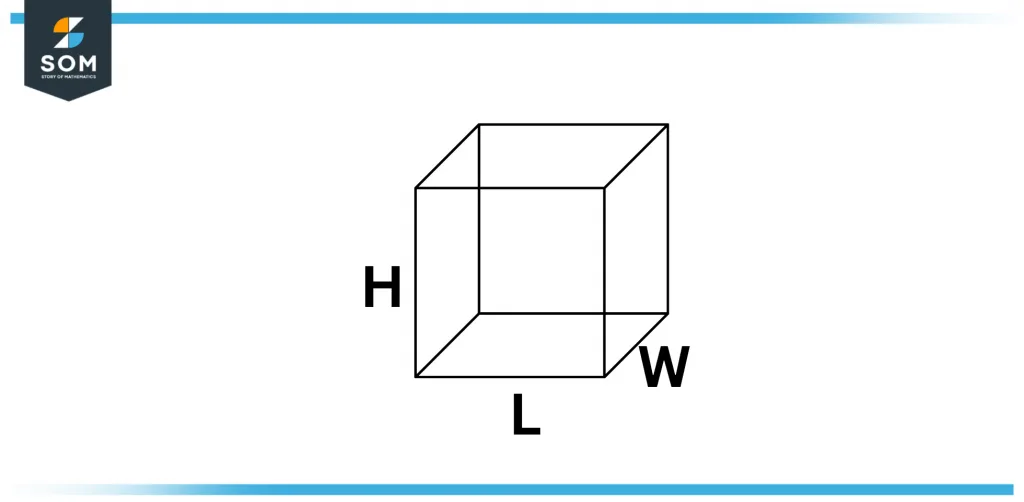
Figure 2 – Cuboid
Width vs. Length
The length and breadth of a figure differ in that length represents the longer side & width represents the shorter side. The length indicates exactly long the figure is, while the width indicates how broad or wide it really is. The width also is known as the breadth.
For instance, if indeed the two sides of a rectangle are 8 cm and 3 cm, we can readily determine that the rectangle’s length is 8 cm and its width is 3 cm.
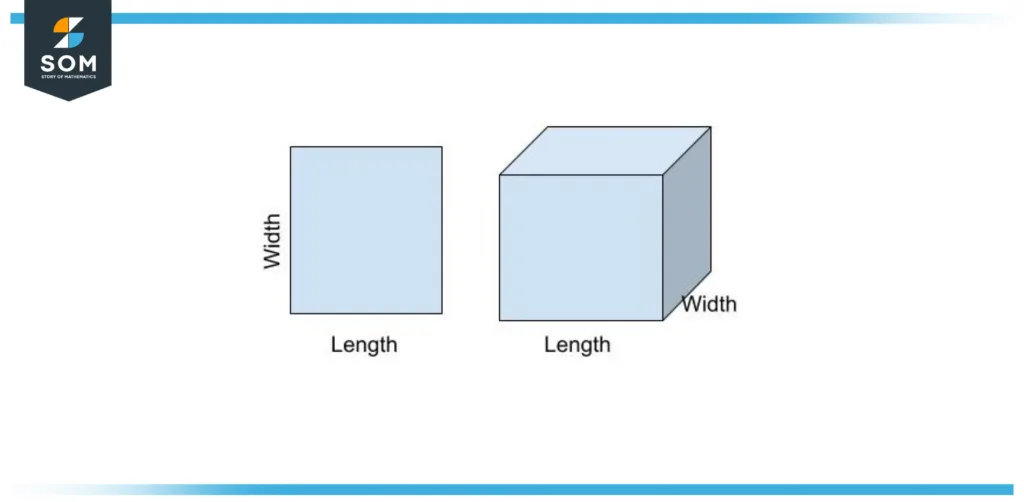
Figure 3 – Length and width of square and cube
The term “width” in mathematics refers to the measurement of the separation between two parallel lines as well as surfaces. It is a basic idea in many disciplines, including mathematics, calculus, physical science, engineering, and biology, to name a few.
In geometry, the term “width” refers to the separation of two parallel sides of a two-dimensional object, like a circle, rectangle, or square. The width of a three-dimensional form, such as a cube, sphere, or cone, is the separation between two parallel faces.
In calculus, breadth is crucial to the understanding of integrals. A definite integral is a kind of integration that calculates the volume of such a three-dimensional space or the area under a curve.
The width of a region can be thought of as a variable in integrals, and the integral is used to determine the total area or volume of the region by adding the products of the region’s width and height at different places.
Additionally, width is important in optimization issues, especially in geometric optimization. The goal in these issues is to either decrease or enhance the breadth of a predetermined shape. For instance, width is used in architecture and engineering to choose the most effective design for a building or structure that needs to support a specific load.
Difference Between Length and Width
When separating length from breadth, there is presently some ambiguity.
The issue is that, based on where you learned it, the two have slightly different descriptions. When learning mathematics, most students are taught that the longest side of a rectangle parallelogram (which has parallel sides) will be the length, and the shortest side will be the width.
This holds true whether the longer side is on the horizontal or vertical axis. However, many people have noticed that the width is typically the dimension parallel to the horizontal plane, and the length is typically the vertical.
Confusion persists because an object’s length isn’t always its longest measurement in certain other respects.
For instance, some cables are wider than they are when sliced into shorter lengths. Additionally, the channel widths of FET transistors are larger in comparison to their channel lengths. However, to the layperson, length merely refers to how long something is, and width refers to how wide a given object is.
The breadth is another name for width. When the lengths of an object or shape form a right angle with the sides, like in the case of a rectangle, it is said to be across that object or shape. This is in contrast with the measurement of the area of the rectangle, which is a product of two units, the length and width, length and width are two basic one-dimensional units.
Today, a wide variety of length units are in use. The meter is the most fundamental unit of length measurement in the SI system. Length can also be measured in inches, feet, yards, and miles using Imperial or English units. There are a few more length units that are not SI.
Numerical Examples of Width
Example 1
If a cuboid is 8 units long, 4 units wide, and 3 units tall, calculate its volume.
Solution
Given that:
Length = 8 units
Width = 4 units
Height = 3 units
We have to find the volume of the cuboid for the given data.
We know that:
Volume of cuboid = L x W x H
By putting values, we get:
Volume of cuboid = 8 x 4 x 3
By simplifying, we get the following:
The volume of the cuboid = 96 units cube.
Example 2
If a cuboid is 8 units long, 5 units wide, and 3 units tall, calculate its volume.
Solution
Given that:
Length = 8 units
Width = 5 units
Height = 3 units
We have to find the volume of the cuboid for the given data.
We know that:
Volume of cuboid = L x W x H
By putting values, we get:
Volume of cuboid = 8 x 5 x 3
By simplifying, we get the following:
The volume of cuboid = 120 units cube.
All images were created with GeoGebra.
